1
$\begingroup$

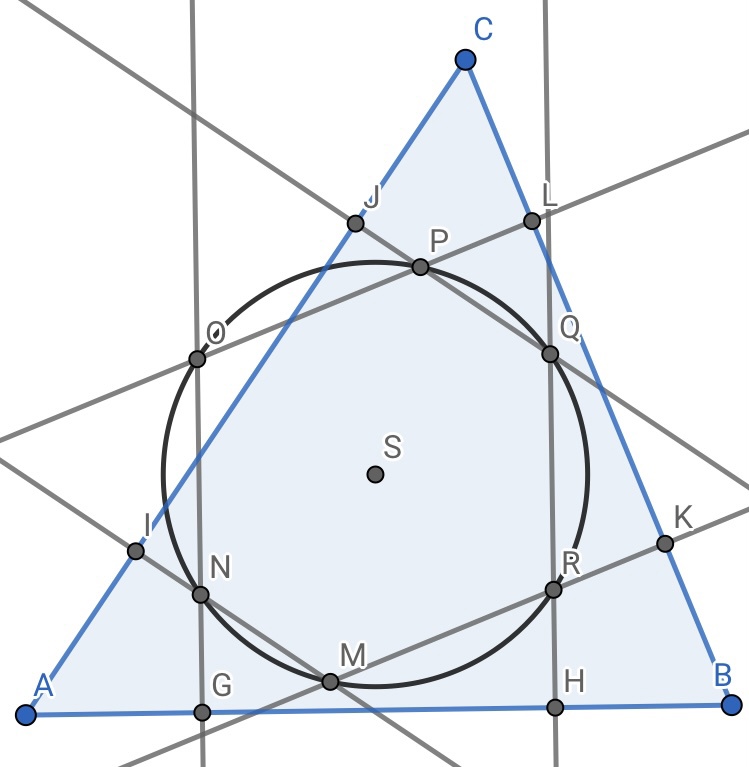
enter image description here

If six points are chosen, two points on each side of a triangle, such that they have the same ratio of distances to vertices, then the perpendicular lines through those points meet at six concyclic points. Has this been shown in the literature? If so, what is the formal name of this circle?

Improve this question
$\endgroup$
5
  • 1
    $\begingroup$ This is easy to prove when you notice the rectangles that the figure gives (using Thales theorem for example). The circumcircle of $ABC$ and this circle are concentric. For $PL=RK, RH=NG, KM=LO$, etc $\endgroup$
    – Toni Mhax
    Commented Sep 3 at 6:09
  • $\begingroup$ In general the diagonals of the hexagon do not pass through the center of the circle, so I don't think either of the claims in the previous comment is correct. The circle is a Tucker circle of the triangle formed by either triple of corresponding perpendiculars. If you'd like I can write up a short proof as a proper answer. $\endgroup$
    – N M
    Commented Sep 4 at 4:07
  • $\begingroup$ While it is true that the diagonals of the hexagon need not be concurrent, it is in fact true that the centre of the new circle coincides with the circumcentre. $\endgroup$
    – terceira
    Commented Sep 4 at 5:16
  • $\begingroup$ @NM, maybe you have another general form for the circle, but in the case i understood in the figure $OPRM$, $QRNO$, $PQMN$ are rectangles, as in the comment, this can be seen by Thales theorem (in basic triangles). I took $LC=KB=rCB$ and $CJ=AI=rAC$ and $AG=HB=rAB$ for the same ratio $r$... $\endgroup$
    – Toni Mhax
    Commented Sep 4 at 5:56
  • $\begingroup$ You're right, I was looking at a more general situation. I interpreted the question to mean that CJ:JI:IA = AG:GH:HB = BK:KL:LC, but not CJ=IA etc. With this setup the six points are still concyclic, but the center is not necessarily the circumcenter. $\endgroup$
    – N M
    Commented Sep 4 at 6:29

0

Reset to default

You must log in to answer this question.

Browse other questions tagged .