Na, Nb, Nc are the outer Nagel points. A'B'C' is the contact triangle. I claim that lines A'B', A'C', B'C' always cut the sides of the triangle NaNbNc at six points corresponding to an unknown circle. Surprisingly its center is not defined in the ETC:
Is it safe to say that this is actually the new central circle associated with the triangle ABC?
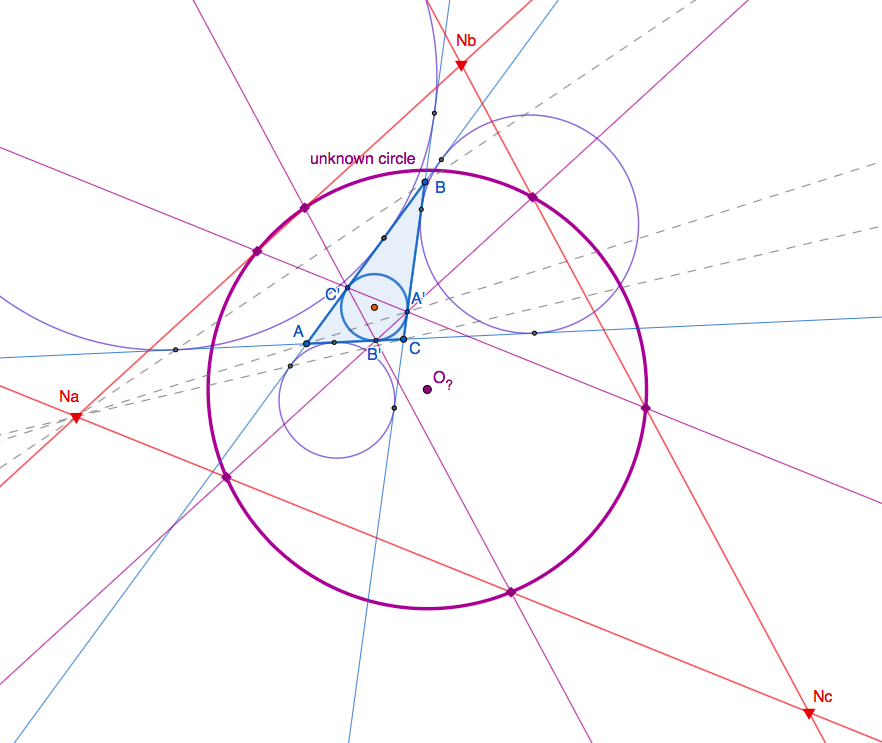
The above post comes with the only question ``Is it safe to say that this is actually the new central circle [...]'', and it is hard to put the hands on the two central objects in it -- that this and that the -- from the given data, so after complementing this post with the one on MSE 4381650 i suppose the issue is to show that the six points mentioned in the post are indeed on a circle, to determine the points coordinates, and the circle equation, so that the central circle definition can be tested. I will provide the equation in barycentric coordinates for the circle, its center and its radius, details for all steps are shown..
We have to fix for later use a clear notation for these six points, so let us state explicitly:
Proposition: Let $\Delta ABC$ be a given triangle with side lengths $a,b,c$. Let $A_1, B_1,C_1$ be the points of contact of the incircle with the sides. The $A$-excircle touches $BC$ in $A_2$, $AB$ in $A_{2B}$, and , $AC$ in $A_{2C}$. Consider also the points $B_2$, $B_{2A}$, $B_{2C}$; $C_2$, $C_{2A}$, $C_{2B}$, which are defined in a similar manner. Define the outer Nagel point $N_a$ as the intersection $N_a=AA_1\cap BC_{2A}\cap CB_{2A}$, and in a similar manner then $N_b,N_c$.
Consider now the line $N_aN_b$, and intersect it with the two lines through $C_1$, further passing through $A_1$, and respectively $B_1$, Denote the two intersections by $M_{ca}$ and $M_{cb}$. Define in a similar manner the other four points.
Then the six $M$-points with indices $ab,ac;ba,bc;ca,cb$ are on a circle whose (homogeneous) barycentric equation is: $$ \begin{aligned} 0 &= -a^2yz-b^2zx-c^2xy +(x+y+z)(ux+vy+wz)\ ,\qquad\text{ where}\\ 4u &= (b+c-2a)^2 - 5a^2\ ,\\ 4v &= (c+a-2b)^2 - 5b^2\ ,\\ 4w &= (a+b-2c)^2 - 5c^2\ . \end{aligned} $$ Let $\Delta$ be the area of $\Delta ABC$.
Proof: We denote by $s=\frac 12(a+b+c)$ the semiperimeter of the given triangle. For a point $P$ we write $P=(x,y,z)$ in case the $(x,y,z)$ are normed barycentric coordinates, i.e. $x+y+z=1$ and $P=xA+yB+zC$. The notation $P=[x:y:z]$ with $x+y+z\ne 0$ is used to denote the point with normed barycentric coordinates $x/(x+y+z)$, $y/(x+y+z)$, and $z/(x+y+z)$.
Then computations involving linear equations, or equations of degree two with one known solution lead to the following formulas for the points involved in the Proposition: $$ \begin{aligned} A &= (1,0,0)\ ,\\ B &= (0,1,0)\ ,\\ C &= (0,0,1)\ ,\\[2mm] A_1 &= [0 \; :\; s-c\; :\; s-b]\ ,\\ B_1 &= [s-c\; :\; 0 \; :\; s-a]\ ,\\ C_1 &= [s-b\; :\; s-a\; :\; 0]\ ,\\[2mm] A_2 &= [0 \; :\; s-b\; :\; s-c]\ ,\\ B_2 &= [s-a\; :\; 0\; :\; s-c]\ ,\\ C_2 &= [s-a\; :\; s-b\; :\; 0]\ ,\\[2mm] A_{2B} &= [-(s-c)\; :\; s \; :\; 0]\ ,\\ A_{2C} &= [-(s-b)\; :\; 0 \; :\; s]\ ,\\ B_{2A} &= [s \; :\; -(s-c)\; :\; 0]\ ,\\ B_{2C} &= [0 \; :\; -(s-a)\; :\; s]\ ,\\ C_{2A} &= [s \; :\; 0 \; :\; -(s-b)]\ ,\\ C_{2B} &= [0 \; :\; s \; :\; -(s-a)]\ ,\\[2mm] N_a &=[ -s\; :\; s-c\; :\; s-b]\\ N_b &=[ s-c\; :\; -s\; :\; s-a]\\ N_c &=[ s-b\; :\; s-a\; :\; -s]\\[2mm] M_{ab} &= \left(\ -\frac12\cdot\frac{b^2+c^2-2bc + ca+ab}{a^2-b^2+c^2} \ ,\ \frac 12\cdot\frac s{s-b} \ ,\ \frac 12\cdot\frac{-2b^2+c^2-bc+ca+2ab}{a^2-b^2+c^2} \ \right)\ ,\\ M_{ac} &= \left(\ -\frac12\cdot\frac{b^2 + c^2 - 2bc + ca + ab}{2c(s-c)} \ ,\ \frac 12\cdot\frac{b^2 - 2c^2 - bc + 2ca + 2ab}{2c(s-c)} \ ,\ \frac 12\cdot\frac s{s-c} \ \right)\ , \end{aligned} $$ and the next $M$-points are obtained by cyclic permutations.
Since the equation of the claimed circle is conserved by cyclic permutations, it is enough to (computer) check it is satisfied for the two points $M_{ab}$, $M_{ac}$, and this is indeed so.
$\square$
Usually, one introduces now $$ \begin{aligned} \alpha &= \cot A = \frac 1{4\Delta}(b^2+c^2-a^2)\ ,\\ \beta &= \cot B = \frac 1{4\Delta}(c^2+a^2-b^2)\ ,\\ \gamma &= \cot C = \frac 1{4\Delta}(a^2+b^2-c^2)\ \end{aligned} $$ and then the following relations are holdind true: $$ \begin{aligned} a^2 &= 2\Delta(\beta+\gamma)\ ,\\ b^2 &= 2\Delta(\gamma+\alpha)\ ,\\ c^2 &= 2\Delta(\alpha+\beta)\ . \end{aligned} $$ Then after setting $$ \begin{aligned} \lambda &= \frac u{2\Delta}\ ,\\ \mu &= \frac v{2\Delta}\ ,\\ \nu &= \frac w{2\Delta}\ , \end{aligned} $$ the above equation of the circle is: $$ \begin{aligned} 0 &= -\frac 1{2\Delta}(a^2yz+b^2zy+c^2xy)+\frac 1{2\Delta}(x+y+z)(ux+vy+wz)\ ,\qquad\text{ i.e.}\\[2mm] 0 &= -(\beta+\gamma)yz -(\gamma+\alpha)zx -(\alpha+\beta)xy + (x+y+z)(\lambda x+\mu y+\nu z)\ . \end{aligned} $$ Under such circumstances, $(\lambda, \mu,\nu)$ are called the barycentric coordinates of the involved circle, and they determine it. The center $\Omega$ of this circle has coordinates $(x_\Omega,y_\Omega,z_\Omega)$ that can be extracted from the formula $$ \begin{aligned} 2x_\Omega &= -(\beta+\gamma)\lambda +\gamma\mu +\beta\nu + (1-\beta\gamma) \\ &= -\frac{a^2}{2\Delta}\cdot \frac u{2\Delta} +\frac{a^2 +b^2 -c^2}{4\Delta}\cdot\frac v{2\Delta} +\frac{a^2 -b^2 +c^2}{4\Delta}\cdot\frac w{2\Delta} \\ &\qquad\qquad + \left(1 -\frac{a^2 +b^2 -c^2}{4\Delta}\cdot\frac{a^2 -b^2 +c^2}{4\Delta}\right) \\ &=\frac1{16\Delta^2} \Big[ 3a^3(b+c) - 3a(b^3+c^3) + a^2(b^2+c^2) -(b^2+c^2)^2 +3abc(b+c-2a)\Big] \ , \end{aligned} $$ and the ones given by cyclic permutations of $a,b,c$ for $y_\Omega,z_\Omega$.
For the special case of $a,b,c$ equal to $6,9,13$ we obtain $\displaystyle\Omega=\frac1{302}(20,101,181)$, and the value to be searched for in ETC Search_6_9_13 is
0.2798855012702361226525051823328973174886103479973667805028
and well, it is not in there.
