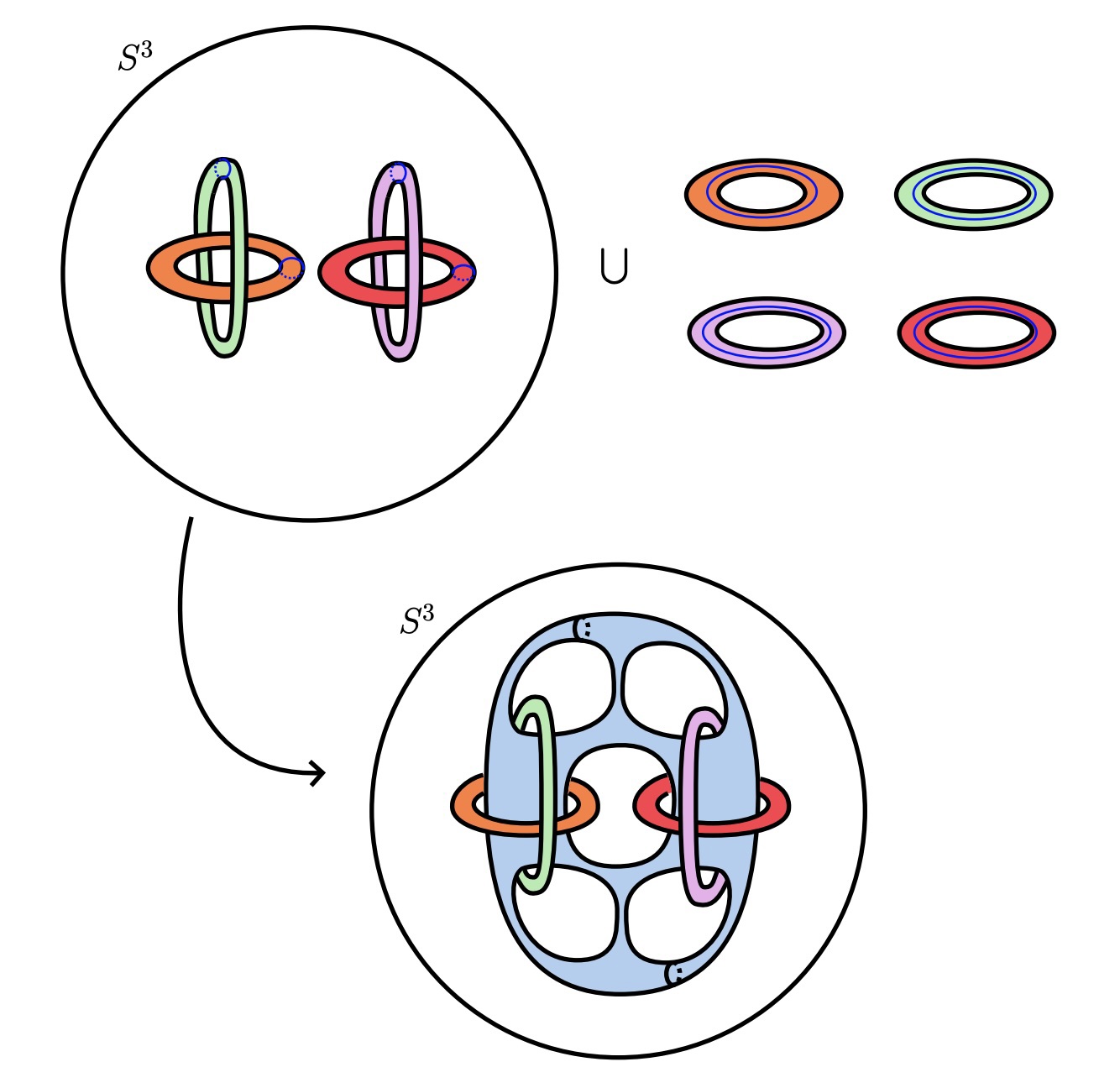
Consider the following three-dimensional topology. Start with $S^3$ and drill out four unlinked tori as shown in the picture. Then, fill in the gaps with the same tori but with their longitudes and meridians exchanged —that is, reattaching the tori after doing an S transform. To finish the construction, drill out a genus five handlebody as shown in the figure, so that the resulting manifold has a genus five surface as a boundary. Is it possible to endow this manifold with a hyperbolic metric?
1 Answer
This three-manifold can also be constructed by taking a genus two surface $S$, crossing with the interval $I$ to get $S \times I$, and attaching a pair of one-handles both of which connect $S \times \{0\}$ to $S \times \{1\}$. Applying the Klein combination theorem gives infinitely many complete hyperbolic metrics on the result.
To see this: Note that the boundary (blue) is compressible along two curves (the compressing disks meet the orange and the red, respectively). After compressing, the remains of the blue is a pair of genus two surfaces. Now find enough annuli (some will need to cross the green, or purple, or both) to obtain the product.