3
$\begingroup$

In Theorem 1.1 (i) of http://matwbn.icm.edu.pl/ksiazki/aa/aa24/aa2451.pdf, Iwaniec showed that a certain type of quadratic polynomial $P(x,y)$ represents infinitely many primes, where $(x,y) \in \mathbb{Z}^2$. 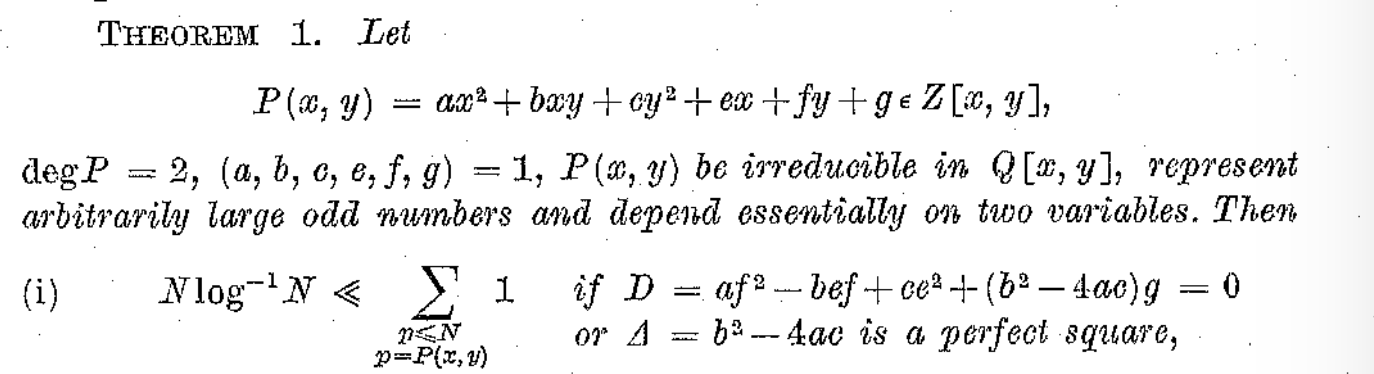enter image description here

Is there any simple argument showing that $P(x,y)$ also represents infinitely many primes, where $(x,y) \in \mathbb{Z}_+^2$?

Any suggestions would be greatly appreciated. Thank you in advance.

Improve this question
$\endgroup$
2
  • 2
    $\begingroup$ Please use a high-level tag like "nt.number-theory". I added this tag now. Regarding high-level tags, see meta.mathoverflow.net/q/1075 $\endgroup$
    – GH from MO
    Commented Jul 15 at 6:45
  • 1
    $\begingroup$ Do you mean "quadratic form" or "quadratic polynomial"? That an irreducible, primitive binary quadratic form represents infinitely many primes is well-known. The point of his paper is to treat arbitrary quadratic polynomials $f$ which are generically geometrically irreducible (unlike forms). $\endgroup$
    – Stanley Yao Xiao
    Commented Jul 15 at 22:06

0

Reset to default

You must log in to answer this question.