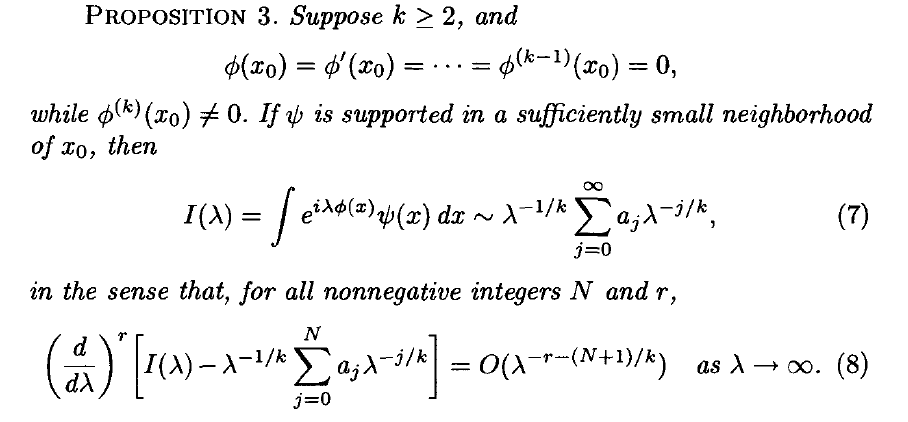The following is mostly adapted from [1] (I am the second co-author). Suppose
$$
\psi(x) \sim x^{-1+\beta/\rho} \sum_{j=0}^{\infty} \psi_j x^{j/\rho}, \quad
\phi(x) \sim x^{s/\rho} \sum_{j=0}^{\infty} \phi_j x^{j/\rho} $$
where $\beta, \rho, s > 0$, $\psi_0 \ne 0$, and $\phi_0 > 0$. To address the original question, we can presumably take $\beta = \rho = 1$ and $s=k$. Under the change of variables $\tau = [g(x)]^{1/s}$, the integral transforms to
$$\int \mathrm{e}^{\mathrm{i} \lambda \phi(x)} \psi(x) \; dx = \int \Psi(\tau) s \tau^{\beta-1} \mathrm{e}^{\mathrm{i} \lambda \tau^s} \; d\tau $$
where
$$ \Psi(\tau) = \tau^{s-\beta} \frac{\psi(\phi^{-1}(\tau^s))}{\phi'(\phi^{-1}(\tau^s))} \sim \sum_{j=0}^\infty \Psi_j \tau^j, $$
for some coefficients $\Psi_j$ given explicitly below.
An asymptotic expansion for the latter integral is readily established by repeatedly applying integration by parts. The contribution of the critical point to the integral (so an asymptotic expansion of the integral, e.g., if we assume $\psi$ has compact support and the only critical point is $x=0$) is
$$ -\sum_{n=0}^{\infty} \left(\frac{\mathrm{i}}{\lambda}\right)^{\sigma_n} \Gamma(\sigma_n) \Psi_n
= \lambda^{-\beta/s} \sum_{j=0}^\infty a_j \lambda^{-j/k}
$$
where we define $\sigma_n = (\beta+n)/s$ and where
$$ a_n = -\mathrm{i}^{\sigma_n} \Gamma(\sigma_n) \Psi_n . $$
The coefficients $\Psi_j$ are explicitly given by
$$ \Psi_n = \frac{\rho}{s} \phi_0^{-\sigma_n} \sum_{j=0}^n \psi_{n-j} \sum_{\pi \in \Pi(j)} \binom{-\sigma_n}{\pi} \prod_{i=1}^{j} \left(\frac{\phi_i}{\phi_0}\right)^{\pi_i}.$$
Here $\Pi(n) \subseteq \mathbb{N}_0^n$ is the set of multi-indices corresponding to partitions of the integer n,
$$ \pi \in \Pi(n) \quad\text{iff}\quad \pi_1 + 2 \pi_2 + 3\pi_3 + \dotsb + n \pi_n = n, $$
and we use a nonstandard definition for the multinomial coefficient,
$$ \binom{z}{\pi} = \frac{z(z-1)\dotsm(z-|\pi|+1)}{\pi_1! \pi_2! \dotsm \pi_n!}, $$
where $|\pi| = \pi_1 + \pi_2 + \dotsb + \pi_n$.
See [1] for details of the derivation.
In particular the first few coefficients are
$$\begin{aligned}
a_0 &= -\frac{\rho}{s}\left(\frac{\mathrm{i}}{\phi_0}\right)^{\beta/s} \Gamma\left(\frac{\beta}{s}\right) \psi_0, \\
a_1 &= -\frac{\rho}{s}\left(\frac{\mathrm{i}}{\phi_0}\right)^{(\beta+1)/s} \Gamma\left(\frac{\beta+1}{s}\right) \left(\psi_1 - \frac{(\beta+1)\psi_0 \phi_1}{s \phi_0}\right), \\
a_2 &= -\frac{\rho}{s}\left(\frac{\mathrm{i}}{\phi_0}\right)^{(\beta+2)/s} \Gamma\left(\frac{\beta+2}{s}\right) \left[\psi_2 +\frac{\beta+2}{s}\left(- \frac{\psi_1 \phi_1}{\phi_0} + \frac{(1+\frac{\beta+2}{s})\psi_0 \phi_1^2}{2\phi_0^2}-\frac{\psi_0\phi_2}{\phi_0}\right)\right] . \\
\end{aligned}$$
[1] Lyness, James N.; Lottes, James W., Asymptotic expansions for oscillatory integrals using inverse functions, BIT 49, No. 2, 397-417 (2009). ZBL1173.41014.