Suppose you have a continuous function $f:[a,b]\times (-\infty, \infty) \rightarrow \mathbb{R}$. I'm trying to understand if it's possible to conclude that due to the compactness of the interval $[a,b]$ there is some $x_0$ such that $$f(x,y) \leq C f(x_0,y) \qquad \forall y\in (-\infty, \infty) \mbox{ with } C>0 \mbox{ constant.}$$
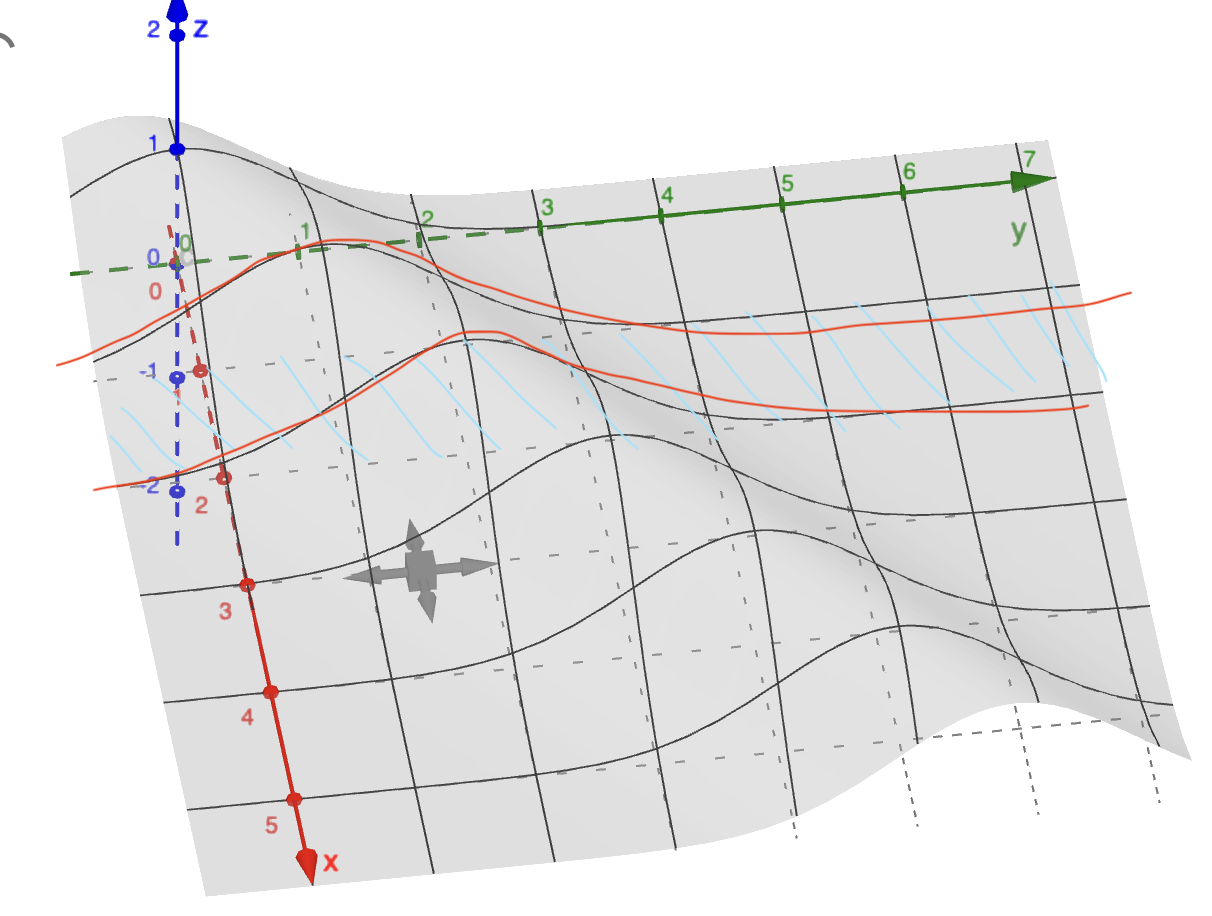
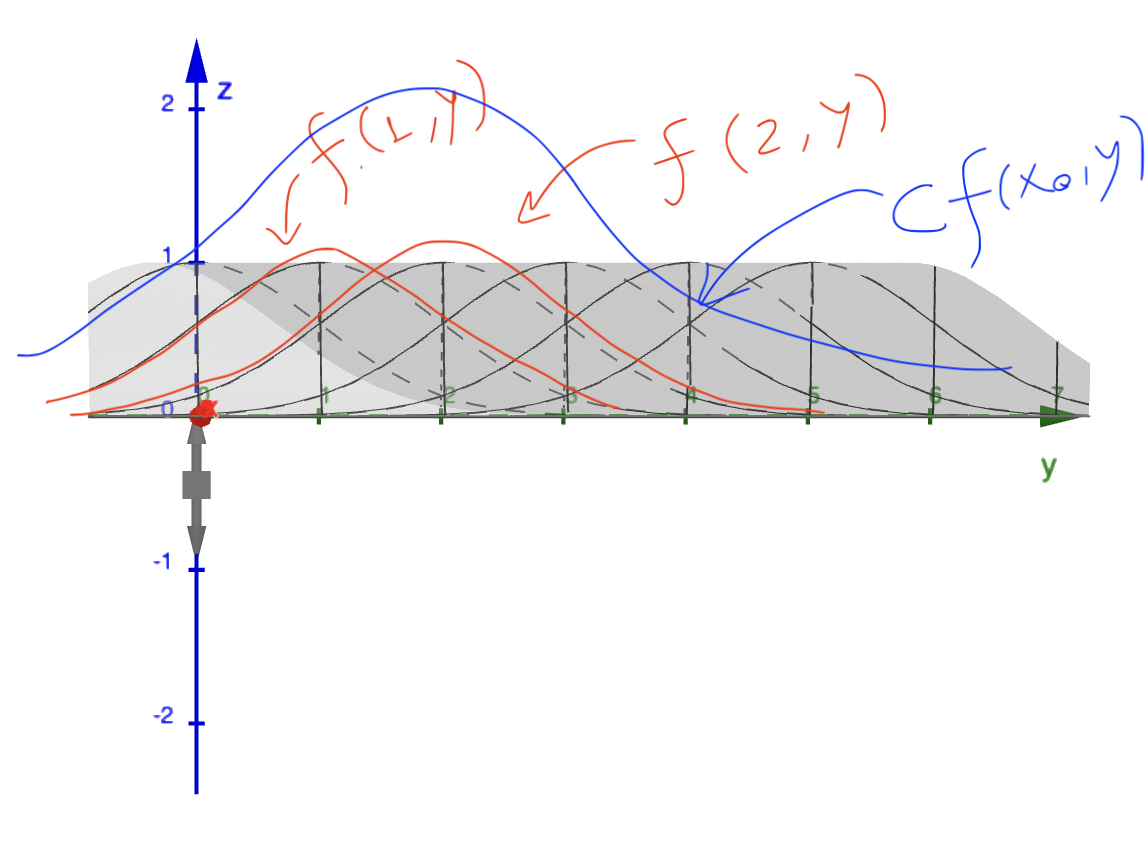
Although [a,b] is compact, my question is that you have to compare the curves $ f(\cdot,y)$ with $y\in (-\infty, \infty)$ for each $x\in [a,b]$. I don't know if it's always true, I can have some geometric intuition when $f(\cdot, y)$ is monotone. By example $𝑓(𝑥,𝑦)=𝑥^2+𝑦^2$ with $x\in [0,1]$ and $y\in (-\infty, \infty)$ for example $𝑓(𝑥,𝑦)\leq 1+𝑦^2=𝑓(1,𝑦)$. Observing a more complicated case $f(x,y)=ℯ^{-(x-y)^2}$ with $x \in [1,2]$ as in the figures below
I'm not sure if it's possible to get a curve like the blue one $C.f(x_0,y)$ in the figure, which dominates $f(x,y)$ with $x \in [1,2]$ and $y \in (-\infty, \infty)$ although the curves $f(\cdot, y)$ are just translations of each other. Are there any analysis results related to this? Even under more restricted assumptions? Thanks in advance!