Dividing a spherical cap into $n$ equal wedges

Question

This is a follow-up of the question Dividing a spherical cap into three equal wedges where the $n=3$ case was shown.

Motivation: Optimal ways to cut an orange.

In this problem, we have a spherical orange of radius $R$, and we do not wish to eat its central column which is modelled as a cylinder of radius $r>0$. Part of the procedure involves an initial cut down the orange tangential to the central column, which produces a spherical cap, then further splitting the cap into $n$ wedges where each cut passes through the midpoint of the initial cut. The goal is to make these $n$ wedges have equal volumes.

The question is whether each of these $n$ wedges can have the same angle. A negative answer has been shown for the $n=3$ case, by deriving closed-form expressions for each volume and proving that they are incompatible with $\alpha_1=\alpha_2=\alpha_3=\pi/3$ and $V_1+V_2+V_3=\pi(R-r)^2(2R+r)/3$, which is the volume of the whole spherical cap.

Can this result be extended to $n>3$ wedges of identical volume with angle $\pi/n$?

Answer 1

The answer is no for $0<r<R$. It is impossible to divide a skewed spherical cap into $n$ wedges with equal angles. The proof goes as follows.

proof sketch:

We show that at each cross section of the sphere consisting sections of the wedges, it is the area of the cross sections of one wedge, always falls below the area of the cross section of another edge, regardless of which cross section of the spherical cap we choose. More formally, consider the following figure:

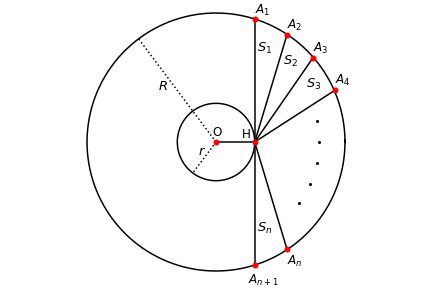
Assume that we have cut a cross section of radius $R$, where the smaller cylindrical stem has radius $r$. Then, if $\measuredangle A_1HA_2=\measuredangle A_2HA_3=\cdots=\measuredangle A_nHA_{n+1}=\frac{\pi}{n}$, we have $$ S_1>S_2>\cdots >S_{\left\lfloor\frac{n}{2}\right\rfloor}. $$ Also, note that due to symmetry, $S_i=S_{n+1-i}$.

To prove this, we need to find the areas $\{S_i\}_{i=1}^n$. Consider the following figure:

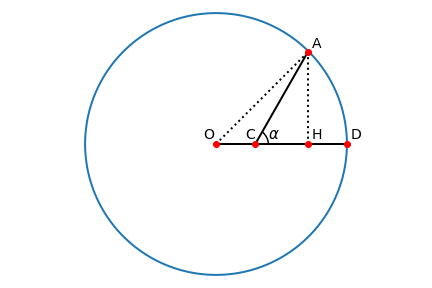
which illustrates a cross section of the sphere. Here we have $\text{OA}=R$ and $\text{OC}=r$. We want to find the area of the section AODA in terms of $\alpha$. By defining $\theta\triangleq\measuredangle\text{AOC}$, we have $$ \text{Area of AODA}=\frac{1}{2}(\theta R^2-Rr\sin\theta). $$ Note that the following relations exist in $\triangle\text{AOH}$: $$ {\text{OC}+\text{CH}=R\cos\theta \\ \text{AH}=R\sin\theta. } $$ Also, in $\triangle\text{ACH}$ we have $CH=AH\cot\alpha$. Therefore $$ CH=AH\cot\alpha=R\cos\theta-\text{OC}\implies{ R\sin\theta\cot\alpha=R\cos\theta-r \implies\\ R\sin\theta\cos\alpha=R\cos\theta\sin\alpha-r\sin\alpha \implies\\ R\sin(\alpha-\theta)=r\sin\alpha \implies\\ \sin(\alpha-\theta)=\frac{r}{R}\sin\alpha \implies\\ \alpha-\theta=2k\pi+\sin^{-1}\left(\frac{r}{R}\sin\alpha\right) \\\text{or}\\ \alpha-\theta=2k\pi+\pi-\sin^{-1}\left(\frac{r}{R}\sin\alpha\right), } $$ for $k\in\Bbb Z$. Since $\alpha\ge \theta$, the only acceptable solution is $\alpha-\theta=\sin^{-1}\left(\frac{r}{R}\sin\alpha\right)$, which leads to $$ \theta=\alpha-\sin^{-1}\left(\frac{r}{R}\sin\alpha\right). $$Let us denote the area of AODA by $I(\alpha;r,R)$. We are now able to express $\{S_i\}_{i=1}^n$ by $I(\alpha;r,R)$ as $$ S_i=I\left(\frac{\pi}{2}-(i-1)\frac{\pi}{n};r,R\right) - I\left(\frac{\pi}{2}-i\frac{\pi}{n};r,R\right)\quad,\quad 1\le i\le\frac{n}{2} $$ and $S_{i}=S_{n+1-i}$. To complete the proof of $S_1>S_2>\cdots >S_{\left\lfloor\frac{n}{2}\right\rfloor}$, we show that the function $I(\alpha;r,R)$ is convex for $\alpha\in(0,\frac{\pi}{2})$. Recall that $$ I(\alpha;r,R)=\frac{1}{2}(\theta R^2-Rr\sin\theta)\quad,\quad \theta=\alpha-\sin^{-1}\left(\frac{r}{R}\sin\alpha\right). $$ Note that $\theta$ is a strictly increasing convex function of $\alpha$ for $\alpha\in(0,\frac{\pi}{2})$ since $$ { \frac{d\theta}{d\alpha}=1+\frac{\frac{r}{R}\sin\alpha}{\left(1-\frac{r^2}{R^2}\sin^2\alpha\right)^\frac{1}{2}}>0, \\ \frac{d^2\theta}{d\alpha^2}=\frac{\frac{r}{R}\sin\alpha-\frac{r^3}{R^3}\sin^3\alpha}{\left(1-\frac{r^2}{R^2}\sin^2\alpha\right)^\frac{3}{2}}>0. } $$ Likewise, $f(\theta)=\frac{1}{2}(\theta R^2-Rr\sin\theta)$ is strictly increasing and convex for $\theta\in(0,\frac{\pi}{2})$. Since the combination of two strictly increasing and convex functions, is also strictly increasing and convex, we have proved that $I(\alpha;r,R)$ is strictly increasing and convex for $\alpha\in(0,\frac{\pi}{2})$.

Due to the convexity of $I(\alpha;r,R)$, we can conclude $$ I\left(\frac{\pi}{2}-i\frac{\pi}{n};r,R\right){= I\left(\frac{1}{2}\left[\frac{\pi}{2}-(i-1)\frac{\pi}{n}\right]+\frac{1}{2}\left[\frac{\pi}{2}-(i+1)\frac{\pi}{n}\right];r,R\right) \\< \frac{1}{2} I\left(\frac{\pi}{2}-(i-1)\frac{\pi}{n};r,R\right) + \frac{1}{2} I\left(\frac{\pi}{2}-(i+1)\frac{\pi}{n};r,R\right) \\\implies I\left(\frac{\pi}{2}-(i-1)\frac{\pi}{n};r,R\right)- I\left(\frac{\pi}{2}-i\frac{\pi}{n};r,R\right) \\> I\left(\frac{\pi}{2}-i\frac{\pi}{n};r,R\right)- I\left(\frac{\pi}{2}-(i+1)\frac{\pi}{n};r,R\right) \\\implies S_i>S_{i+1}\quad,\quad 1\le i\le \frac{n}{2}. } $$ Since this chain of inequality happens for any value of $R$ and $r$, provided that $0<r<R$, we have proved that a similar inequality exists among the 3-dimensional wedges and the proof is complete $\blacksquare$