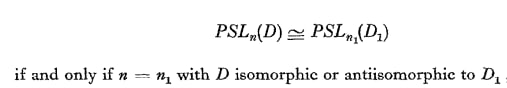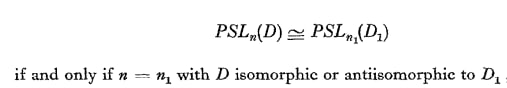
What's written above is true for all $n, n_1 \geq 2$ and all skew fields except two finite cases: $PSL(2, \Bbb F_7) \cong PSL(3, \Bbb F_2)$, $PSL(2, \Bbb F_4) \cong PSL(2, \Bbb F_5)$. (V. M. Petechuk, Isomorphisms between linear groups over division rings, Can. J. Math.Vol. 45 (5), 1993 pp. 997-1008). Case $n \geq 3$ is more or less folklore, with field case settled by Shreier-wan der Waerden, and skew field case by Dieudonne-Hua.
Addressing exact question on $PGL(n)$: I remember convincing myself a few years ago that isomorphism between $PGL(2)$-s over infinite skew fields should induce two-way inclusions between according $PSL(2)$-s, and a posteriori isomorphism, so claim should follow from $PSL$ reflecting isomorphisms; unfortunately, I have forgot exact arguments and have failed to reproduce them now. For $n \geq 3$ you can find exact proof in Hahn's paper referenced below, but dimension restriction is unavoidable if you follow his strategy.
Sligtly more general case where $D$ is merely a subring of division ring (but $n \geq 5$) can be found in O.T. O'Meara, A general isomorphism theory for linear groups, 1977. Possibilities of producing Morita equivalences from abstract group isomorphisms (for $n \geq 3$) is covered in A. J. Hahn, Category equivalences and linear groups over rings. Journal of Algebra, 77(2), 505–543.
As far as I know, possibility of abstract isomorphism between different linear groups over non-isomorphic skew fields is still an open problem.
An outdated, but pretty comprehensive survey on related topics can be found here https://web.osu.cz/~Zusmanovich/links/files/weisfeiler/1981-commalg.pdf