3
$\begingroup$

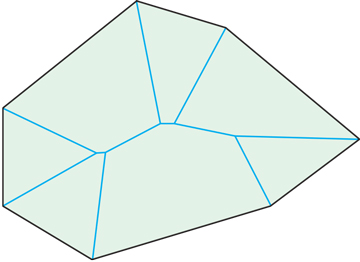
Let $P$ be a convex polygon.

Q1. What is the shortest collection of line segments $S$ inside $P$ with the property that both $P$ and $S$ have the same sequence of orthogonal shadows as $P$ and $S$ rotate?

An orthogonal shadow is cast by parallel light rays. For example, for $P$ a unit-square, the X below has length $2 \sqrt{2}$, less than the perimeter $4$ of $P$, but casts the same shadows: every lightray that intersects $\partial P$ intersects $S$; every lightray that intersects $S$ intersects $\partial P$:

     Square

Q2. Is $S$ always connected? Clearly it must span the vertices of $P$.

Q3. Is $S$ the medial axis of $P$?

     Polygon

     Medial axis. Fig.5.1(b) from Discrete and Computational Geometry.

I feel I should know or be able to find the answers to these questions, but I am not hitting on the appropriate search terms.

My real interest is in $\mathbb{R}^3$, but first let's explore $\mathbb{R}^2$.

Answered by Gerry Myerson:

  • Q1: Open problem
  • Q2: Not necessarily connected
  • Q3: No
Improve this question
$\endgroup$
5
  • 3
    $\begingroup$ The appropriate search term is opaque square. See facstaff.susqu.edu/brakke/opaque/opaqsq.html for what may be the solution. $\endgroup$
    – Gerry Myerson
    Commented Jun 26, 2022 at 12:07
  • $\begingroup$ @GerryMyerson: Thanks, that answers all three questions! $\endgroup$
    – Joseph O'Rourke
    Commented Jun 26, 2022 at 12:08
  • 1
    $\begingroup$ The proposal pointed to by @GerryMyerson has a length of $\sqrt{2}+\sqrt{3/2}$, indeed less than $2\sqrt{2}$: wolframalpha.com/input/… $\endgroup$
    – user44143
    Commented Jun 26, 2022 at 12:24
  • 1
    $\begingroup$ For a lower bound on the length $L$ of an opaque square, I can only prove that $L \ge 2$: Consider an approximation by curves, and let $p(t)\, dt$ be the fraction of those curves whose angle with the horizontal is between $t$ and $t+dt$. Considering shadows from a light at angle $u$ with the horizontal gives the inequality $$L \int_0^{\pi}|\sin(t-u)|\, p(t)\,dt \ge |\sin u|+|\cos u|$$ and averaging those inequalities over all possible $u$ gives $L\ge2$. Can anyone get a higher lower bound? $\endgroup$
    – user44143
    Commented Jun 26, 2022 at 19:01
  • $\begingroup$ @MattF.: Interesting question (perhaps worth posting separately?) Quite a gap between $\approx 2.64$ and your lower bound on $L$. $\endgroup$
    – Joseph O'Rourke
    Commented Jun 26, 2022 at 22:08

1 Answer 1

Reset to default
2
$\begingroup$

The current best lower bound on the total length of segments in $S$ is 2.00002:

A. Kawamura, S. Moriyama, Y. Otachi and J. Pach, A lower bound on opaque sets, Comput. Geom. 80 (2019), 13–22. https://doi.org/10.1016/j.comgeo.2019.01.002

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .