The twin prime constant is defined as
$$
\Pi_2 = \prod_{\substack{p\in\mathcal{P}\\p>2}}\frac{p-2}{p-1}\left(1-\frac{1}{p}\right)^{-1},
$$
where $\mathcal{P}$ is the set of primes. I'm interested in the finite setting, where $P_k$ is the product of the $k$ first primes. The corresponding constant is then
$$
\Pi_2(k) = \prod_{\substack{p>2\\p\mid P_k}}\frac{p-2}{p-1}\left(1-\frac{1}{p}\right)^{-1} $$
My question concerns the related sum
$$
\frac{1}{m}\sum_{j=1}^{m} \prod_{\substack{p\mid j \\ p\mid P_k\\p>2}}\frac{p-1}{p-2}.
$$
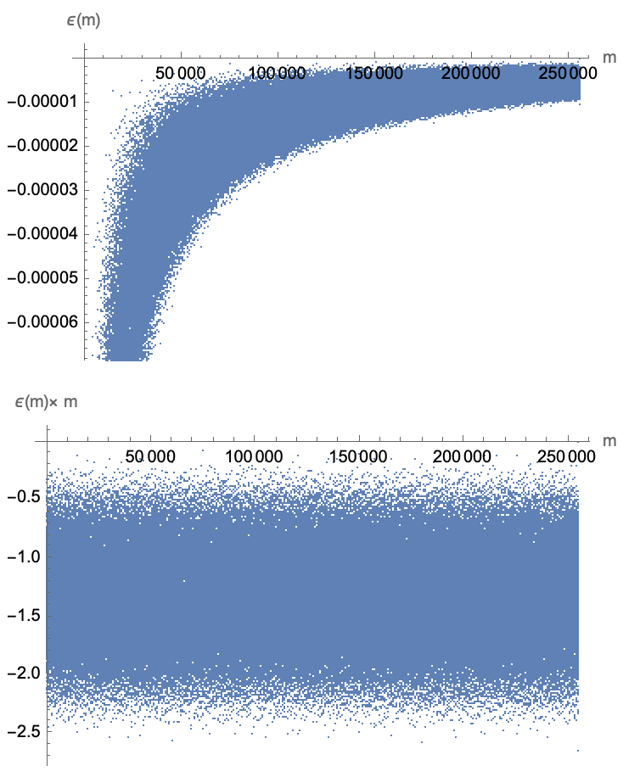
When $m=P_k$ this sum is exactly equal to $\Pi_2(k)^{-1}$, but I would like to know how fast this sum converges.
Question Is it possible to prove an estimate for the error $$ \epsilon_k(m)= \frac{1}{m}\sum_{j=1}^{m} \prod_{\substack{p\mid j \\ p\mid P_k\\p>2}}\frac{p-1}{p-2} - \Pi_2(k)^{-1}? $$ The numerics below (for $k=7$) suggest that $\epsilon_k(m)=O(1/m)$, but I haven't been able to show this theoretically.