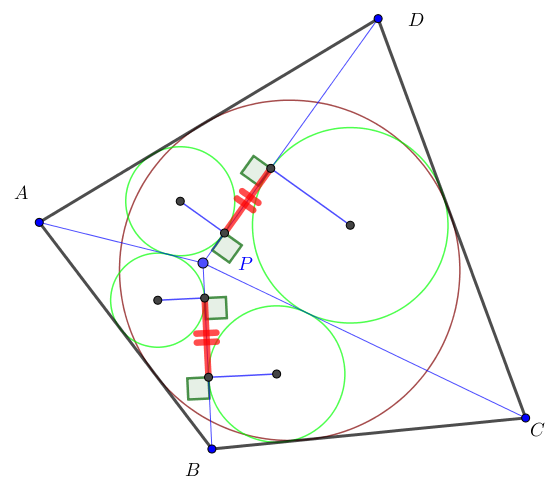
Let $ABCD$ be a quadrilateral, $P$ be a point in the plane let $E$, $F$ be the projections of the incenters of triangles $\triangle CPB$, $\triangle BPA$ onto $PB$ respectively; Let $G$, $H$ be the projections of the incenters of triangles $\triangle APD$, $\triangle DPC$ onto $PD$ respectively. Then $ABCD$ is a tangential quadrilateral if only if with every arbitrary point P in the plane $EF=GH$.
Following picture: $ABCD$ be a tangential quadrilateral if only if with $P$ be every arbitrary point in the plane then two red segment lengths are equal