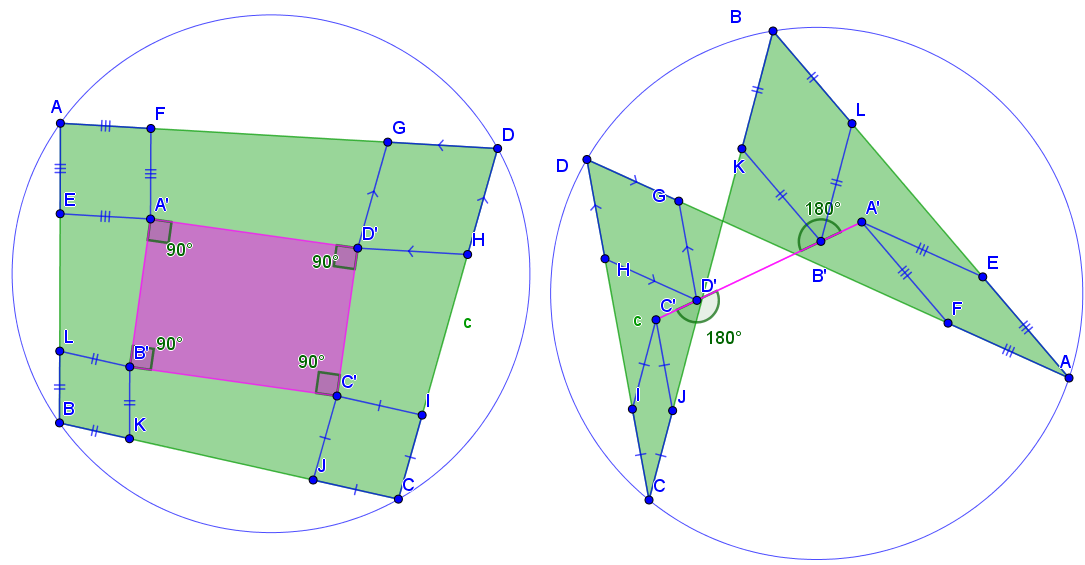
I am looking for a proof of a like result as follows and Higher-dimensional generalizations?
Let $A, B, C, D$ be four point with lengths of $AB, BC, CD, DA$ are $a, b, c, d$ respectively. Let $F \in DA, E \in AB$ such that $AE$ $=AF$ $=\frac{da}{a+b+c+d}$ construct point $A'$ such that $AEA'F$ is a rhombus (See Figure). Construct $B', C', D'$ cyclically, then:
1) Four points $A', B', C', D'$ form a parallelogram (like Varignon's theorem)
2) Four points $A', B', C', D'$ form a rectangle if $ABCD$ is convex cyclic quadrilateral (Like Japanese theorem for cyclic quadrilaterals.
Why I call this result is Brother of Japanese theorem for cyclic quadrilaterals?
Let $ABCD$ is convex cyclic quadrilateral, let $F \in DA, E \in AB$ such that $AE$ $=AF$ $=\frac{da}{a+d+BD}$ construct point $A'$ such that $AEA'F$ is a rhombus then $A'$ is the incenter of $\triangle ABD$. Construct $B', C', D'$ cyclically, then $A', B', C', D'$ form a rectangle. This is Japanese theorem for cyclic quadrilaterals
3) Four points $A', B', C', D'$ are collinear with $A'C'$, $B'D'$ have the same midpoint if $ABCD$ is the concave cyclic quadrilaterals (Like Butterfly theorem)
See also: