The following is obviously not optimal, but at least it shows that the optimal $c$ satisfies $c \leqslant 4$. On the other hand, $c \geqslant 2 / \log 2 \approx 2.885$, so if $c$ is to be integer, it remains to show that in fact $c < 4$ and hence necessarily $c = 3$.
Edit: As remarked in the comments, the following argument works for continuous decreasing functions, not necessarily convex.
Notation: Write $|A|$ for the area of $A$.
By approximation, it is sufficient to consider the case when $f'(0^+)$ and $f'(b^-)$ are finite and non-zero, and $f$ is strictly convex (edit: this is not really needed). Denote by $F$ the region under the graph of $f$:
$$ F = \{(x, y) \in [0, \infty)^2 : y \leqslant f(x)\} .$$
Rather than assuming that $|F| = 1$, we allow for arbitrary $|F|$ and study the inequality
$$ \inf \sum_{j = 1}^k |R_j| \leqslant c |F| , \tag{$\spadesuit$} $$
where the infimum is taken over all possible families of "proper" rectangles $R_j$ that cover $F$.
We construct rectangles $R_j = [0, a_j] \times [0, b_j]$ in an iterative way. By $D_j$ we denote the "decreasing" diagonal of $R_j$: the line segment connecting $(0, a_j)$ with $(b_j, 0)$.
First, we choose $b_1 = b$ and $a_1 = b / |f'(0^+)|$, so that the diagonal $D_1$ is tangent to the graph of $f$ at $(0, b)$.
Once $a_j$ and $b_j$ are chosen, we set $b_{j+1} = f(a_j)$ and we choose $a_{j+1}$ in such a way that the diagonal $D_{j+1}$ is tangent to the graph of $f$. More precisely, $a_{j+1}$ is the largest number such that $D_j$ is contained in $F$. If $a_{j+1} = a$, we set $k = j + 1$, and we stop.
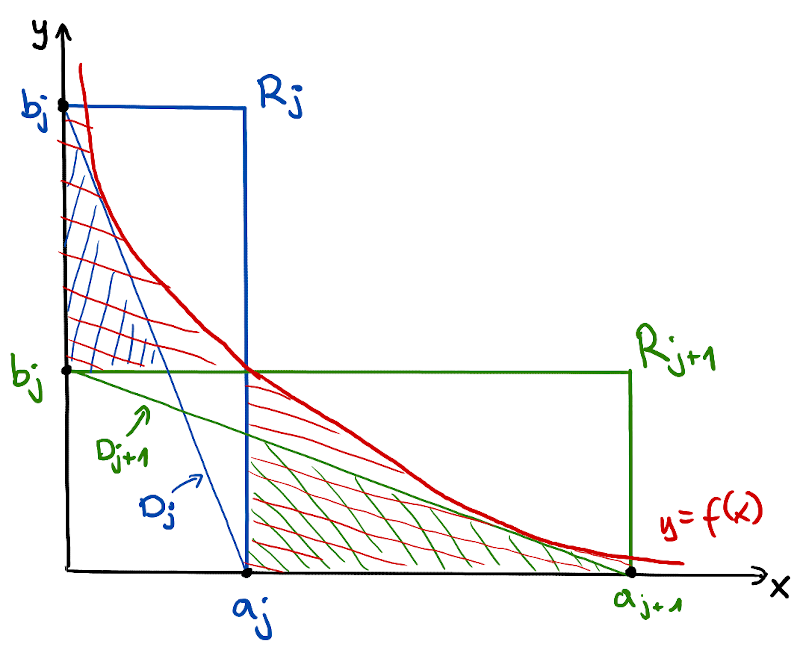
Step 1. Consider two adjacent rectangles $R_j = [0, a_j] \times [0, b_j]$ and $R_{j+1} = [0, a_{j+1}] \times [0, b_{j+1}]$ (see figure above). The diagonal $D_j$ is tangent to the graph of $f$ at a certain point $(\alpha, \beta)$. This point lies below the segment with endpoints $(0, b_j)$ and $(a_j, b_{j+1})$ (the "decreasing" diagonal of $[0, a_j] \times [b_j, b_{j+1}]$). Thus, this segment necessarily lies above the secant line of $f$ passing through $(\alpha, \beta)$ and $(a_j, b_{j+1})$, and this, in turn, lies above the tangent line to the graph of $f$ at $(a_j, b_{j+1})$. It follows that,
$$ \frac{b_j - b_{j+1}}{a_j} \geqslant |f'(a_j)| . $$
Similarly,
$$ |f'(a_j)| \geqslant \frac{b_{j+1}}{a_{j+1} - a_j} \, , $$
and hence
$$ \frac{b_j - b_{j+1}}{a_j} \times \frac{a_{j+1} - a_j}{b_{j+1}} \geqslant 1 . $$
We write $a_j = p a_{j+1}$ and $b_{j+1} = q a_{j+1}$. The above inequality reads
$$ \frac{1 - q}{p} \times \frac{1 - p}{q} \geqslant 1 . $$
Equivalently, $(1 - p) (1 - q) \geqslant p q$, or
$$ p + q \leqslant 1 . $$
Geometrically speaking: the "decreasing" diagonal of a larger rectangle $[0, a_{j+1}] \times [0, b_j]$ passes above the point $(a_j, b_j)$.
Step 2. The above implies that our procedure ends after a finite number of steps. Indeed:
$$ \frac{b_{j+1} / a_{j+1}}{b_j / a_j} = p q \leqslant \frac{1}{4} , $$
and so $b_j / a_j$ eventually becomes less than or equal to $|f'(a^-)|$. This, however, means that $b_j \leqslant a |f'(a^-)|$ and consequently $a_j = a$ and $k = j$. (This shows that in fact $k \leqslant \log_4 |f'(0^+) / f'(a^-)|$.)
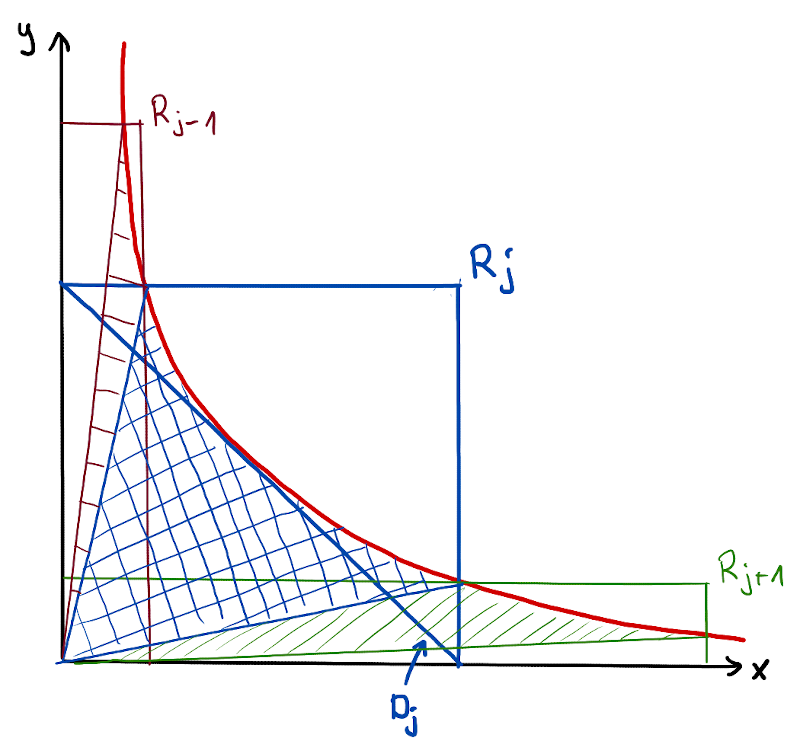
Step 3. We divide $F$ with line segments that connect $(a_j, b_{j+1})$ with the origin. Let $F_j$ be the curvilinear triangle with vertices $(0, 0)$, $(a_{j-1}, b_j)$ and $(a_j, b_{j+1})$ (the blue region in the figure above). We claim that
$$|F_j| \geqslant \tfrac{1}{4} (|R_j| + |R_{j+1}|) . \tag{$\clubsuit$}$$
In order to prove this claim, observe that $|F_j|$ is clearly greater than the area of the blue region $F_j'$ in the following picture.
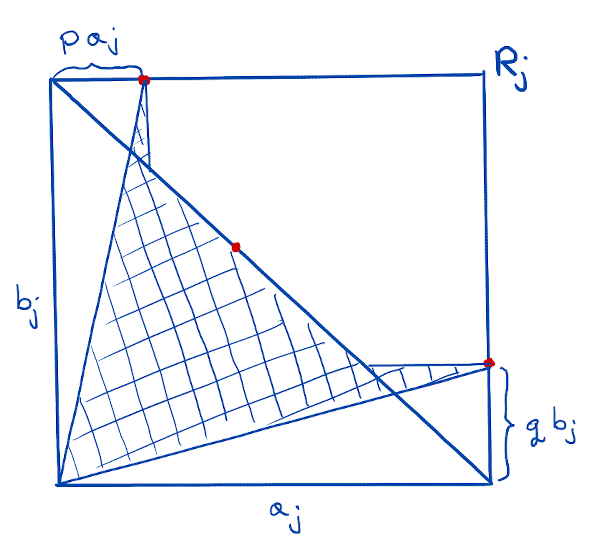
Denote $p = a_{j-1} / a_j$ and $q = b_{j+1} / b_j$, and let $(\alpha, \beta)$ be the point at which the diagonal $D_j$ is tangent to the graph of $f$. Since $p a_j = a_{j-1} \leqslant \alpha$ and $q b_j = b_{j+1} \leqslant \beta$, and $\tfrac{\alpha}{a_j} + \tfrac{\beta}{b_j} = 1$ (the point $(\alpha, \beta)$ lies on the diagonal $D_j$), we have $p + q \leqslant 1$. The area of the blue region on the last picture is equal to
$$ \tfrac{1}{2} (1 - p - q + p^2 + q^2) a_j b_j , $$
and this is minimised at $p = q = \tfrac12$. Thus,
$$ |F_j| \geqslant |F_j'| \geqslant \tfrac{1}{4} a_j b_j = \tfrac{1}{4} |R_j| , $$
as claimed. Our claim is proved.
Step 4. By summing up over all $j$, we see that
$$ |F| \geqslant \sum_j |F_j| \geqslant \frac{1}{4} \sum_j |R_j| . $$
In other words, the inequality ($\spadesuit$) holds with $c = 4$.
The constant $c = 4$ in ($\spadesuit$) does not seem optimal, but it cannot be improved much. If $f(x) = \tfrac{1}{x}$ for $x \in [1, 2^n]$, and $f$ is otherwise linear, then the optimal choice seems to be $R_0 = [0,1]\times[0,2]$, $R_j = [0, 2^j] \times [0, 2^{1-j}]$ for $j = 1,2, \ldots, n$ and $R_{n+1} = [0, 2^{n+1}] \times [0, 2^{-n}]$. The area under $f$ is $3 + n \log 2$, while the sum of the areas of $R_j$ is $4 + 2 n$, and so $c \geqslant 2 / \log 2 \approx 2.885$.



