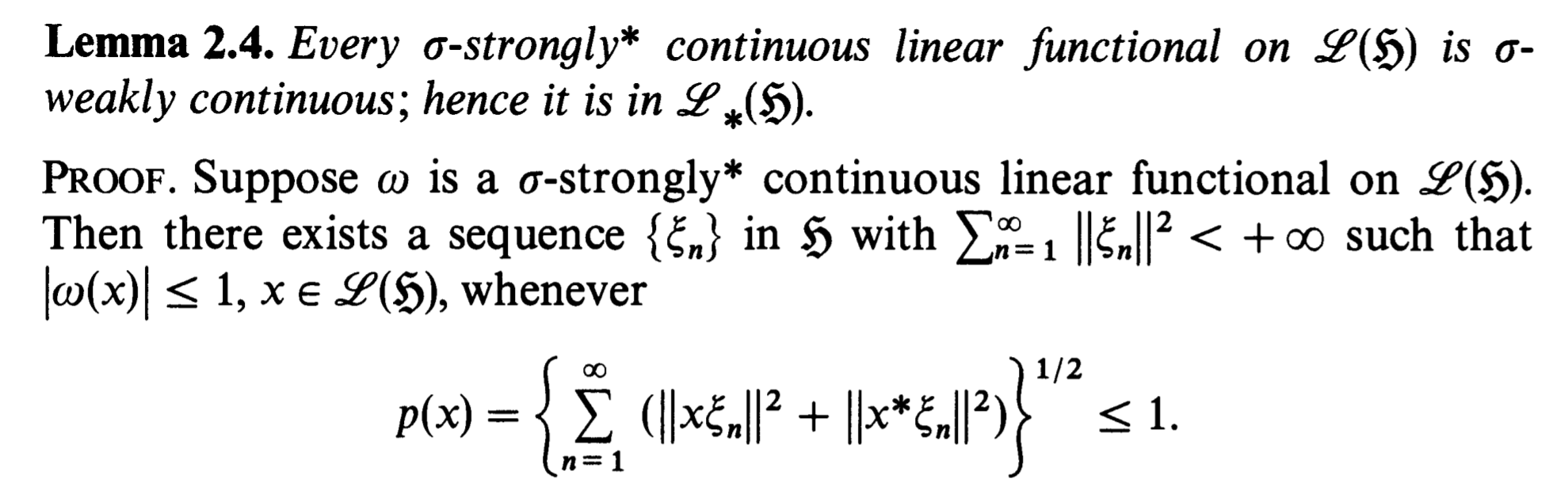So, we are dealing with locally convex topological vector spaces. I think, in general, given a family of seminorms, you would need to consider the finite intersections of the open balls they form, see wikipedia article. So a basic open set about $0$ is of the form
$$ \{ x : p(x)<r \ (p\in F) \} $$
where $F$ is a finite subset of the set $\mathcal P$ of generating seminorms, and $r>0$. However, in the special case of the $\sigma$-strong$^*$-toplogy, this isn't necessary.
In our case, the seminorms are of the form
$$ p_{\xi}(x) = \Big( \sum_{n=1}^\infty \|x\xi_n\|^2 + \|x^*\xi_n\|^2 \Big)^{1/2}, $$
for any sequence $\xi=(\xi_n)$ with $\sum_n \|\xi_n\|^2<\infty$. We can always rescale, so it suffices to take $r=1$ above. Now notice the special property: given $\xi,\eta$, with $\gamma$ the union of the sequences $\xi,\eta$ (say $\gamma_{2n} = \xi_n, \gamma_{2n-1} = \eta_n$) then if $p_\gamma(x) < 1$ then certainly $p_\xi(x)<1$ and $p_\eta(x)<1$. The same argument applies to finite families.
We conclude that for our $\mathcal P$, for any basic open set $U$ about $0$ there is some $p\in\mathcal P$ so that $\{ x : p(x)<1 \}$ is contained in $U$.
A linear functional $f$ is continuous if and only if $f$ is bounded on some open set about $0$. So, with $\mathcal P$ having our property, f is continuous if and only if there is some $p\in\mathcal P$ with $\{ f(x) : p(x)<1 \}$ bounded. Again by rescaling, equivalently, there is some $p\in\mathcal P$ with $|f(x)|\leq 1$ for all $x$ with $p(x)<1$.
Finally, a further standard rescaling argument shows that you can use $p(x)\leq 1$ instead of $p(x)<1$.