In his book [1], Richter-Gebert introduces a notion of stable equivalence for primary basic semialgebraic sets (subsets of $\mathbb{R}^n$ defined by a conjunction of polynomial equations and strict inequalities with rational coefficients) and claims that stably equivalent sets have the same homotopy type. From the rest of the book, it then follows that (among other interesting things) all the homotopy types of primary basic semialgebraic sets occur as realization spaces of 4-polytopes.
Apparently, there have been many different notions of stable equivalence in the literature. He writes
The common idea is that semialgebraic sets that only differ by a "trivial fibration" and a rational change of coordinates should be considered as stably equivalent, while semialgebraic sets that differ in certain "characteristic properties" should not turn out to be stably equivalent. In particular, stable equivalence should preserve the thomotopy type, and respect the algebraic complexity and singularity structure.
My question concerns one kind of map which is used in the definition of stable equivalence, namely stable projection which relates to the "trivial fibration" aspect in the above quote. Richter-Gebert claims in [1, Lemma 2.5.2], that if $W$ stably projects onto $V$, then $W$ and $V$ have the same homotopy type but omits the proof. I would like to know why this is true.
Let me recite the definition. Let $V \subseteq \mathbb{R}^n$ and $W \subseteq \mathbb{R}^{n+m}$ be basic semialgebraic sets with $V = \pi(W)$ where $\pi$ the coordinate projection which deletes the last $m$ coordinates. This projection is stable according to [1, Section 2.5] if there exist polynomials $\phi_i, \psi_j \in \mathbb{Q}[v_1, \dots, v_n, w_1, \dots, w_m]$ (where $i$ and $j$ run over finite, possibly empty index sets) such that (1) the degree of all $\phi_i$ and $\psi_j$ in the variables $w_1, \dots, w_m$ is 1, and (2) $$ W = \{\, (v,w) \in \mathbb{R}^{n+m} : \text{$v \in V$ and $\phi_i(v,w) > 0$ and $\psi_j(v,w) = 0$} \,\}. $$ Thus, all fibers of $\pi$ are relative interiors of rational polyhedra whose defining affine functionals vary polynomially in the image point $v$. In particular they are convex sets and it seems "intuitively clear" that these convex sets may be (uniformly!) deformation-retracted to yield a copy of $V$ inside of $W$, thus proving the homotopy equivalence.
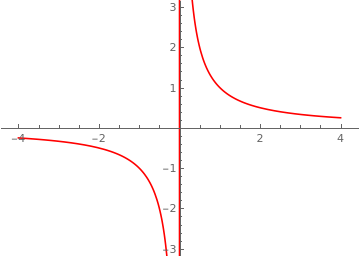
After many discussions about the technicalities behind this idea, my colleague came up with the following example: $$ W = \{\, (v,w) \in \mathbb{R}^2 : v(vw - 1) = 0 \,\}. $$ $W$ is the union of a hyperbola with the $w$-axis. This set projects to the entire $v$-axis and its fibers are all given by a single rational affine-linear equation in $w$ whose parameters polynomially depend on $v$. This is a stable projection per definition, but $W$ has three path-connected components whereas $V$ only has one, which contradicts the homotopy equivalence.
I wonder if I overlooked something. Is this really a counterexample to Richter-Gebert's lemma? If yes, can someone who is more versed in these topological notions (or alternative definitions of stable equivalence) think of a way to repair the definition in a way that preserves the intention of the book: showing homotopy type universality of realization spaces of polytopes?
More concretely, I wonder if there are any additional topological assumptions to make about $\pi$ to ensure that it has local sections around every point in $V$ (that is, for every point $v$ an open neighborhood and a continuous right-inverse for $\pi$ defined on that neighborhood). Since the fibers are convex, I believe that a partition of unity can be used to convex-combine the locally finitely many local sections to obtain a continuous global one, which $W$ then may be contracted to. But finding local sections seems hard especially on points where the rank of the linear system $\Psi_v \cdot w = b_v$ occuring in the definition of the fiber $\pi^{-1}(v)$ is locally non-constant (the rank may increase in every neighborhood of $v$, which is what happens in the above example at $v=0$).
These are observations made by a non-topologist who has run out of colleagues to pester. I would also greatly appreciate pointers to the proper jargon and any literature dealing with problems of this kind.
If I did overlook something and this is not a counterexample, how to prove [1, Lemma 2.5.2] (the homotopy part) properly?