0
$\begingroup$

enter image description here This is from https://www.cambridge.org/core/services/aop-cambridge-core/content/view/CB20539885C03522D141C34024707702/S1446788700014026a.pdf/div-class-title-operator-algebras-with-a-reduction-property-div.pdf

(This is also Proposition 2.3.2 of https://arxiv.org/pdf/1311.3822.pdf

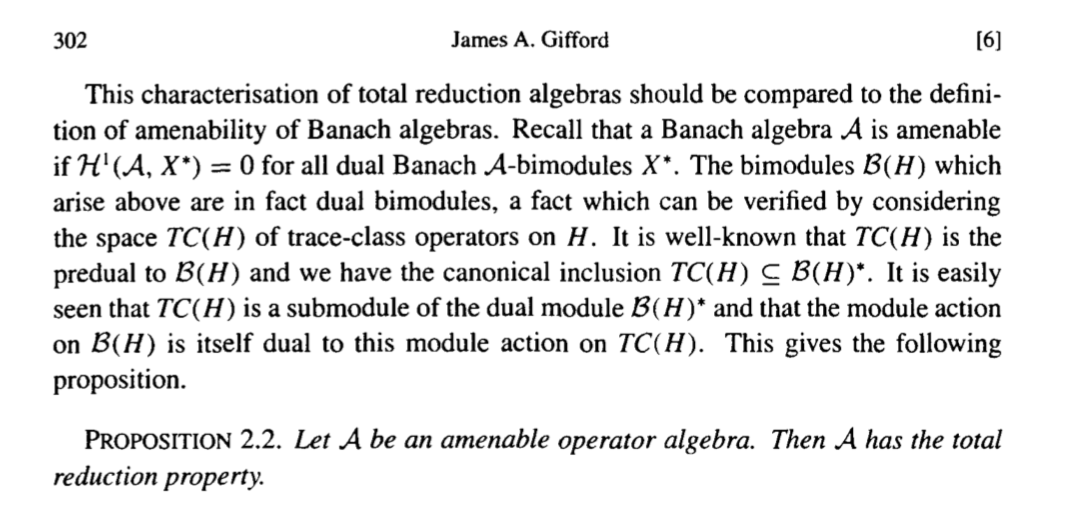
I don't quiet understand how the paragraph above gives the proof of Proposition 2.2. Could someone provide a full proof of this?

Thank you in advance!

Improve this question
$\endgroup$
3
  • 2
    $\begingroup$ That's because the assumption of Theorem 2.1 is satisfied. (Also it's easy to show Proposition 2.2 directly.) $\endgroup$
    – Narutaka OZAWA
    Commented Aug 10, 2021 at 2:07
  • 1
    $\begingroup$ For the benefit of other readers: as Taka has pointed out, Theorem 2.1 characterizes the total reduction property in terms of the vanishing of certain degree-1 cohomology groups. The passage quoted above shows that amenability, as originally defined by Johnson, is equivalent to vanishing of an even larger class of degree-1 cohomology groups. $\endgroup$
    – Yemon Choi
    Commented Aug 10, 2021 at 16:50
  • $\begingroup$ Also, to elaborate on Taka's point that Prop 2.2 can be "proved directly": for most practical/intuitive purposes, the better definition of amenability for Banach algebras is in terms of the existence of bounded approximate diagonals. Once you have such gadgets, there is a simple way to use them to average projections to get projections which respect the algebra action, and hence get the (total) reduction property $\endgroup$
    – Yemon Choi
    Commented Aug 10, 2021 at 16:56

0

Reset to default

You must log in to answer this question.