Thirteen-point conic and four-point line, are they new?

Question

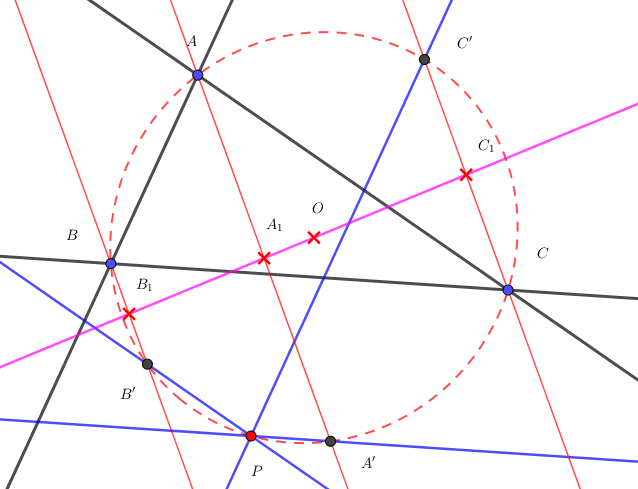
We know that Five points determine a conic and Two Points Determine a Line. Here I found a simple construct of a conic through $7$ points (in PS I note that how the conic through thirteen points) and a line through $4$ points as follows:

Let $ABC$ be a triangle and $P$ be arbitrary point in the plane, let $A'$, $B'$, $C'$ lie on the line through $P$ and parallel to $BC$, $CA$, $AB$ respectively such that $AA' \parallel BB' \parallel CC'$. I am looking for a proof that

• Seven points $A$, $B$, $C$, $A'$, $B'$, $C'$ and $P$ lie on a conic
• Four points: midpoints of $AA'$, $BB'$, $CC'$ and center of the conic are collinear?

PS: Three lines: The line through midpoints of $BC$ and $PA'$, The line through midpoints of $CA$ and $PB'$ and The line through midpoints of $AB$ and $PC'$ are concurrent at the center of the conic (denote the point of concurrence is $O$). Then easily show that reflection of $A, B, C, A', B', C'$ in $O$ also lie on the conic. This mean the conic through $13$ points.

See also:

• Nine-point conic
• Dao's theorem (conics)
• Hexagons with Opposite Sides Parallel

Answer 1

There is a systematic method to solve problems of this sort (the $p,q$ method) by reducing them to computations which can be done by hand. Assume the vertices are $(0,0), (1,0), (p,q)$. The three new points have the form $(x+t(p-1),y+tq), (x-up,y-uq), (x+s,y)$ with three parameters.These can be reduced to one free parameter by the parallelism condition. One then uses the condition on the rank of the matrix with rows $$(x^2 ,xy , y^2 ,x ,y, 1)$$ one for each of the seven points, which ensures that they line on a conic. Now compute the quadratic equation of the corresponding conic (with coefficients which depend on $p,q,x,y$). It is then routine to compute the coordinates of $O$ and verify the collinearity condition.

This is, of course, not as elegant as a (presumable) synthetic proof but it has the advantage of placing the result in a general context. It often suggests possible refinements (in this case I would try replacing the parallelism condition by suitable restraints on the angles between the three directions).