Is it a new method to construction of a conic, how can prove?

Question

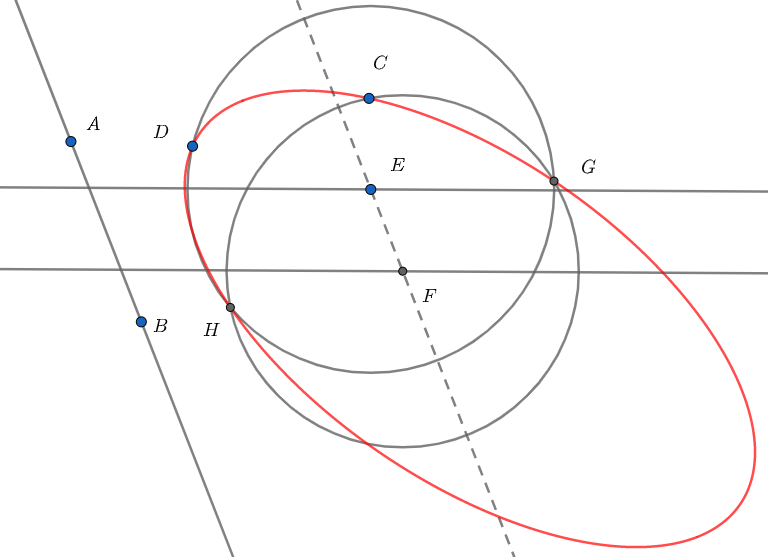
There are some methods to construct a conic, example: Based on Pascal theorem, Steiner construction, .....I propose a method to construct a conic as follows:

Let $L_1, L_2$ be two parallel lines, let $A, B, C, D$ be four points in the plane. Let $E$ be a point lie on the line $L_1$, $F$ be the point lie on line $L_2$ such that $EF \parallel AB$. Let circle $(E, ED)$ meets the circle $(F, FC)$ at two points $H$, $G$.

My question: I am looking for a proof that locus of $H, G$ is a conic section when $E$ be moved on line $L_1$.

See also:

• A chain of six circles associated with a conic

Answer 1

Without loss of generality, let $L_1$ be the x-axis and $t$ be a parameter. Denote $C,D,E,F$ by $$C(x_1,y_1),D(x_2,y_2),E(t,0),F(t+a,b)$$ such that the vector $<a,b>$ is in the same direction as $\overrightarrow{AB}$ and $x_1,y_1,x_2,y_2,a,b$ are constants. Then the locus of $H$ (or $G$) satisfies $$\frac{PE}{ED}=\frac{PF}{FC}=1,$$ where $P(x,y)$ represents $H$ (or $G$). In terms of equations, one has $$(x-t)^2+y^2=(x_2-t)^2+y_2^2\qquad (1)$$ and $$(x-t-a)^2+(y-b)^2=(x_1-t-a)^2+(y_1-b)^2\qquad (2)$$ Then from (1), one gets $$t=\frac{x^2+y^2-x_2^2-y_2^2}{2(x-x_2)}\qquad (3)$$ Subtracting (2) from (1) gives $$(2x-2t-a)(a)+(2y-b)(b)=(x_1+x_2-2t-a)(x_2-x_1+a)+(y_2+y_1-b)(y_2-y_1+b)\quad (4)$$ Now substituting (3) into (4) and clearing denominator, one gets a quadratic equation in $x$ and $y$, so it gives an equation for a conic.