6
$\begingroup$

Can you prove or disprove the following claim?

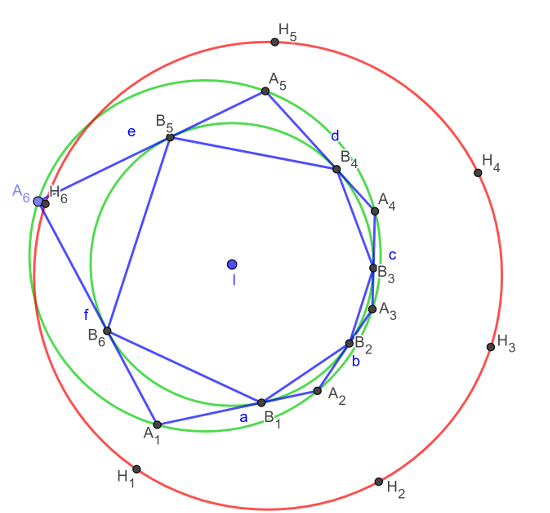
Claim. Let $A_1,A_2, \ldots ,A_n$ be the vertices of an $n$-sided tangential polygon and let $B_1,B_2, \ldots ,B_n$ be the contact points of the inscribed circle and polygon sides such that $B_1$ lies on $A_1A_2$, $B_2$ lies on $A_2A_3$ ,etc. Denote by $H_1,H_2, \ldots,H_n$ the orthocenters of the triangles $\triangle A_1B_1B_n$, $\triangle A_2B_2B_1$,....,$\triangle A_nB_{n}B_{n-1}$ . Then the polygon is cyclic if and only if $H_1,H_2,\ldots ,H_n$ are concyclic.

Picture for the case $n=6$:

enter image description here

GeoGebra applets that demonstrate this claim can be found here , here and here.

Improve this question
$\endgroup$
2
  • $\begingroup$ what is concyclic? $\endgroup$
    – Dima Pasechnik
    Commented Mar 13, 2021 at 12:05
  • $\begingroup$ @DimaPasechnik Concyclic points are the points that lie on the same circle. $\endgroup$
    – Pedja
    Commented Mar 13, 2021 at 12:12

1 Answer 1

Reset to default
8
$\begingroup$

$H_i$ lies on the ray $IA_i$ and $IH_i\cdot IA_i=2r^2$ (where $r=IB_i$), since the midpoint of $IH_i$ is the midpoint of $B_iB_{i-1}$. Hence $H_i$ is the image of $A_i$ under the inversion with respect to center $I$ and radius $\sqrt{2}r$. Thus the result.

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .