Clarification regarding a claim in Heilbronn’s 1934 paper

Question

I was reading Heilbronn’s 1934 paper where he proves that $H(d) \to \infty$ as $d \to -\infty$, where $H(d)$ is the ideal class number of the imaginary quadratic field with discriminant $d$. I couldn't reason out one of his claims in the proof to a lemma. I have attached an image of this part below.

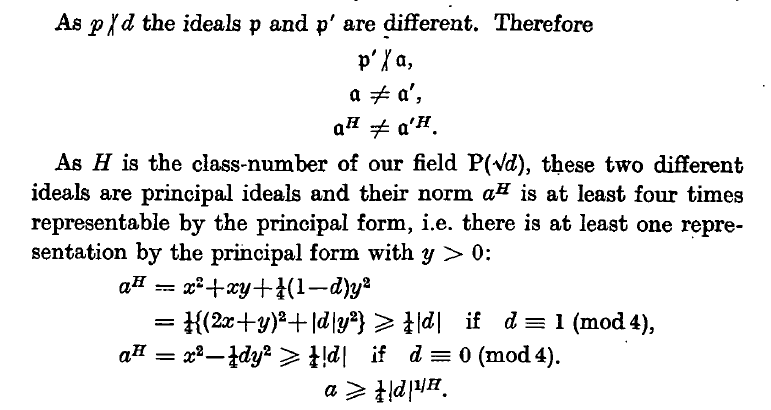
I am struggling with the claim that ".. $a^H$ is at least four times representable by the principal form". I suppose that he is appealing to a lemma which is mentioned earlier in the paper without proof, I state it below in a slightly modified way preceded briefly by some background.

Let $I$ be an ideal in the ring of integers of $K = \mathbb{Q}[\sqrt{d}]$ with $\mathbb{Z}$-basis $(\alpha, \beta)$. Then we may associate $I$ with the form $f_{I, (\alpha, \beta)}(x, y) = \frac{Nm(\alpha x + \beta y)}{Nm(I)}$. It can be proven that this $f_{I, (\alpha, \beta)}$ is an integral primitive binary quadratic form with discriminant $d$, and further that this induces a group isomorphism between the classgroup of $K$ and the proper equivalence classes of forms with discriminant $d$ under certain restricrtions to include only positive definite forms.

The following is the statement of the lemma: If $J$ is any ideal in the same class as $I$ then $Nm(J)$ can be represented by $f_{I, (\alpha, \beta)}$ in two distinct ways.

The following is my proof of this fact: W.l.o.g we may assume that $J = I$. Now since $Nm(I) \in I$, $Nm(I) = \alpha x + \beta y$ for some $x, y \in \mathbb{Z}$. Then $f_{I, (\alpha, \beta)}(x, y) = \frac{Nm(\alpha x + \beta y)}{Nm(I)} = \frac{Nm(Nm(I))}{Nm(I)} = Nm(I)$. And $f_{I, (\alpha, \beta)}(-x, -y)$ is another representation.

Going back to Heilbronn’s claim, I am assuming that since $\mathfrak{a}^H \neq \mathfrak{a'}^H$ and the norm of each of them is representable in at least two different ways by the principal form (based on the lemma above) $a^H = Nm(\mathfrak{a}^H) = Nm(\mathfrak{a'}^H)$ is representable in at least 4 ways.

But my question is that, couldn't it be the case that these representations overlap. For example, suppose $(\alpha, \beta)$ is a $\mathbb{Z}$-basis for $\mathfrak{a}^H$ then $(\alpha', \beta')$ is a $\mathbb{Z}$-basis for $\mathfrak{a'}^H$. Here I use $'$ to denote conjugation. Isn't it possible that $a^H = Nm(\mathfrak{a}^H) = \alpha x + \beta y = \alpha' x + \beta' y = Nm(\mathfrak{a'}^H) = a^H$. In that case, going through the proof of the lemma above, it is easy to see that the representations overlap.

Probably I am missing something here. Would be really helpful if someone could provide a clarification.

Thanks in advance.

Answer 1

One can see more directly that $a^H$ has a representation by the principal form with $y\neq 0$ (hence also with $y>0$). Indeed, let $$\omega:=\begin{cases}\sqrt{d},&d\equiv 0\pmod{4}; \\ (1+\sqrt{d})/2,&d\equiv 1\pmod{4}.\end{cases}$$ Then the ring of integers is $\mathfrak{o}=\mathbb{Z}+\omega\mathbb{Z}$, and the principal form is $(x+\omega y)(x+\omega'y)$. By assumption, $\mathfrak{a}^H$ is a principal ideal, say $\mathfrak{a}^H=\lambda\mathfrak{o}$ with $\lambda\in\mathfrak{o}$. In addition, $\mathfrak{a}^H\neq \mathfrak{a}'^H$, hence $\lambda\neq\lambda'$. Let us write $\lambda$ as $x+y\omega$ with $x,y\in\mathbb{Z}$, then $y\neq 0$, and $$a^H=N(\mathfrak{a}^H)=\lambda\lambda'=(x+\omega y)(x+\omega'y).$$ Done.

P.S. When constructing $f_{I,(\alpha,\beta)}(x,y)$, one needs to use an oriented basis $(\alpha,\beta)$ of $I$. Oriented means that $(\alpha'\beta-\alpha\beta')/\sqrt{d}>0$, or equivalently $(\alpha'\beta-\alpha\beta')/\sqrt{d}=N(I)$.