6
$\begingroup$

My question is the about the equivalence of two definitions of automorphic forms on a semisimple Lie group.

The most common definition of automorphic forms on a semisimple Lie group $G$ with respect to a discrete subgroup $\Gamma$ is given by a smooth function $f:G\to \mathbb{C}$ with the following properties:

  1. left $\Gamma$-invariant,
  2. $K$-finite,
  3. $Z(\mathfrak{g})$-finite,
  4. slowly increasing.

Is this equivalent to the ones defined for $\operatorname{SL}(2,\mathbb{R})$ and $\operatorname{SL}(2,\mathbb{Z})$ where we have an automorphy factor $J(g,z)=cz+d$? I also find a general definition with automorphy factors as follows. [Borel, Armand. "Introduction to automorphic forms." Proc. Symp. Pure Math. Vol. 9. No. 199210. 1966.]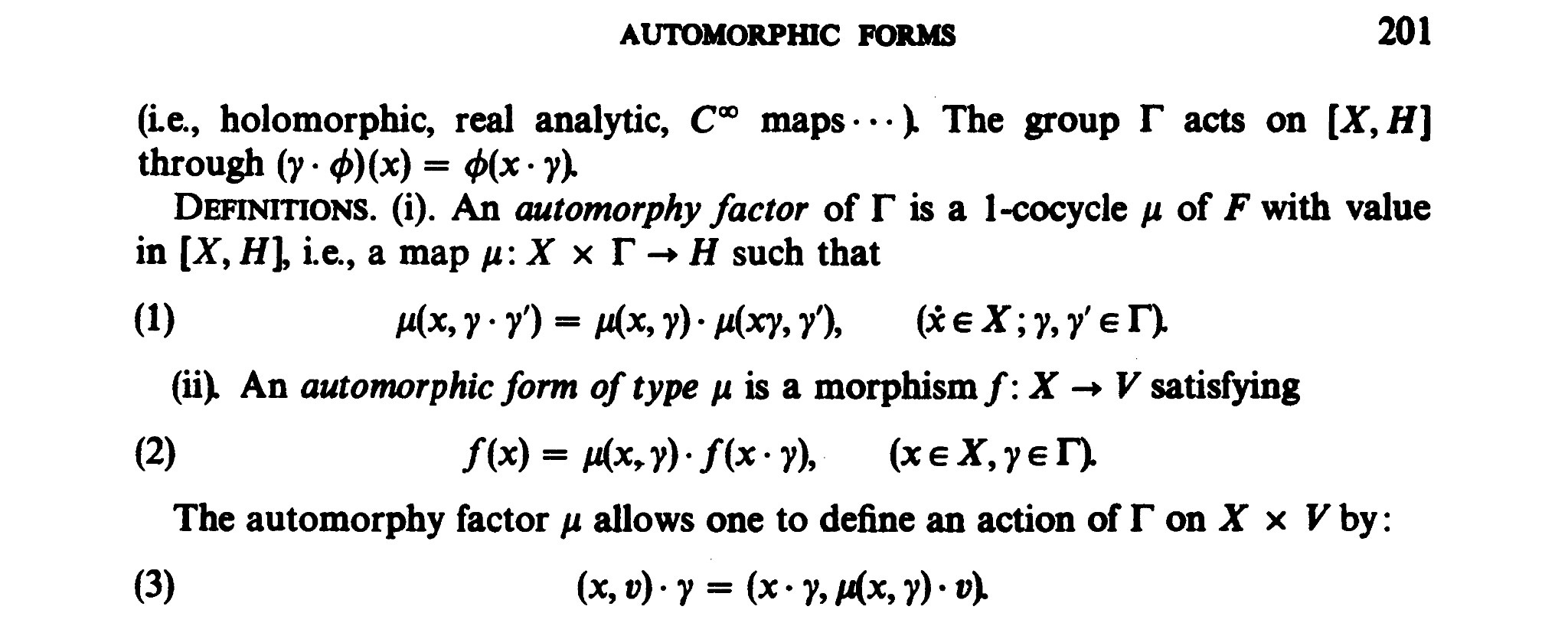enter image description here

I think given a classical automorphic form on $\operatorname {SL}(2,\mathbb{R})$, one could get a $\Gamma$-invariant form by multiplying an automorphy factor $j$. See page 283 of [Bump, Automorphic forms and representations]. So I guess the two definitions differs by such a automorphic factor $j:G/K\times G\to K_\mathbb{C}$.

Improve this question
$\endgroup$
3
  • $\begingroup$ Clarify right or left invariant and finite. For a modular form $f\in M_k(\Gamma)$ with $\Gamma$ a finite index subgroup of $SL_2(\Bbb{Z})$ then $F(\gamma)=(c_\gamma i + d_\gamma)^{-k} f(\gamma . i)$ is an automorphic form $\in C^\infty( \Gamma\backslash SL_2(\Bbb{R}))$ and if $f$ is a cusp form then $F\in L^2( \Gamma\backslash SL_2(\Bbb{R}))$. The right translates of $F$ then generate an Hilbert space $V$ and the right action of $SL_2(\Bbb{R})$ on $V$ is the automorphic representation. $\endgroup$
    – reuns
    Commented Dec 2, 2020 at 22:17
  • $\begingroup$ Does this answer your question? mathoverflow.net/q/124754/6518 $\endgroup$
    – Kimball
    Commented Dec 2, 2020 at 22:54
  • $\begingroup$ $SL(2)$ is ill-formated and should be $\mathrm{SL}(2)$ $\endgroup$
    – YCor
    Commented Dec 3, 2020 at 9:47

1 Answer 1

Reset to default
3
$\begingroup$

There are two notions: automorphic (or modular) form on a domain $G/K$, which would potentially have an "automorphy factor/cocycle" $\mu:\Gamma\times G/K\to \mathbb C^\times$ (or to a larger $GL_n$ for vector-valued automorphic forms), and automorphic forms on_a_group $G$, which have no cocycle, but instead are left $\Gamma$-invariant, and right $K$-equivariant.

When the cocycle extends from $\Gamma\times G/K$ to $G\times G/K$, the procedure in Borel's Boulder article gives an automorphic form on the group attached to an automorphic form on the domain.

A meaningful instance of a cocycle not extending from the discrete subgroup $\Gamma_o(4)$ to the natural ambient Lie group $SL_2(\mathbb R)$ is the example of half-integral-weight automorphic forms "on the upper half-plane", as in G. Shimura's papers around 1973.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Thank you. I think you mean that a given automorphic on the domain can NOT always extend to the one on a group. But can we get the one on a domain from the one defined on the group? I guess we can just divide it by a cocycle as $F(g)=j(g,i)f(i)$ for $G=SL_2(\mathbb{R})$ (here $i$ is just the coset $0\in G/K$). BTW: Your short notes help me quite a lot. Thank you so much! $\endgroup$
    – Jun Yang
    Commented Dec 4, 2020 at 2:07

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .