A random variable whose characteristic function decreases the fastest

Question

A random variable $X$ is "good" for $(a_0, b_0) \in (0,1)^2$ if its characteristic function $\varphi_X(t)$ satisfies the following constraints:

1. $\forall t : \varphi_X(t) \geq 0$.
2. $\varphi_X$ is monotonically decreasing on $\mathbb{R}_{+}$.
3. $\varphi(a_0) = b_0$.

Given $(a_0, b_0)$ and $a_0 < x < 1$, I would like to find a random variable $X^*$ which is "good" for $(a_0, b_0)$ and whose characteristic function $\varphi_X$ has the lowest value at $x$, i.e. for any random variable $X'$ which is good for $(a_0, b_0)$ it holds that $\varphi_X(x) \leq \varphi_{X'}(x)$.

My conjecture is that a Gaussian satisfies this optimality criterion. My idea of proving it was thinking about the connections between $\varphi_X(t)$ and the entropy of $X$ ($h(X):= \mathbb{E}[-\log(X)]$): it is known that a Gaussian maximizes the entropy, so if it can be shown that $\varphi_X(t)$ decrease in a rate depends on the entropy, perhaps the claim would follow. However I couldn't obtain a more rigorous claim.

Answer 1

Your minimization problem is clearly a problem of infinite-dimensional linear programming. Usually, such problems do not have a closed-form solution. Your problem, too, seems unlikely to have such a solution in full generality; however, see Remark 1 at the end of this answer.

One thing that can be definitely said is that your conjecture "that a Gaussian satisfies this optimality criterion" is false. Indeed, for any $(a_0,b_0)\in(0,1)^2$, let $c:=c_{a_0,b_0}:=-a_0^{-2}\ln b_0$, so that $c$ is the positive real root of the equation $\phi(a_0)=b_0$, where $\phi(t):=\phi_c(t):=e^{-ct^2}$. Then $\phi$ is a characteristic function and your conditions 1--3 are satisfied with $\phi$ in place of $\varphi=\varphi_X$.

Let now $\psi(t):=\psi_k(t):=\max(0,1-|t|/k)$ for all real $t$, where $k:=k_{a_0,b_0}:=a_0/(1-b_0)$ is the only root of the equation $1-a_0/k=b_0$. Then $\phi$ is a characteristic function and your conditions 1--3 are satisfied as well with $\psi$ in place of $\varphi=\varphi_X$.

However, $k>a_0$ and $\psi(k)=0<\phi(k)$. $\Box$

Remark 1: This also shows that for each $t\ge k=k_{a_0,b_0}$ the function $\psi_k$ is a minimizer of $\varphi(t)$ over all characteristic functions $\varphi$ satisfying your conditions 1--3.

Moreover, note that $t=a_0$ is a positive real root of the equation $\phi_c(t)=\psi_k(t)$. The latter equation may have one or two positive real roots. Let $a_{\max}$ be the largest root of the equation $\phi_c(t)=\psi_k(t)$. It follows that $a_{\max}<k$ and for each $t>a_{\max}$ we have $\psi_k(t)<\phi_c(t)$. In particular, if $a_{\max}=a_0$ (that is, if $a_0$ is the largest root of the equation $\phi_c(t)=\psi_k(t)$), then $a_0<k$ and for each $t\ge a_0$ we have $\psi_k(t)<\phi_c(t)$.

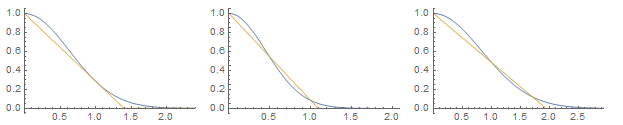
The condition $a_{\max}=a_0$ can be rewritten as $\psi'_k(a_0)\le\phi'_c(a_0)$ (with $c=c_{a_0,b_0}$ and $k=k_{a_0,b_0}$), and then further as $0<b\le b_*$, where $b_*=0.28466\dots$ is the only root $b\in(0,1)$ of the equation $1-b=2b\ln\frac1b$; the necessary and sufficient condition $0<b\le b_*$ for $a_{\max}=a_0$ does not involve the scaling parameter $a_0$ (and of course it should not).

For $a_0=1$, here are the graphs $\{(t,\phi_c(t))\colon t\in[0,k+1)\}$ (blue) and $\{(t,\psi_k(t))\colon t\in[0,k+1)\}$ (gold) (i) for $b=b_*$ and hence $a_{\max}=a_0[=1]$ (left); (ii) for $b=b_*-2/10$ and hence $a_{\max}=a_0[=1]$ (middle); and (ii) for $b=b_*+2/10$ and hence $a_{\max}>a_0[=1]$ (right):