A question on $a_i(n) = a_i(\pi(n)) + a_i(n-\pi(n))$ with $a_i(n) = 1$ for $n \le i$

Question

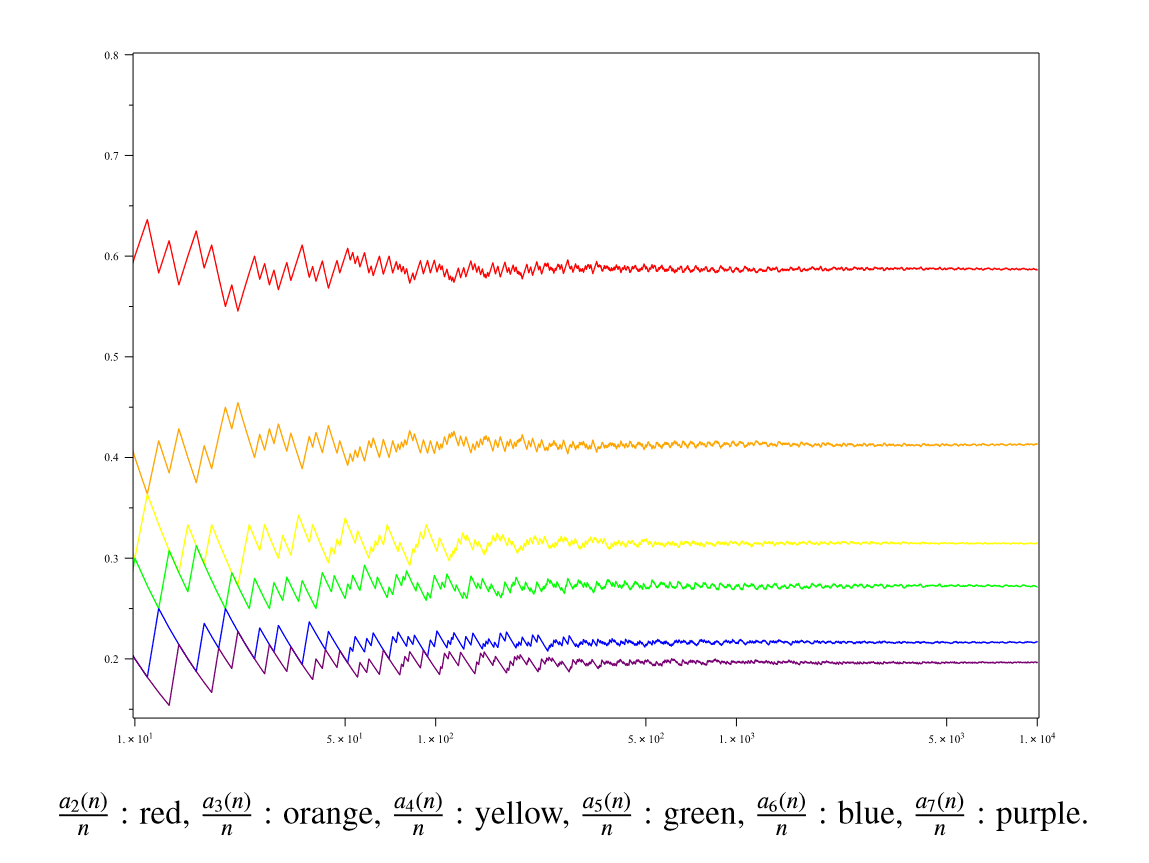
Let $a_i(n) = a_i(\pi(n)) + a_i(n-\pi(n))$ with $a_i(n) = 1$ for $n \le i$ where $\pi(n)$ is the prime-counting function.

By definition, it is obvious that $a_1(n) = n$ and $a_2(n)$ is https://oeis.org/A316434.

Question. Does $\lim_{n\to\infty}\frac{a_i(n)}n$ exist for any fixed value of $i \ge 2$ ?

(Below plot shows initial values of $\frac{a_i(n)}n$ for $2 \le i \le 7$.)

Thanks.