Let $s$ be the (“square wave”) $1$-periodic real function such that $s(x) = 1$ if $0<x<\frac{1}{2}$ and $s(x) = -1$ if $\frac{1}{2}<x<1$ (and maybe $s(0)=s(\frac{1}{2})=0$ for the sake of definitiveness, but I'll only be using $s$ a.e.). In $L^2(\mathbb{R}/\mathbb{Z})$, we have $$ s(x) = \frac{4}{\pi} \sum_{m=0}^{+\infty} \frac{\sin(2(2m+1)\pi x)}{2m+1} \tag{*} $$ by a straightforward computation of its Fourier coefficients. Now the Fourier coefficients in question are multiplicative, so I'd like to use the Möbius inversion formula to reconstruct the sine wave using the square waves $s(kx)$, namely, I would like to state: $$ \frac{4}{\pi} \sin(2\pi x) = \sum_{m=0}^{+\infty} \frac{\mu(2m+1)\,s((2m+1)x)}{2m+1} \tag{†} $$ where $\mu$ is the Möbius function. Unless I am mistaken, (†) is clear if we just demand weak $L^2$ convergence of the RHS. But I don't know how to say more. Specifically:
Main question: Is there (norm) $L^2$ convergence in (†)?
Bonus points: What can be said about convergence almost everywhere? For even more credits, what can be said about convergence for a particular $x$ (maybe every $x\not\in\mathbb{Q}$)? For super extra credits, discuss uniform convergence (I know the Gibbs phenomenon prevents uniform convergence in (*), but I don't see why this would forbid it in (†)).
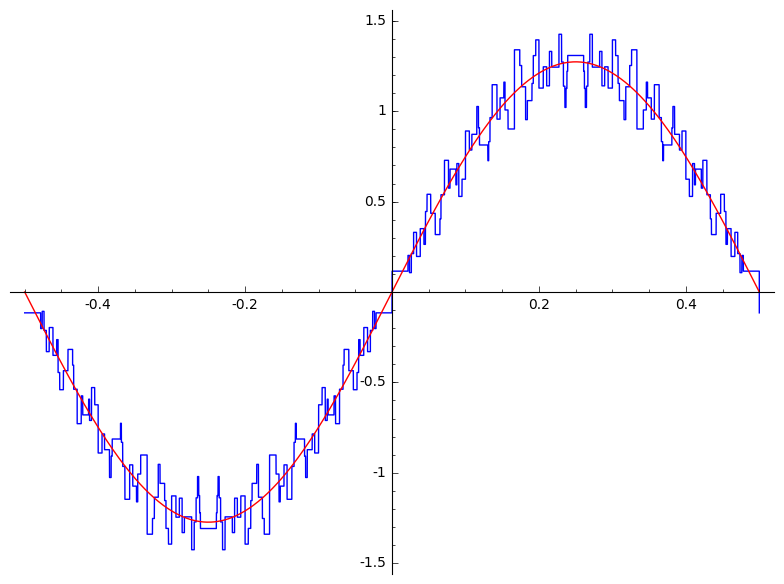
Here is a graph of the partial sum for $m\leq 12$ of the RHS of (†):
Remarks:
Graphs, as well as a rendering as a sound wave (which is interesting, if not very pleasant to listen to) of the first few partial sums of the RHS of (†) are given in this Twitter thread.
Lest anyone make the same mistake I made when thinking about this problem at first, the $s(kx)$ are not orthogonal in $L^2(\mathbb{R}/\mathbb{Z})$. (In fact, applying Gram-Schmidt to the $s(kx)$ produces a rather unexpected sequence of functions: see here for some graphs.)
I have a feeling this might be related to the question (which has independent interest) of finding an upper bound on $\sum_{k|n,\;k\leq B\;}\mu(k)$ as a function of $n$ and independently of $B$. Maybe I should ask this as a separate question?
The fact that the value at $0^+$ of the RHS of (†) converges to $0$ is equivalent to the prime number theorem, so the extra credits probably depend on some number theory, but I don't know what to think about mere $L^2$ convergence.