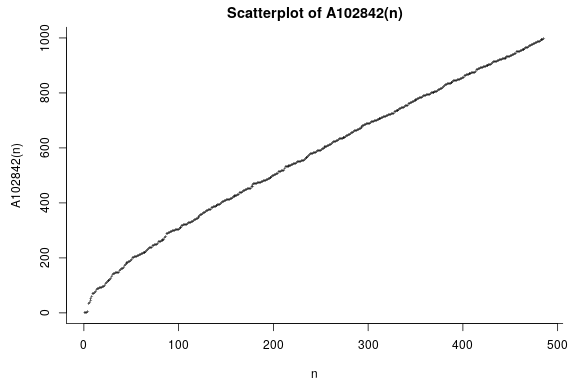
A number $n$ is called insipid if the groups having a core-free maximal subgroup of index $n$ are exactly $A_n$ and $S_n$. There is an OEIS enter for these numbers: A102842. There are exactly $486$ insipid numbers less than $1000$.
Question: Are there infinitely many insipid numbers?
Let $\iota(r)$ be the number of insipid numbers less than $r$. The following plot (from OEIS) leads to:
Bonus question: Is it true that $\lim_{r \to \infty}r/\iota(r)=2$?