$B=(B_t,t\in[0,1])$ a standard brownian motion on $[0,1]$.
For $t\in[0,1]$, we define $$\mathcal{F}_t=\sigma(B_s,s\in[0,t]),$$ $$\mathcal{G}_t=\mathcal{F}_t\,\vee\,\sigma(B_1).$$ How can we show $$\mathbb{E}[B_t-B_s|\mathcal{G}_s]=\frac{t-s}{1-s}(B_1-B_s)$$
(I'm changing your notations a bit, because I want to call $B$ a Brownian bridge.)
Fix $0<s\leq1$, and let $W$ be a standard Brownian motion indexed by $[0,1]$. I call the (law of the) random variable $u\in[0,s]\mapsto W_u-\frac usW_s$ a Brownian bridge of size $s$. It is but the Brownian motion modified to be $0$ at $s$, by subtracting a linear function.
I claim that the "superposition" of a Brownian bridge $B$ of size $s$ and a normal variable $N$ of variance $1$ is a Brownian motion on $[0,1]$: here, superposition means the process $$u\mapsto B_u+uN\text.$$ One can prove this fact by noting that such a process is Gaussian, and has the desired finite dimensional covariances.
Now for what you're asking: the Brownian motion $W$ can be decomposed in for independent variables (see image below):
(To prove this, I would go the other way around and show that given such random independent variables, the "superposition" is Gaussian and has the required covariance.)
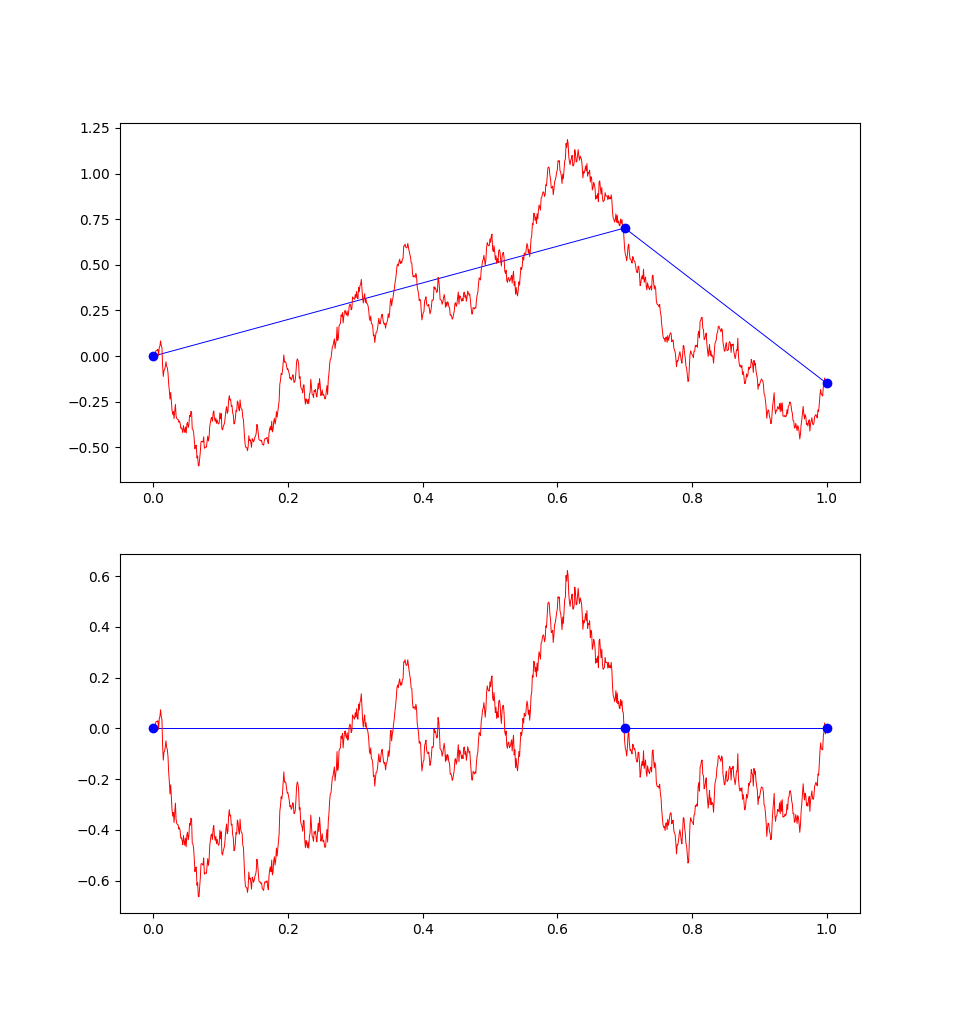
Once this is done, we see that $$\mathcal{G}_t = \sigma(N,N',B)$$ and so $$ \mathbb{E}[W_t-W_s|\mathcal{G}_s] = \mathbb{E}\left[B'_{t_s} + \frac{t-s}{1-s}N'\middle|\mathcal{G}_s\right] = \frac{t-s}{1-s}N' = \frac{t-s}{1-s}(B_{1-s}-B_s)\text. $$
