3
$\begingroup$

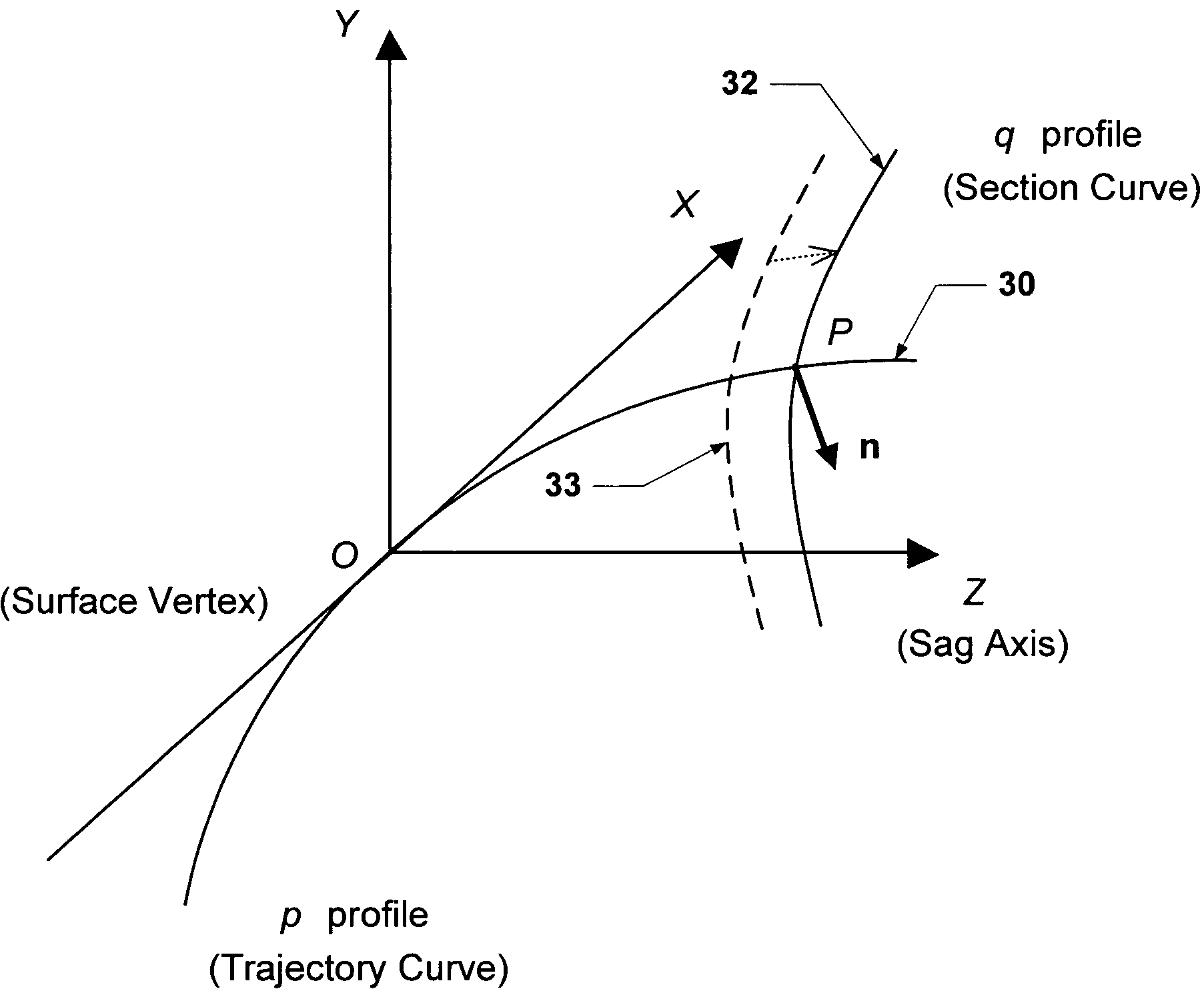
I'm looking for a general equation/function z = f(x, y, radius1, radius2, p1, p2) for an atoric surface. p1 and p2 could be either eccentricity or conic constant values. Can anyone help me with that?

In case it is not clear what kind of surface it is, it's similar to a toric surface (so with spherical cross sections in two perpendicular axes where each has its own radius), but now the cross section won't be spherical anymore. Instead, each principal axis has each its own eccentricity or conic constant, causing the cross section to be a parabola, hyperbola, ellipse or a circle (when conic constant equals 0).

I'm not necessarily looking for the complete 3D surface of a solid. A surface with the above mentioned properties is good enough for me.

If I picked the wrong tags, please tell me.

Improve this question
$\endgroup$
8
  • 1
    $\begingroup$ A quick Google search suggests that the term "toric surface" is used mostly in optical engineering (lenses). Also, I do not understand what you mean by a surface to have "spherical cross section". $\endgroup$
    – Alex M.
    Commented Jul 31, 2018 at 12:35
  • 2
    $\begingroup$ By "toric surface" I initially thought you meant a two-dimensional toric variety, especially given the algebraic geometry tag, but now I suspect you mean something like the illustration for the article "toric lens" on Wikipedia. Is that what you're talking about? $\endgroup$
    – Daniel McLaury
    Commented Jul 31, 2018 at 12:37
  • $\begingroup$ Oh, are you just saying that you want the equation for the surface swept out by dragging a conic section along a path that is itself a conic section? $\endgroup$
    – Daniel McLaury
    Commented Jul 31, 2018 at 12:41
  • 1
    $\begingroup$ A general parametric equation for an atoric surface can be found in this patent description (equations 1 and 2 in the section "description of the invention"). $\endgroup$
    – Carlo Beenakker
    Commented Jul 31, 2018 at 12:41
  • $\begingroup$ I changed the tags. If you still think the original ones were more descriptive of the question, you're of course welcome to restore them. $\endgroup$
    – Qfwfq
    Commented Jul 31, 2018 at 13:25

1 Answer 1

Reset to default
2
$\begingroup$

For reference, here is the description of the atoric surface described in Spectacle lenses incorporating atoric surfaces.

The profile lies in the $x$–$z$ plane and is parameterised by two variables $u,v$: $$x(u,v)=u+a(u)q(v),\;\;y(u,v)=v,\;\;z(u,v)=p(u)+\gamma(u)q(v)$$ $$p(u)=\frac{c_p u^2}{1+\sqrt{1-\epsilon c_p^2 u^2}}+{\cal O}(u^4),\;\;q(v)=\frac{c_q v^2}{1+\sqrt{1-\epsilon c_q^2 v^2}}+{\cal O}(v^4),$$ and $n=(a(u),0,\gamma(u))$ is an inward unit normal vector of the $p(u)$ profile, as indicated in the figure:

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .