How many points appear in the plane when the chain of n-gons is close?

Question

Let $A_{11}A_{12}\cdots A_{1n}$ be a regular $n$ polygon, we call $A_{11}A_{12}\cdots A_{1n}$ is the $1st-n-gons$. Now we construct the $2nd-n-gon$ based two condition as follows:

1. $2nd-n-gons$ is regular $n-gons$ with a side $A_{1n}A_{11}$.

2. $2nd-n-gons$ and $1st-n-gons$ with the same orientation.

Let $2nd-n-gons$ is $A_{21}A_{22}A_{23}\cdots A_{2n}$ where $A_{21}=A_{1n}$ and $A_{22}=A_{11}$

Construct $3rd-n-gons$ based two condistions as follows:

1. $3rd-n-gons$ is regular $n-gons$ with a side $A_{2n}A_{11}$.

2. $3nd-n-gons$ and $1st-n-gons$ with the same orientation.

Let $3rd-n-gons$ is $A_{31}A_{32}A_{33}\cdots A_{3n}$ where $A_{31}=A_{2n}$ and $A_{32}=A_{11}$

Construct the $kth-n-gons$ are similarly construction of $2nd-n-gons$ and $3rd-n-gons$. Then the chain of these gons must be close.

My question: How many points appear in the plane when the chain of n-gons is close?

Example:

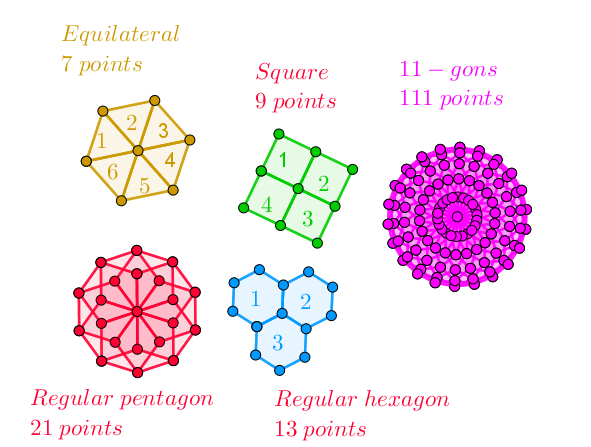
• $n=3$, construct 6 equilaterals then these chain is close and the number of points is $7$

• $n=4$, construct 4 squares then these chain is close and the number of points is $9$

• $n=5$, construct $10$ regular pentagons then these chain is close and the number of points is $21$

• $n=6$, construct $3$ regular hexagons then these chain is close and the number of points is $13$

• $n=11$, construct $22$ time, $11-gons$ then these chain is close and the number of points is $111$

See also

• Figurate Numbers

Answer 1

We start by determining how many repetitions of this process are done. The rotation is by the interior angle $\theta := \pi - \frac{2 \pi}{n} = \frac{n - 2}{n} \pi$, and we want to know for which $k$ we have that $k \theta$ is an integer multiple of $2\pi$. Equivalently, we want to know the denominator of the reduced form of $\frac{n-2}{2n}$.

If $n$ is odd, $k = 2n$. If $n$ is even and not divisible by $4$, $k = \frac{n}{2}$. If $n$ is divisible by $4$, $k = n$.

Each point will then have $k$ "copies", each rotated from the original by an integer multiple of $\frac{2\pi}{k}$ (except the central one, which only appears as itself). We can't simply count using the original equilateral, though, as some pairs of vertcies on the original equilateral will "pair up", and one may be a "copy" of another (as the "first" point always will, by definition).

So our next job is to determine when one vertex will be a "copy" of another.

Two vertices can "copy" each other only if they are the same distance from the center. This restricts us to $A_i$ and $A_{n+2-i}$ for each $i$. But even then, they do not always "copy" each other (as can be seen by the hexagon).

Claim: $A_i$ and $A_{n+2-i}$ fail to "copy" each other if and only if $n$ is even but not divisible by $4$ and $i$ is odd.

Proof: By using inscribed angles, we can see that the angle $A_i A_1 A_{n+2-i}$ is $\pi - (i-1)\frac{2\pi}{n}$. The copies of $A_i$ appear every $\frac{2\pi}{k}$ rotation around $A_1$, so $A_i$ and $A_{n+2-i}$ "copy" each other if and only if $\pi - (i-1)\frac{2\pi}{n}$ is an integer multiple of $\frac{2\pi}{k}$. Equivalently, they copy if and only if $\frac{k (n+2-2i)}{2n}$ is an integer. If $n$ is odd, $k=2n$, so this is true. If $n$ is divisible by $4$, then $k=n$ and $n+2-2i$ is even, so this is true. If $n$ is even but not divisible by $4$, then $k=\frac{n}{2}$, so this is about whether $n+2-2i$ is divisible by $4$. It is if and only if $i$ is even, so the claim is proven.

We can now answer the question by counting. If $n$ is odd, the answer is $1 + n(n-1)$. If $n$ is divisible by $4$, the answer is $1+\frac{n^2}{2}$. If $n$ is even but not divisible by $4$, the answer is $1 + (\frac{n}{2})(\frac{3n-2}{4})$.