Parabolic envelope of fireworks

Question

The envelope of parabolic trajectories from a common launch point is itself a parabola. In the U.S. soon many will have a chance to observe this fact directly, as the 4th of July is traditionally celebrated with fireworks.

If the launch point is the origin, and the trajectory starts off at angle $\theta$ and velocity $v$, then under unit gravity it follows that the parabola $$ y = x \tan \theta - [x^2 /(2 v^2)] (1 + \tan^2 \theta) $$ and the envelope of all such trajectories, is another parabola: $$ y = v^2 /2 - x^2 / (2v^2) $$

---

             

---

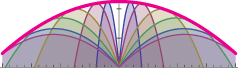
These equations are not difficult to derive. I have two questions. First, is there a way to see that the envelope of parabolic trajectories is itself a parabola, without computing these equations? Is there a purely geometric argument? Perhaps there is a way to nest cones and obtain the above picture through conic sections, but I couldn't see it.

Second, of course the trajectories are actually pieces of ellipses, not parabolas, if we follow the true inverse-square law of gravity. Is the envelope of these elliptical trajectories also an ellipse? (I didn't try to work out the equations.) Perhaps the same geometric viewpoint (if it exists) could apply, e.g. by slightly tilting the sections.

Answer 1

• E. Torricelli, who was the last Galileo's secretary, suggested a purely geometrical method to find the envelope in his De motu Proiectorum. He also coined the term `parabola of safety'. Apparently it was the first example of computation of an envelope. The method is briefly described in this note.

• Another approach is to launch identical missiles with the same velocity at all possible angles simultaneously. At time $t$, their positions describe a circle $$x^2+\left(y-\frac{t^2}{2}\right)^2=(vt)^2.$$ The latter equation has a unique solution in $t$ provided $(x,y)$ belongs to the parabola $$y=\frac{v^2}{2}-\frac{x^2}{2v^2}.$$

• In the case of missiles moving in a Kepler field (with the attractive potential $\sim -1/r$), the envelope of elliptic trajectories is indeed an ellipse. A web search gave the nice short article which contains several elementary geometric proofs of this and related results.

Edit. A free version of J.-M. Richard article can be found here.

Answer 2

It is easy to see that all these parabolas have the same directrix. Height of a directirix correspond to energy of the body. So you have the family of parabolas with the common point $P$ and the directrix $l$. It is easy to prove, (using just definition of parabola as a locus of points...) that all of the touched the parabola with the focus at $P$ and the directrix $l_1$, which parallel $l$ (actually $l$ is midline of $P$ and $l_1$).

The same holds for sun-earth set. If Earth decides to fly in other direction (but with the same speed) its path will be always touch the fixed ellipse with foci in Sun and this position of Earth.

Answer 3

I found another article to supplement those to which Andrey linked: Eugene I Butikov, "Comment on 'The envelope of projectile trajectories'," Eur. J. Phys. 24 L5-L9, 2003. He also explains the expanding-circles viewpoint that is Andrey's second bullet. He imagines first that there is no gravity, in which case the particles are on the surface of an expanding sphere whose radius $r$ equals $v t$. "With gravity, this uniformly expanding sphere is falling freely as a whole with the acceleration of free fall." He then finds the envelope of these falling, expanding circles.

Later in the note he considers water drops spun off a spinning, wet bicycle wheel. To continue the 4th-of-July theme, these could be sparks from a spinning sparkler wheel. He proves that again, the envelope of the drops/sparks is a parabola.