2
$\begingroup$

Take two fixed points in a plane and a triangle of fixed shape. Constrain two sides of the triangle to each touch one of the two points. As the triangle moves under this constraint the third side forms an envelope curve. Is this curve just an elliptical arc or something else?

This question arises in "lost in a forest" type problems.

Improve this question
$\endgroup$
2
  • 1
    $\begingroup$ Are you saying something like this?: "There is a movable rigid triangle $ABC$ and fixed points which start as $P=A$ and $Q$ on $AB.$ We slide the triangle around keeping $Q$ on the line $AB$ and $P$ on the line $AC$ until $A$ arrives at $Q.$ Find the envelope of all the points on edge $BC.$"? or is that not quite it? $\endgroup$
    – Aaron Meyerowitz
    Commented May 14, 2016 at 21:30
  • $\begingroup$ That is exactly what I meant. Thank you for clarifying. $\endgroup$
    – Philip Gibbs
    Commented May 14, 2016 at 22:48

3 Answers 3

Reset to default
3
$\begingroup$

Not an answer, just an illustration.

                Tri2Pts

Improve this answer
$\endgroup$
1
  • 1
    $\begingroup$ Yes, that's how it would look and you can see the envelope forming. If the edges of the triangle are continued beyond the vertices it should be possible to turn the triangle through 360 degrees so that the envelope forms a closed curve. $\endgroup$
    – Philip Gibbs
    Commented May 14, 2016 at 17:29
1
$\begingroup$

By elementary differential geometry, the locus is an arc of a circle.

Improve this answer
$\endgroup$
2
  • 1
    $\begingroup$ Thanks you are right. I found a geometric proof. The centre of the circle and the two fixed points form a triangle similar to the one being moved. The vertex of the triangle moves round its circumscribed circle. $\endgroup$
    – Philip Gibbs
    Commented May 14, 2016 at 21:56
  • 1
    $\begingroup$ @PhilipGibbs: It would be useful if one of you would prove the claims (which I do not doubt). $\endgroup$
    – Joseph O'Rourke
    Commented May 15, 2016 at 2:22
1
$\begingroup$

With thanks to @Joseph O'Rourke and @goleta who gave me the clues I will post a solution to my own question. I will use the nomenclature for the points provided by @Aaron Meyerowitz.

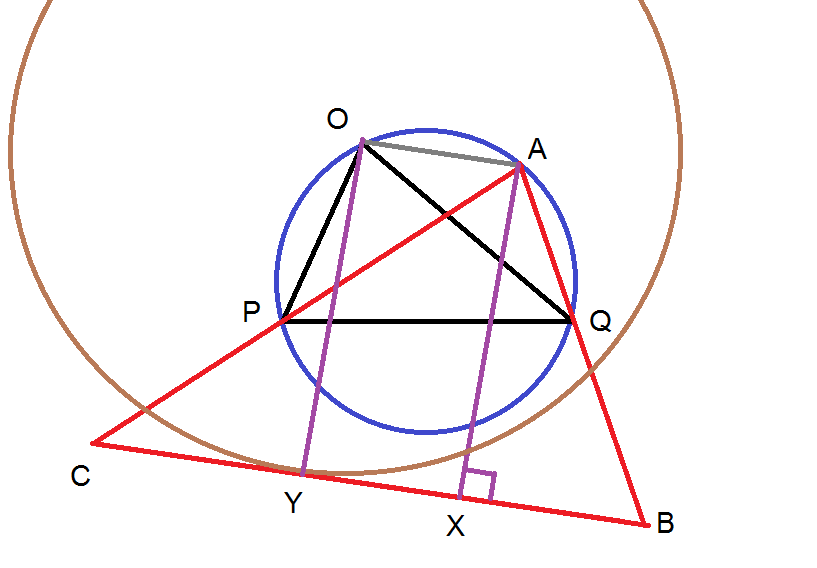enter image description here Construct a triangle $OPQ$ with the given side $PQ$ such that it is similar to the reflection of the given triangle $ABC$ i.e. $\angle OPQ$ = $\angle ABC$ and $\angle OQP$ = $\angle ACB$.

Draw the circumscribed circle of triangle $OPQ$. Because $\angle POQ$ = $\angle CAB$ = $\angle PAQ$ it follows that $A$ must also lie on this circle (angles subtended from points on a circle by a chord of that circle are equal)

Also, $\angle OAP$ = $\angle OQP$ = $\angle ACB$, therefore $OA$ is parallel to $CB$. Therefore the distance $OY$ from the point $O$ to the side $BC$ is equal to the distance $r$ from the point $A$ to the side $BC$ and this is the fixed height of the triangle. Therefore the side $BC$ is tangent to the circle with centre $O$ and radius $r$ and the envelope of the edge must be an arc of that circle.

If anyone knows a prior proof of this please comment so that I can cite it.

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .