Can you point out a reference for the fact that solutions for the initial-boundary value problem associated to $$\partial_t u + \partial^4_x u = 0$$ with $u(0,\cdot) >0$ can change sign (that is, need not be positive)?
1 Answer
For a delta-function initial condition, $u(x,0)=\delta(x)$, the solution follows upon Fourier transformation, $$u(x,t)=\frac{1}{\pi}\int_0^\infty e^{-k^4 t} \cos kx\,dk .$$ This is a hypergeometric function which oscillates around zero, see the plot of $u(x,t)$ for $t=0.01,0.1,1$.

The delta-function initial condition is not strictly positive, but we can alternatively choose a gaussian $u(x,0)=\exp(-ax^2)$ upon convolution: $$u(x,t)=\frac{1}{\pi}\int_{-\infty}^\infty \int_0^\infty e^{-ax'^2}e^{-k^4 t} \cos k(x-x')\,dk dx'.$$ The sign change persists, see plot for $a=10$, $t=0.01$.
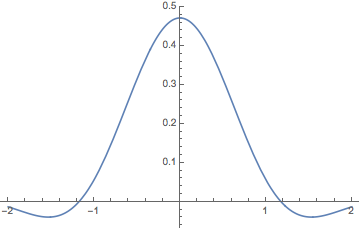
The OP asks for a reference, for example, the figure above is similar to figure 1 of this 2008 paper.
-
$\begingroup$ It seems the cosine in the integrand is the one causing the change sign, irrelevant of the positive initial function. I don't why there would be a specific first reference that discusses this problem. $\endgroup$– AlanCommented May 4, 2018 at 18:38
-
$\begingroup$ @Alan ---no, it's not that trivial; suppose you replace the $k^4$ in the exponent by $k^2$; the cosine is still there, but now it is indeed true that a positive initial distribution remains positive; that is just what you would expect for a diffusion equation, the density cannot become negative; so it is somewhat remarkable that by going from second derivative with respect to $x$ to fourth derivative the positivity is lost. $\endgroup$ Commented May 4, 2018 at 19:25
-
$\begingroup$ How do you then prove these two claims in your last comment? $\endgroup$– AlanCommented May 4, 2018 at 20:48
-
$\begingroup$ you mean a proof that the solution of the diffusion equation $\partial_t u-\partial_x^2 u=0$ stays positive for all times? this follows from the fact that the Fourier transform with respect to $k$ of $e^{-k^2 t}$ is again a Gaussian, and the convolution of any positive initial distribution with a Gaussian remains positive. Replacing the $k^2$ by $k^4$ spoils that, you get this hypergeometric function that changes sign. $\endgroup$ Commented May 4, 2018 at 21:01
-
1$\begingroup$ the integrand is positive, so the integral must also be positive: $u(x,t)=t^{-1/2}\int u(x',0)\exp[(x-x')^2/4t]\,dx'>0$ when $u(x,0)>0$ for all $x$. $\endgroup$ Commented May 5, 2018 at 0:37
