Since Brahmagupta's formula and Bretschneider's formula we have the inequality:
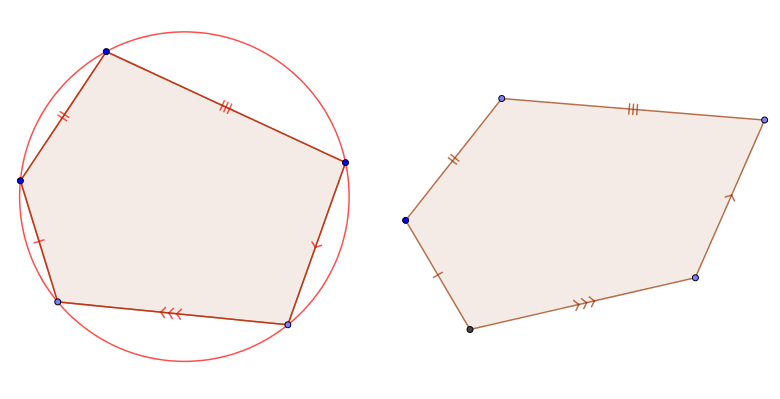
Any two quardrilaterals $A_1A_2A_3A_4$ and $B_1B_2B_3B_4$ with the same sidelengths and $A_1A_2A_3A_4$ is a cyclic but $B_1B_2B_3B_4$ is not a cyclic then area of $A_1A_2A_3A_4$ $\ge$ area of $B_1B_2B_3B_4$
Isoperimetric inequality state that:
For the length L of a closed curve and the area A of the planar region that it encloses, that $L^2 \ge 4\pi .Area$ and that equality holds if and only if the curve is a circle.
I conjecture that:
Let $A_1A_2...A_n$ and $B_1B_2...B_n$ be two n-polygons with the lengths $a_1, a_2,...,a_n$ and $b_1, b_2,...,b_n$ such that with $i \in n$ then exist $j \in n$ such that $a_i=b_j$. If $A_1A_2...A_n$ is a cyclic and $B_1B_2...B_n$ is not a cyclic then area of $A_1A_2...A_n$ $\ge$ area of $B_1B_2...B_n$.
I am looking for the proof of the inequality above.