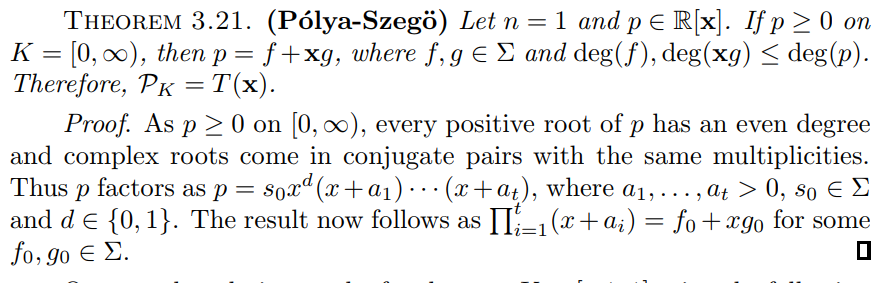12
$\begingroup$

A polynomial $p \in \mathbb{R}[x_1,\dots,x_n]$ is said to be positive on a subset $S$ of $\mathbb{R}^n$ if $p(x) > 0$ for every $x \in S$. The polynomial $p$ is called nonnegative if $p(x) \ge 0$ for every $x \in S$.

It is known that if $p \in \mathbb{R}[x]$, then $p$ is nonnegative on $\mathbb{R}$ if and only if $p = q^2 + r^2$, with $q,r \in \mathbb{R}[x]$.

I am wondering what is known about the set $\mathscr{P} := \{ p \in \mathbb{R}[x]\mid p(x) \ge 0,~\forall x\ge 0 \}$—since $f(x) = x \in \mathscr{P}$, it is clear that so this set is distinct from the globally-nonnegative case above.

Any insight/references would be greatly appreciated.

Improve this question
$\endgroup$
2
  • 2
    $\begingroup$ A minor quibble: The first sentence states a definition of positive polynomials on subsets of $\mathbb{R}^n.$ Then the entire rest is only about non-negative polynomials on subsets of $\mathbb{R}.$ $\endgroup$
    – Aaron Meyerowitz
    Commented Mar 12, 2018 at 15:27
  • $\begingroup$ @AaronMeyerowitz: edited; thank you! $\endgroup$
    – Pietro Paparella
    Commented Mar 12, 2018 at 16:28

2 Answers 2

Reset to default
17
$\begingroup$

Such a polynomial may be represented as $q^2 +xr^2 $ (proof: this is true for all irreducible divisors which have degree 1 or 2, and the set of polynomials of this kind is a multiplicative monoid by Euler identity.)

Strictly positive for $x>0$ polynomials may be represented as $q(x)/(x+1)^n$, where $q$ has non-negative coefficients.

Improve this answer
$\endgroup$
7
  • 6
    $\begingroup$ The second description of $\mathscr{P}$ is wrong, take e.g. $p(x)=(x-1)^2$. If the polynomial $p(x)(x+1)^n$ would have non-negative coefficients, then it couldn't vanish in $1$. $\endgroup$
    – Peter Mueller
    Commented Mar 12, 2018 at 13:19
  • $\begingroup$ @Fedor: do you have a reference for this or did you just come up with that? $\endgroup$
    – Pietro Paparella
    Commented Mar 12, 2018 at 16:20
  • $\begingroup$ Indeed, thank you! This is true for strictly positive on $(0,+\infty)$ polynomials. Fixed. $\endgroup$
    – Fedor Petrov
    Commented Mar 12, 2018 at 19:31
  • 1
    $\begingroup$ @PietroPaparella for which of two facts? Actually I know both for many years, but finding a suitable reference is another task. $\endgroup$
    – Fedor Petrov
    Commented Mar 12, 2018 at 20:45
  • 1
    $\begingroup$ A reference for the first fact would be great — thanks! $\endgroup$
    – Pietro Paparella
    Commented Mar 12, 2018 at 21:04
1
$\begingroup$

The result is known as the Pólya-Szegö theorem: enter image description here

Note that $\Sigma = \Sigma_n := \{ p \in \mathbb{R}[x] \mid p~\text{SOS}\}$, in which 'SOS' stands for sum of squares.

A reference for the result: https://link.springer.com/chapter/10.1007/978-0-387-09686-5_7

Improve this answer
$\endgroup$
3
  • $\begingroup$ The proof is strange. Where are factors with complex roots? $\endgroup$
    – Fedor Petrov
    Commented Mar 13, 2018 at 18:29
  • $\begingroup$ Are the nonreal roots absorbed in the polynomial $s_0$? That's the only thing I can come up with. $\endgroup$
    – Pietro Paparella
    Commented Mar 13, 2018 at 18:31
  • 1
    $\begingroup$ Ah, I see: it proves a weaker statement, not $f^2 +xg^2 $, but $f^2 +g^2 +xh^2 +xq^2 $ $\endgroup$
    – Fedor Petrov
    Commented Mar 13, 2018 at 19:00

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .