0
$\begingroup$

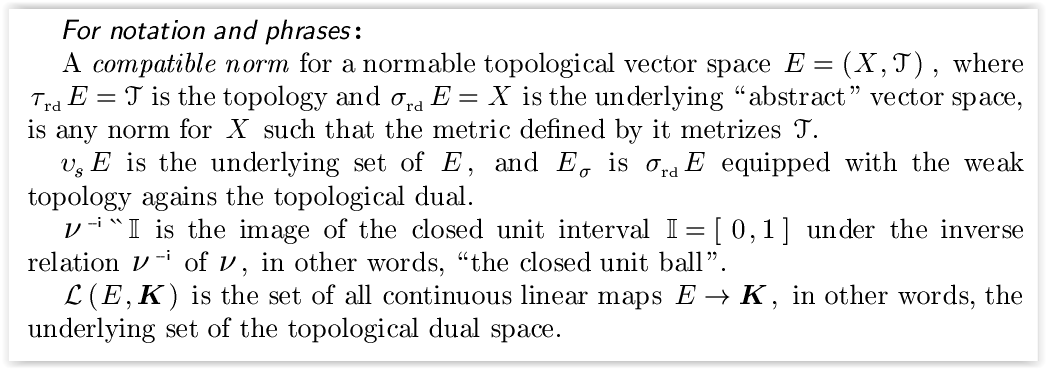
In Jarchow's Locally Convex Spaces this not being quasi-complete is asserted on page 206 referring to Corollary 11.4.4 on page 228 saying that a Banach space is reflexive if and only if its closed unit ball is weakly (sequentially/countably) compact.

I see how the compactness property implies the quasi-completeness, but I do not see how the converse (that is needed here) would be deduced.

Is the asserted non-quasi-completeness "well-known", and is there a reference containing a proof, or is Jarchow's assertion false?

Added. (25.6.2017) Having read certain places in R. E. Edwards' Functional Analysis, J. Horváth's Topological Vector Spaces and H. Jarchow's Locally Convex Spaces, I have now found (from my head) the following result:

Proposition 4 Notation

If someone can give a precise reference to an explicitly formulated previously published result with proper proof (not any vague explanations) of a result with the same content as Proposition 4 above, I am still interested to know. If it is posted as an answer, and I can easily check it via the Internet, I will accept it.

Improve this question
$\endgroup$

1 Answer 1

Reset to default
1
$\begingroup$

This is a well-known fact in Banach space theory (even locally convex space theory in a suitable form). See, for example, the classical monographs of Köthe or Schaefer. The direction you are looking for follows from the Alaoglu theorem (for the version involving compactness). For the variants, see the Eberlein-Smulian theorem, e.g., in Grothendieck's text.

Improve this answer
$\endgroup$
9
  • $\begingroup$ Could you be more specific? You mention 3 texts but no theorem or page numbers. How are you supposing Alaoglu's theorem to be applied here? $\endgroup$
    – TaQ
    Commented Jun 23, 2017 at 23:09
  • $\begingroup$ I don't have access to the texts now so can't give you page and theorem numbers. The theorem of Alaoglu (sometimes Banach-Alaoglu) states that the unit ball of the dual of a Banach space is weak star compact. In the case of reflexivity, this gives what you require. $\endgroup$
    – traun
    Commented Jun 24, 2017 at 5:54
  • 1
    $\begingroup$ Have managed to dig up Grotndieck's text "Espaces vectoriels topologiques". The result mentioned is on p. 277. By the way, if $E$ is a Banach space, then it bidual is the linear span of the bipolar of its unit ball in the algebraic dual of its dual. The latter is, by the bipolar theorem, its closure in the weak topology induced by $E'$. From this, everything follows. $\endgroup$
    – traun
    Commented Jun 24, 2017 at 6:04
  • $\begingroup$ found an article "Banach-Alaoglu theorems" by L. Erdös easily available online which would seem to have everything your heart might desire. $\endgroup$
    – traun
    Commented Jun 24, 2017 at 8:53
  • 1
    $\begingroup$ We seem to be talking at cross purposes so let me try again (I do want to help). It seems to me that the difficulty lies in the fact that you are talking about quasi-completeness, me about compactness of unit balls. But in the context of your question, these coincide. This is because the unit ball of a Banach space (resp. of a dual Banach space) is precompact for the weak (resp. for the weak star uniformity) so that they are compact for these topologies if and only they are complete for the associated uniformities. Please accept this in the spirit it is intended and not as "teaching". $\endgroup$
    – traun
    Commented Jun 25, 2017 at 15:58

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .