It is known that $$\int_ 0^{\infty}\frac {e^{-x - \frac {1} {x}}} {x} dx=2 K_0(2),$$ but now I want to get the closed form approximate result of $$\int_ 0^a\frac {e^{-x - \frac {1} {x}}} {x} dx.$$ I have searched the classic Table of Integrals, Series, and Products, but there is no pattern match this situation. Is there some approaches to the problem?
$\begingroup$
$\endgroup$
2
-
1$\begingroup$ If you want large $a$, there is an asymptotic expansion for $\int_a^\infty$ that starts $e^{-a} (1/a-2/a^2+9/(2a^3)+\cdots)$. $\endgroup$– Brendan McKayCommented Dec 4, 2016 at 3:43
-
$\begingroup$ What range of $a$ did you have in mind? $\endgroup$– Pat DevlinCommented Dec 4, 2016 at 5:47
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$

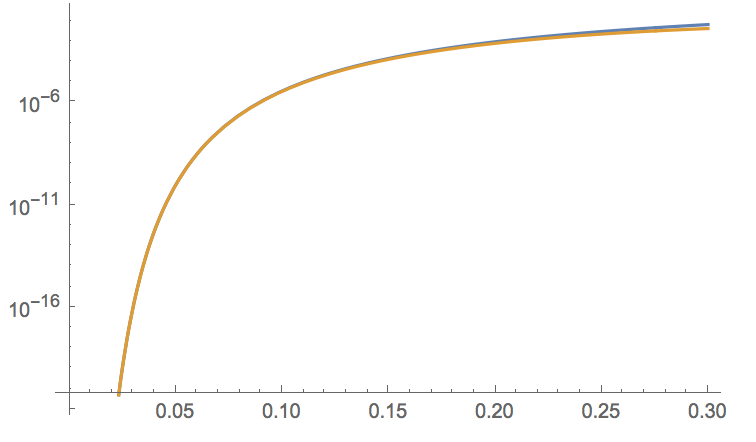
The blue curve is the desired integral $\int_ 0^{a}\frac {e^{-x - \frac {1} {x}}} {x} dx$, the orange curve is the approximate answer $2K_0(2)a^3(1+a^3)^{-1}$.
You could use this for a global approximation. In the small-$a$ region the asymptotics is $e^{-1/a}(a-2a^2)$, which is quite accurate up to about $a=0.2$, see plot below. Incidentally, the small-$a$ and large-$a$ asymptotics (see Brendan McKay's comment) are the same upon replacement of $a$ by $1/a$.