A chain of six circles associated with a conic

Question

I found this problems three years ago. But I never have been a proof. Recently I posted in math.stackexchange.com. I am looking for a solution of the following problems:

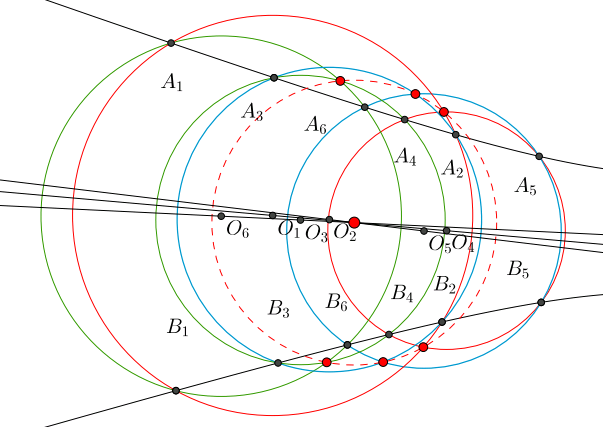
A chain of six circles associated with a conic.

Let $A_1, ..., A_6$ and $B_1, ..., B_6$ be 12 points lying on a conic, and suppose that for $i=1, ..., 5$ through $A_i, A_{i+1}, B_{i+1}, B_i$ passes a circle $(O_i)$. Then through $A_6, B_6, A_1, B_1$ as well passes a circle $(O_6)$. Let $P_1, P_4$ be intersection points of $(O_1)$ and $(O_4)$; the same for $P_2, P_5$ and $P_3, P_6$. Show that:

1. Three lines $O_1O_4$, $O_2O_5$, and $O_3O_6$ have a common point $O$.

2. Six points $P_1, ..., P_6$ lie on a circle with center in $O$.

My remark: With six points and six lines we get the Pascal theorem. With 12 points and six circles we have this problem

• I checked it by Geogebra, But I can not calculate.

• See also: Sequences of Concyclic Points on a Conic and Geogebra A chain of six circles associated with a conic

• There are two other chains of six circles

Answer 1

Since $С_1$, $C_2$, and your conic $\alpha$ pass through $A_2$ and $B_2$ we get that $A_1B_1$ and $A_3B_3$ are parallel (it calls three conic theorem http://mathworld.wolfram.com/ThreeConicsTheorem.html). Applying it several times to your construction you get that $C_6$ exists.

Intersection of $O_1O_4$, $O_2O_5$ and $O_3O_6$ follows from the Pappus theorem applied to the triple parallel lines $O_1O_2$, $O_3O_4$, and $O_5O_6$ and the triple $O_2O_3$, $O_4O_5$, and $O_6O_1$.

Upd. Regarding the second question: @zeb was almost right. We need lemma with little bit different combinatorics: $(abcd)$, $(aba_1b_1)$, $(abc_1d_1)$, $(cda_1b_1)$, $(cdc_1d_1)$ => $(a_1b_1c_1d_1)$.

Lets show that $P_1$, $P_4$, $P_3$, and $P_6$ lie on a circle. For that we note that the following quadruples circumscribed $(A_2B_2A_5B_5)$, $(A_2B_2P_1P_4)$, $(A_2B_2P_3P_6)$, $(A_5B_5P_1P_4)$, $(A_5B_5P_3P_6)$ and then apply the Lemma.

It is clear that center of this circle is $O$ because it is lie on the corresponded perpendicular bisectors of $P_iP_{i+3}$. Therefore all $P_i$ lie on the fixed circle with center at $O$.