Dao's theorem on six circumcenters associated with a cyclic hexagon

Question

This questions from Ngo Quang Duong's paper

In 2013, O. T. Dao published without proof a theorem with title Another seven circles theorem in Cut the Knot, a free site for popular expositionsof many topics in mathematics.

The calculation of barycentric coordinate for concurrence given by N. Dergiades takes more than 72 pages A4. In 09-2014, N. Dergiades gave an elegant proof of this theorem and renamed this theorem: Dao’s theorem on six circumcenters associated with a cyclic hexagon. In 10-2014, T. Cohl, a Taiwan student, gave a synthetic proof for this theorem. Two proofs were published in the Forum Geometricorum journal.

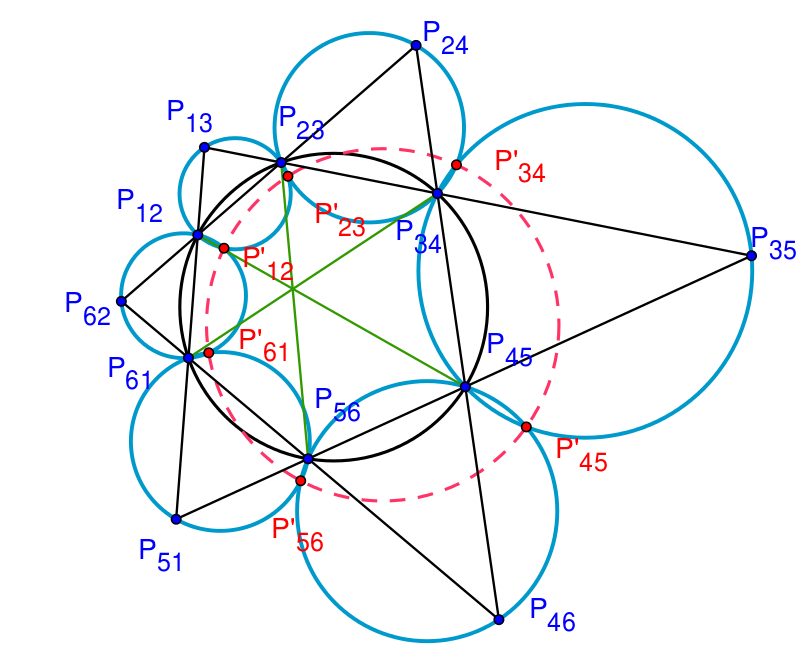
We consider the following configuration: Let $L_1, L_2, L_3, L_4, L_5, L_6$ be six lines and let $P_{ij}= L_i \cap L_j$, such that $P_{12},P_{23}, P_{34}, P_{45}, P_{56}, P_{61}$ lie on a circle. Let $(O_{ijk})$ is circle $(P_{ij}, P_{jk}, P_{ik})$ with center $O_{ijk}$. Let $(O_{ijk})$ meets $(O_{jkh})$ again at $P'_{jk}$. (We taking subscripts modulo 6.)

I am looking a solution for the problem as follows:

Problem 1 Ngo Quang Duong: Let $P_{12}P_{45}, P_{34}P_{61}, P_{56}P_{23}$ are concurrent at $P$. Then six points $P'_{12}$, $P'_{23}$, $P'_{34}$, $P'_{45}$, $P'_{56}$, $P'_{61}$ lie on a circle.

PS: I posted this topic because the problem 1 look like Miquel's Pentagram Theorem

Answer 1

• The solution of problem in our paper On an Extension of Miquel's Theorem to a Cyclic Hexagon;

• Relative configuration in here On the eight circles theorem and its dual