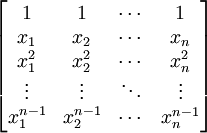
Let $x_1,x_2,\cdots,x_n\in\mathbb{R} $ or $\mathbb{C}$. By the non-degeneracy of Vandermonde matrix
the maps
$$ f: \mathbb{R}\longrightarrow\mathbb{R}^n,$$ $$ x\longmapsto (1,x,x^2,\cdots,x^{n-1}) $$ and $$ f:\mathbb{R}^2=\mathbb{C}\longrightarrow \mathbb{R}\times\mathbb{C}^{n-1}=\mathbb{R}^{2(n-1)+1}, $$ $$ z\longmapsto (1,z,z^2,\cdots,z^{n-1}) $$ satisfy the condition:
(C) for any distinct $x_1,x_2,\cdots,x_n$, their images are linearly independent.
Question: how to construct maps satisfying (C)
$$ f: \mathbb{R}^m\longrightarrow \mathbb{R}^{m(n-1)+1} $$ for $m\geq 3$?