the "Kahn-Priddy map" and "multiplicative $p$-local equivalence"

Question

The following is a part of a paper that I need to understand

I totally do not know the argument. Could you explain? Thanks.

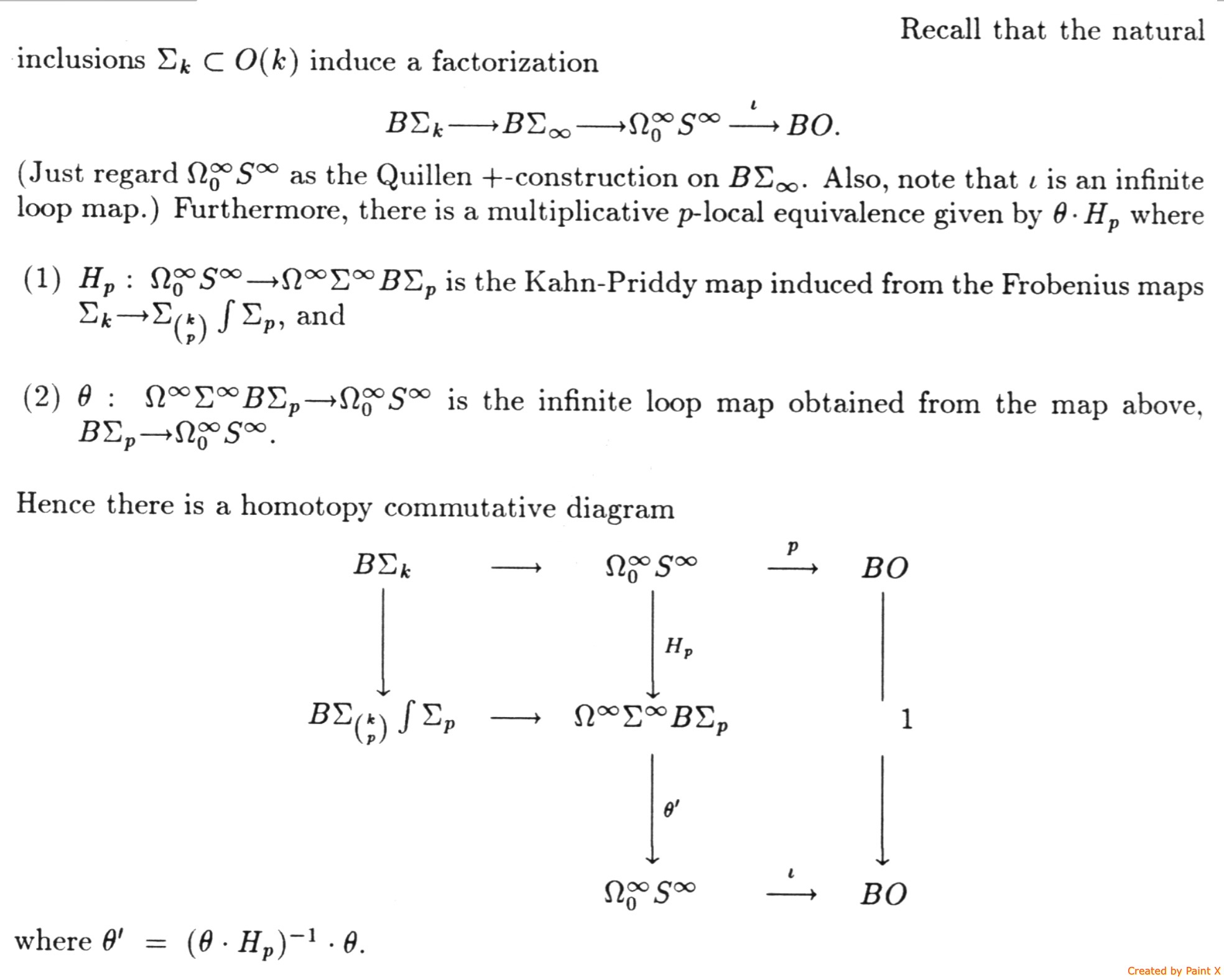
Let $\Sigma_n$ be the $n$-th symmetric group and $\Sigma_\infty$ be the direct limit. Let $\Omega^nS^n$ be the $n$-iterated loop space on the $n$-sphere and $\Omega_k^nS^n$ be the subset of $\Omega^nS^n$ consisting of maps $S^n\to S^n$ of degree $k$. Let $\Omega^\infty_kS^\infty$ be the direct limit of $\Omega^n_kS^n$ with respect to $n$. I want to find references or explanations of the following items:

(1). $\Omega^\infty_0S^\infty$ is the Quillen plus construction on $B\Sigma_\infty$.

For any prime $p$,

(2). there is a "Frobenius map": $\Sigma_k\to \Sigma_{{k}\choose{p}}\int \Sigma_p$ (What does the integral mean?) which induces a "Kahn-Priddy map" $H_p:\Omega^\infty_0S^\infty\to \Omega^\infty \Sigma^\infty B\Sigma_p$.

(3). the Quillen plus construction gives a map $i: B\Sigma_p\to \Omega^\infty_0S^\infty$. This map induces a map $\theta_p: \Omega^\infty\Sigma^\infty B\Sigma_p\to \Omega_0^\infty S^\infty$.

(4). The composition $\theta_p H_p:\Omega_0^\infty S^\infty\to \Omega_0^\infty S^\infty$ is a "multiplicative $p$-local equivalence" (what means "multiplicative $p$-local equivalence"?).

Could you give a help for references or explanations? What do the above items mean?

Answer 1

For (1) Adams's book ``Infinite loop spaces'' contains good explanations. Also, a paper by Barratt and Priddy `On the Homology of Non-Connected Monoids and Their Associated Groups', I think is a good reference.

For (2), the maps you consider are the Stable James-Hopf invariants, and not Kahn-Priddy as far as I know, which arise from Snaith splitting. A good reference is Kuhn's paper on `The geometry of the James-Hopf maps'.

(3) and (4) are related to the extension of the Kahn-Priddy theorem (related to the case p=2 of Whitehead conjecture) to odd primes, and I think you can find proofs in Kuhn and Priddy's paper `The transfer and Whitehead's conjecture'

as well as more, what I call, `computation-free' work, in his other paper

`EXTENDED POWERS OF SPECTRA AND A GENERALIZED KAHN-PRIDDY THEOREM'.

Added, I think for the getting a $p$-local epimorphism $B\Sigma_p$ is not enough, and you need another $D_2$-factor for that. You may look at, Theorem 1.1(2) of the second paper for this extra factor.

EDIT: In general, for a path connected space $X$ the stable James-Hopf invariants are not multiplicative which you can see by homology computations; look at Kuhn's paper `The Homology of the James-Hopf maps'. But, in the cases such as $X=S^0$ you may get a map which deloops once, but probably not an infinite loop map, even not an $\Omega^2$ map in the case of $Q_0S^0\to Q\mathbb{R}P$; again look at Kuhn's paper on the homology, Remark 2.15. In that paper, the author shows that there is a choice $Q_0S^0\to Q\mathbb{R}P$ which deloops once. You probably can do the same of other $B\Sigma_r$ factors.

You may look at papers of Barratt and Eccles, as well as Kuhn, to see how one may get James-Hopf maps $QX\to QD_rX$ when $X$ is not path connected.