3
$\begingroup$

2-bridge links $L(p/q)$ are described by the continuous fraction expansion $\frac{p}{q}=\left[a_1,a_2,\ldots,a_n\right]$, where the $a_i$ are the numbers of twists in the boxes below:

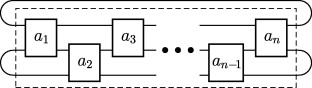
Looking at some knot table I've got the impression that a 2-bridge knot has vanishing Chern-Simons invariant if and only if the continued fraction expansion is symmetric in the sense that $$a_1=a_n,a_2=a_{n-1},\ldots.$$ For example $$5/2=\left[2,2\right], 13/5=\left[2,1,1,2\right], 17/4=\left[4,4\right], 25/7=\left[3,1,1,3\right]$$ $$29/12=\left[2,2,2,2\right], 41/9=\left[4,1,1,4\right], \ldots, 149/44=\left[3,2,1,1,2,3\right],\ldots$$ all have vanishing Chern-Simons invariant.

Question: is this true, is it known and what is a citeable reference?

(I would already be happy with the "if"-part, i.e., that all these knots satisfy $CS=0$.)

Improve this question
$\endgroup$
1
  • $\begingroup$ I'm not sure how relevant this is, but the symmetry property has something to do with having a reversible branched double cover. $\endgroup$
    – Marco Golla
    Commented Mar 20, 2015 at 12:42

1 Answer 1

Reset to default
2
$\begingroup$

EDIT: the original answer had a typo the minus sign was missing from: $q^{\pm 1}=-q \mod 2p$

Here is an argument for the forward direction:

Each knot in the list above appears to be amphichiral (i.e. equivalent to it's mirror image under ambient isotopy). If we parametrize 2-bridge knots by $(p,q)$ where $p,q$ both odd, a result of Schubert (also see Theorem 12.6 of Burde and Zieschang's "Knots") states that a 2-bridge knot $(p,q)$ is amphichiral if and only if $q^{\pm 1}=-q \mod 2p$.

Since the Chern-Simons invariant is sensitive to orientation, it vanishes for an amphichiral 2-bridge knot complement (more generally any amphichiral knot complement).

Improve this answer
$\endgroup$
5
  • $\begingroup$ Thank you very much. The equivalence of the above described symmetry to $q=\pm q^{-1}\ mod\ p$ appears to be a classical fact. $\endgroup$
    – ThiKu
    Commented Mar 25, 2015 at 1:36
  • $\begingroup$ One direction is due to Serret sites.mathdoc.fr/JMPA/PDF/JMPA_1848_1_13_A2_0.pdf . I didn't find the Original source for the other direction, but iT is mentioned in arxiv.org/pdf/1406.7571v2.pdf $\endgroup$
    – ThiKu
    Commented Mar 25, 2015 at 1:39
  • $\begingroup$ I can not edit your answer, but obviously $2p$ has to be $p$ in the equation. $\endgroup$
    – ThiKu
    Commented Mar 25, 2015 at 1:41
  • $\begingroup$ Actually, since we are interested in oriented 2-bridge knots we do want it to be $2p$. Equivalence of unoriented 2-bridge knots only requires congruence mod $p$. $\endgroup$
    – Neil Hoffman
    Commented Mar 25, 2015 at 2:12
  • $\begingroup$ Ah, I see. So it works just for knots, not for links. $\endgroup$
    – ThiKu
    Commented Mar 25, 2015 at 3:03

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .