Let $M$ be a oriented compact $3$-manifold, closed or with boundary. For any incompressible surface $F$, define a function $i_F$ on the set of homotopy classes of closed curves in $M$ by $$i_F (\alpha) = \alpha \cap F $$ the geometric intersection number of $\alpha$ with $F$.
Is it true that two incompressible, $\partial$-incompressible surfaces $F$ and $F'$ are isotopic if $i_F =i_{F'}$?
Yes, this is true (with an appropriate definition of $i_F(\alpha)$).
An incompressible surface gives rise to an action of $\pi_1(M)$ on a tree (see for example Chapter 1 of Shalen's notes). For a homotopy class of loops $\alpha$, define $i_F(\alpha)=\|\alpha\|$ to be the translation length of $\alpha$ acting on this tree (well-defined up to conjugacy). In fact, $\alpha$ can be homotoped so that the geometric intersection number is $\|\alpha\|$. Then this defines a length function on conjugacy classes in $\pi_1(M)$, satisfying the Culler-Morgan axioms (1.11):
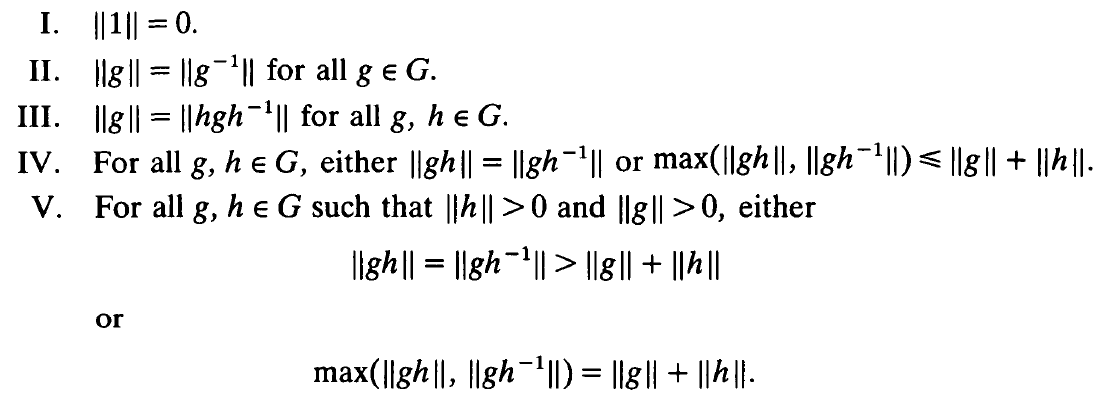
Culler and Morgan show that their axioms uniquely characterize a minimal action of a group on a tree, if the action is semi-simple. It's not hard to see that if the surface is not a fiber or semi-fiber, that the action is semi-simple, essentially from the cocompactness of the action. In the fiber or semi-fiber case, the intersection number determines a homomorphism to $\mathbb{Z}$ or $D_\infty$, whose kernel is finitely generated and determines the surface group. In the semifibered case, I don't think $I_F(\alpha)$ distinguishes the two non-orientable surfaces. However, your assumption of incompressible surface usually means the surface is orientable, and thus this case won't appear.
Thus, suppose two surfaces induce the same length function. Then they induce the same action on a tree, and thus the edge stabilizer will be the same, implying that the surfaces are homotopic. But homotopic incompressible surfaces are isotopic, by a result of Waldhausen (Corollary 5.5; Waldhausen assumed that the manifold is irreducible, but I believe this was only to avoid the pitfall of a potential counterexample to the Poincaré conjecture as a connect summand).
