Let $(p,q)$ be a pair of coprime (positive) integers. Consider the torus knot $T_{p,q}$. What is the minimal genus of an (embedded) oriented Seifert surface for this knot?
It is not had to convince oneself that in the simplest case $p =2$, there is a Seifert surface of genus $(q-1)/2$. I do not know whether that is optimal.
One of the "classical" proofs involves the Alexander polynomial.
The knot group $\pi_1(S^3\setminus T_{p,q})$ has a presentation $\langle x,y \mid x^p = y^q\rangle$, and using Fox calculus one can quickly compute the Alexander polynomial to be $$\Delta_{T_{p,q}}(t) = \frac{(t^{pq}-1)(t-1)}{(t^p-1)(t^q-1)}.$$ The degree of the Alexander polynomial gives a bound on the genus, so we get $2g(T_{p,q})\ge\deg\Delta_{T_{p,q}} = (p-1)(q-1)$. Since this lower bound agrees with the upper bound given by Seifert's algorithm, you're done.
Here's another route: the standard picture of the torus knot is a positive braid, so applying Seifert's algorithm gives a minimal genus surface. There are (say) $p$ seifert circles and $q(p-1)$ crossings, so rearranging the Euler characteristic gives the genus as $(p-1)(q-1)/2$.
Another approach I got from the paper Fibered Links in $S^3$, available at https://doi.org/10.1016/j.exmath.2016.06.006.
Torus knots are algebraic, so they are fibered. It is known that the fiber surface of a fibered knot is the minimal genus Seifert surface. Example 3.2 of the aforementioned paper presents a fiber surface, hence the min genus Seifert surface, for the torus knot $T(p,q)$ as a blackboard framed embedding of the complete bipartite graph $K_{p,q}$ under a certain embedding in $S^3$. Here it is in the case $(p,q)=(3,4)$, Figure 6 from the paper.
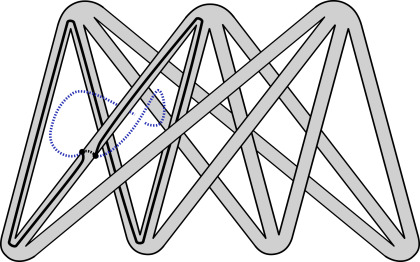
The Euler characteristic of such a surface is just $(p+q)-(pq)$. On the other hand, the surface has 1 boundary component so its Euler characteristic is $1-2g$ where $g$ is the genus. Thus $T(p,q)$ has genus $(p-1)(q-1)/2$.
