9
$\begingroup$

While thinking about this interesting question asked by Dylan Thurston, it occured to me that in every case that I know, the closer a knot diagram is to being alternating, the better its properties. For example, by the Tait Conjecture, a reduced diagram of an alternating link has the smallest number of crossings.

We can define the distance of a diagram from being alternating by a lexicographical ordering according to lengths of sequences of consecutive over/undercrossings. Other measures on non-alternating-ness are possible, but I'd like to ask about this "most naive" one.

Question:
  • Is the crossing number of a knot type always realized by a `most alternating' reduced diagram according to the above metric? (This would hugely generalize the Tait Conjecture so surely the answer is no, but where can I find a counterexample?)
  • And its stick number?
  • And its prime decomposition? (related to this question.)
  • And its representation of a braid closure/ plat closure?

Note that I'm not asking about diagrams in which various symmetries are visible, because then the statement would be false. For example, the following diagram for the figure eight knot illustrates its amphicheirality, but is not alternating:

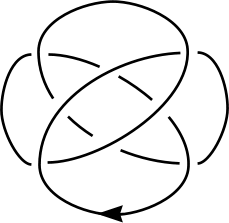
Amphicheiral Figure Eight Knot

Essentially, the question is whether the more alternating a diagram is, the better it is as a diagram. Does this basically seem to be the case?

To reverse the question and to make it easier to answer precisely, an equivalent question is:

Is there an example of "less alternating" reduced diagram with a better property as a diagram (excluding visualization of symmetries) than any "more alternating" diagram of the same knot?

Please feel free to replace the word "knot" by "link", "virtual knot", "virtual link", or even "virtual tangle" in the above question.

Improve this question
$\endgroup$
2
  • $\begingroup$ @User43408: If you are indeed Ken Perko, welcome to MO! Your suggested edit doesn't seem to fit completely with this question. You could ask that as a separate question if you see fit. $\endgroup$
    – Lucia
    Commented Dec 2, 2014 at 0:03
  • 1
    $\begingroup$ This is the comment left by user43408 in the form of an edit: So are the 29 Perko knots (through 12 crossings) somehow the least alternating, being the only examples (among thousands in this range) with minimal crossing projections exhibiting more than one writhe? // I can edit, but lack sufficient reputation to comment. How silly is that? --Ken Perko $\endgroup$
    – Yoav Kallus
    Commented Dec 2, 2014 at 0:15

2 Answers 2

Reset to default
8
$\begingroup$

This is an answer to your second question. As noticed by Steve Bleiler in "A note on Unknotting number", the minimal crossing diagram for the two bridge knot 10_8 ((29,5) in two bridge notation) has unknotting number 3, while a 14 crossing diagram has unknotting number 2, which is the unknotting number of the knot.

EDIT: In looking at the literature, this observation is also attributed to Yasutaka Nakanishi, "Unknotting numbers and knot diagrams with the minimum crossings," Math. Sem. Notes Kobe Univ., 11(2):257–258, 1983.

Improve this answer
$\endgroup$
1
  • 1
    $\begingroup$ Nice answer! I hadn't known this result. My quibble is that unknotting number (hence the result) is an much about the crossing change move (as opposed to some other local move, e.g. the delta move or the pass move) as it is about the diagram- one might not necessarily expect "more alternating" to be "better" with respect to a specific (family of) local move(s), so this is an excellent point. $\endgroup$
    – Daniel Moskovich
    Commented Oct 27, 2014 at 8:29
1
$\begingroup$

Braid closure representations have a computational and notational advantage, even when they aren't alternating. Diagrams with the minimal bridge number are also usually not alternating, but make the fundamental group easier to compute.

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .