A curious puzzle for which I would appreciate an explanation.
For $x$ and $y$ both uniformly and independently distributed in $[0,1]$, the value of $\lfloor 1/(x y) \rfloor$ has a bias toward odd numbers. Here are $10$ random trials: $$51, 34, 1, 239, 9, 4, 2, 1, 1, 1 $$ with $7$ odd numbers. Here are $10^6$ trials, placed into even and odd bins:
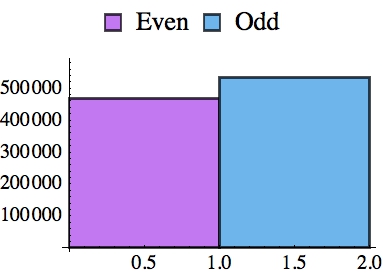
About 53% of the reciprocals are odd. If I use the ceiling function instead of the floor, the bias reverses, with approximately 47% odd. And finally, if I round to the nearest integer instead, then about 48% are odd.
None of these biases appear to be statistical or numerical artifacts (in particular, it seems that the 47% and 48% are numerically distinguishable), although I encourage you to check me on this.
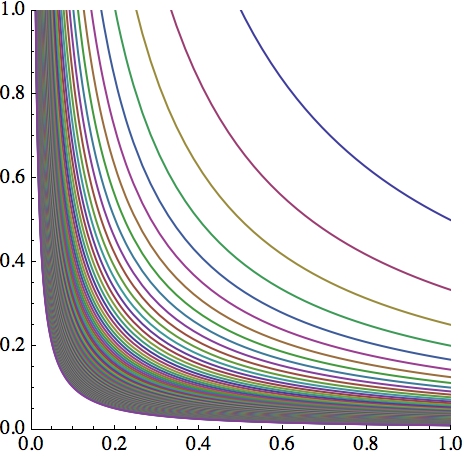
Update. To supplement Noam Elkies' answer, a plot of $x y = 1/n$ for $n=2,\ldots,100$: