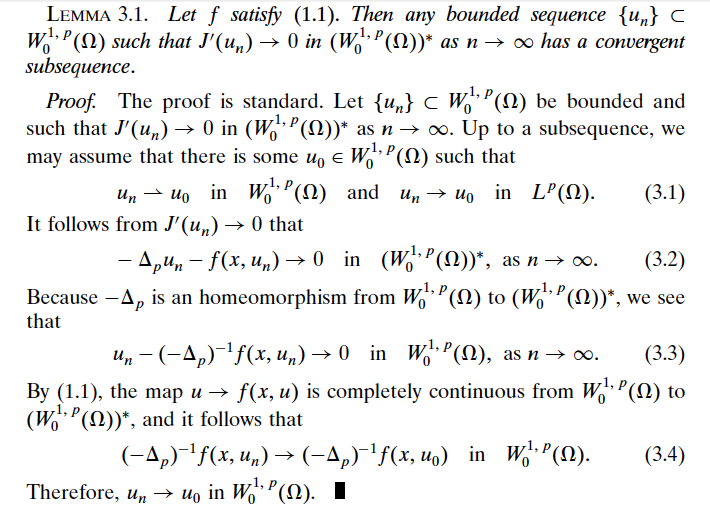
This is really about what the Frechet derivative $J'(u)$ is. For given $u\in W^{1,p}_0$ it is by definition the unique element in the dual space $(W^{1,p}_0)^*=W^{-1,p'}$ such that
$$
J(u+h)=J(u)+\left<J'(u),h\right>_{(W^{1,p}_0)^*,W^{1,p}_0}+o(|h|_{W^{1,p}_0}).
$$
Here you can easily compute for fixed $u\in W^{1,p}_0$ and arbitrary "test-function" (or first order perturbation)
$$
\frac{1}{p}\int_{\Omega}|\nabla(u+h)|^p=\frac{1}{p}\int_{\Omega}|\nabla u|^p+\int_{\Omega}|\nabla u|^{p-2}\nabla u\cdot\nabla h+o(|\nabla h|_{L^p})
$$
and
$$
\int_{\Omega}F(x,u+h)\,dx=\int_{\Omega}F(x,u)\,dx+\int_{\Omega}\partial_u F(x,u)h\,dx+o(| h|_{L^p})
$$
hence by definition of $f=\partial_u F$
$$
\left<J'(u),h\right>_{(W^{1,p}_0)^*,W^{1,p}_0}=\int_\Omega|\nabla u|^{p-2}\nabla u\cdot\nabla h \,dx-\int_{\Omega}f(x,u) h\,dx.
$$
The first term in the right-hand side is nothing but the weak formulation of the (negative) $p$-Laplacian, i-e assuming that everything is smooth enough and integrating by parts
$$
\int_\Omega|\nabla u|^{p-2}\nabla u\cdot\nabla h=-\int_\Omega\operatorname{div}(|\nabla u|^{p-2}\nabla u) h=-\int_{\Omega}(\Delta_p u)h.
$$
This means that $J'(u)=-\Delta_p u-f(x,u)$ as elements of $(W^{1,p}_0)^*$, which is exactly your statement.