In Rivasseau's and Wang's How to Resum Feynman Graphs, on page 11 they illustrate the intermediate field method for the $\phi^4$ interaction and represent Feynman graphs as ribbon graphs. I had to read up about ribbon graphs as I've never heard of them before and still don't fully understand their use for the intermediate field method. For the $\phi^4$ interaction, we have:
In that case each vertex has exactly four half-lines. There are exactly three ways to pair these half-lines into two pairs. Hence each fully labeled (vacuum) graph of order $n$ (with labels on vertices and half-lines), which has $2n$ lines can be decomposed exactly into $3^n$ labeled graphs $G'$ with degree $3$ and two different types of lines
- the $2n$ old ordinary lines
- $n$ new dotted lines which indicate the pairing chosen at each vertex (see Figure 5).
The extension illustrated in Figure 5 looks as follows:
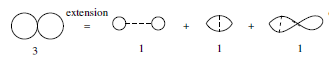
I'm not sure I correctly understand the way to create such "3-body extensions". I understand it in that way that we "split up" the given vertex into two vertices and add an edge between them. Then, each vertex has two additional half-edges and there are three ways to pair them together. Joining the upper half-edges together, we get the second term on the RHS and joining the two half-edges on one side together, we will get the first term on the RHS (the dashed line would be the new edge between the two vertices). The only case left is the one of joining the upper left and the lower right (and the upper right and lower left) half-edge together. However, when drawing that specific case, I don't see any way to stretch or rotate it such that the third term on the RHS results.
Am I misunderstanding the way 3-body extensions are intended to get or am I just missing a way to stretch the edges to get the third term on the RHS? Also, where exactly do we need the cyclic ordering characterising ribbon graphs? And why are there two dashed lines in the third term on the RHS?
