0
$\begingroup$

Would like to build once more on this question.

Take $s=\sigma + ti, s \in \mathbb{C}, 0<\Re(\sigma)<1$.

Let's assume it is proven that:

$$\zeta(1-s) - \zeta(s)$$

has all its zeros on the critical line when:

$$\chi(s)=2^s \pi^{s-1} \sin(\frac{\pi s}{2}) \phantom. \Gamma(1-s) = \pm 1$$

The other zeros are the $\rho$'s and they simply emerge when $0 - 0 = 0$, and obviously no further information can be derived about the validity of the RH. Searching for ways around this (unsuccesfully), I stumbled on another question.

From:

$$\zeta_H(s,a) = \zeta_H(s,a+1) + a^{-s}$$

it follows that:

$$\zeta(1-s) - \zeta(s) = \zeta_H(1-s,2) - \zeta_H(s,2)$$

And the latter term can be factored into:

$$ \left( \sqrt{(\zeta_H(1-s,2)} - \sqrt {\zeta_H(s,2)} \right) \left( \sqrt{(\zeta_H(1-s,2)} + \sqrt {\zeta_H(s,2)} \right)$$

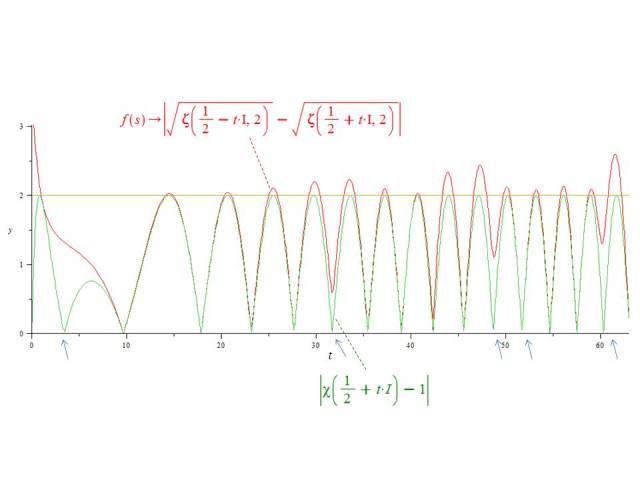
A plot of both factors for $\sigma = \frac12$ revealed:

  • that all $\rho$ are produced as zeros of the second factor only.

  • the first factor shows discontinuities at the $\rho$'s, but also for a few additional values.

  • the discontinuities vanish when taking the absolute value (this only works when $\sigma = \frac12$).

  • this absolute function has zeros, but all $\rho$'s will now be residing on the line $y=2$.

Assume: $f(t) = |\left( \sqrt{(\zeta_H(\frac12-t i,2)} - \sqrt {\zeta_H(\frac12+t i,2)} \right)|$.

Note that $f(t)$ shares all its zeros with $|\chi(\frac12+t i)-1|$, but not the other way around. There appear to be 'missing zeros' in $f(t)$ that emerge at random spots. Given the similarities and overlaps between the two plots, I wondered if $f(t)$ might be a 'distorted' version of $|\chi(\frac12+t i)-1|$. A distortion potentially caused by randomly missing zeros in a "Hadamard"-type infinite product of the zeros of $|\chi(\frac12+t i)-1|$?

Question:

The function $\chi(s)-1$ is a meromorphic function, could the Weierstrass/Hadamard factorisation theorem be used to express $\chi(s)-1$ as an infinite product of its zeros?

Improve this question
$\endgroup$
6
  • $\begingroup$ $\chi(s)$ is a meromorphic function. I do not understand the assertion that $|\chi(s)-1|$ is entire. The modulus of a non constant meromorphic function is not entire. If you think only on the critical line $|\chi(0.5+it)-1|= 2 |\sin\vartheta(t)|$ has real zeros, and the modulus is not differentiable as a function of a real variable. $\endgroup$
    – juan
    Commented Sep 2, 2012 at 7:48
  • $\begingroup$ @Juan. You are right and I have corrected it in my question. Note that I do not necessarily need the modulus, since I am predominantly interested in the overlapping zeros, however that still doesn't make $\chi(s)-1$ entire of course. Do you think it is possible to find such an infinite product (similar to the Hadamard product for $\zeta(s)$)? $\endgroup$
    – Agno
    Commented Sep 2, 2012 at 12:29
  • $\begingroup$ @Agno Observe that $\zeta(s)=\chi(s)\zeta(1-s)$ gives $\chi(\frac12+it)=\zeta(\frac12+it)/\zeta(\frac12-it)$. Then your function $f(t)=|\sqrt{\zeta(\frac12-it)}-\sqrt{\zeta(\frac12+it)}|=|\sqrt{\zeta(\frac12-it)}|\cdot|1-\sqrt{\chi(\frac12+it)}|$. (If you take adequate roots). I think that the graph is not correct $f(t)$ vanish at the Gram point t=31.717979954764 or you are taking differents roots of $\zeta(\frac12+it)=\zeta(\frac12-it)=$ that at this point is a negative real number. At this point $\chi(t)=1$. $\endgroup$
    – juan
    Commented Sep 2, 2012 at 16:37
  • $\begingroup$ @Juan. I now suddenly see that I have put you on the wrong foot. $f(t)$ is a difference between the roots of the Hurwitz zetas $(a=2)$ and not of the regular ones $(a=1)$. The correct formula is listed in red in the picture, but in the text I forgot to put the roots in the formula. Have corrected it now. $\endgroup$
    – Agno
    Commented Sep 2, 2012 at 17:09
  • $\begingroup$ @Agno The real points $t$ where $\chi(t)=1$ are the Gram points. At a Gram point $\zeta(1/2+i g_n,2)=\zeta(1/2-i g_n,2)$ your function $f(t)$ would be $0$ at $g_n$ unless $\zeta(1/2+i g_n,2)<0$. This is equivalent to $\zeta(1/2+i g_n)<1$. This happen for $g_3 = 31.71$, $g_8=48.71$, $g_{12}=60.35$, $g_{18}=76.17$, $g_{23}=88.38$, $g_{26}=95.41$ $\dots$ $\endgroup$
    – juan
    Commented Sep 2, 2012 at 17:42

0

Reset to default

You must log in to answer this question.

Browse other questions tagged .