$\nabla \times (F\times \mathbf v)=g$, $\operatorname{div}(\mathbf v)=0$

Question

I want to solve the equation: $$ \begin{cases} \nabla \times (F\times\mathbf v)=g, \\ \operatorname{div}(\mathbf v)=0, \end{cases}\label{1}\tag{1} $$ where $F$ and $g$ are given vector fields.
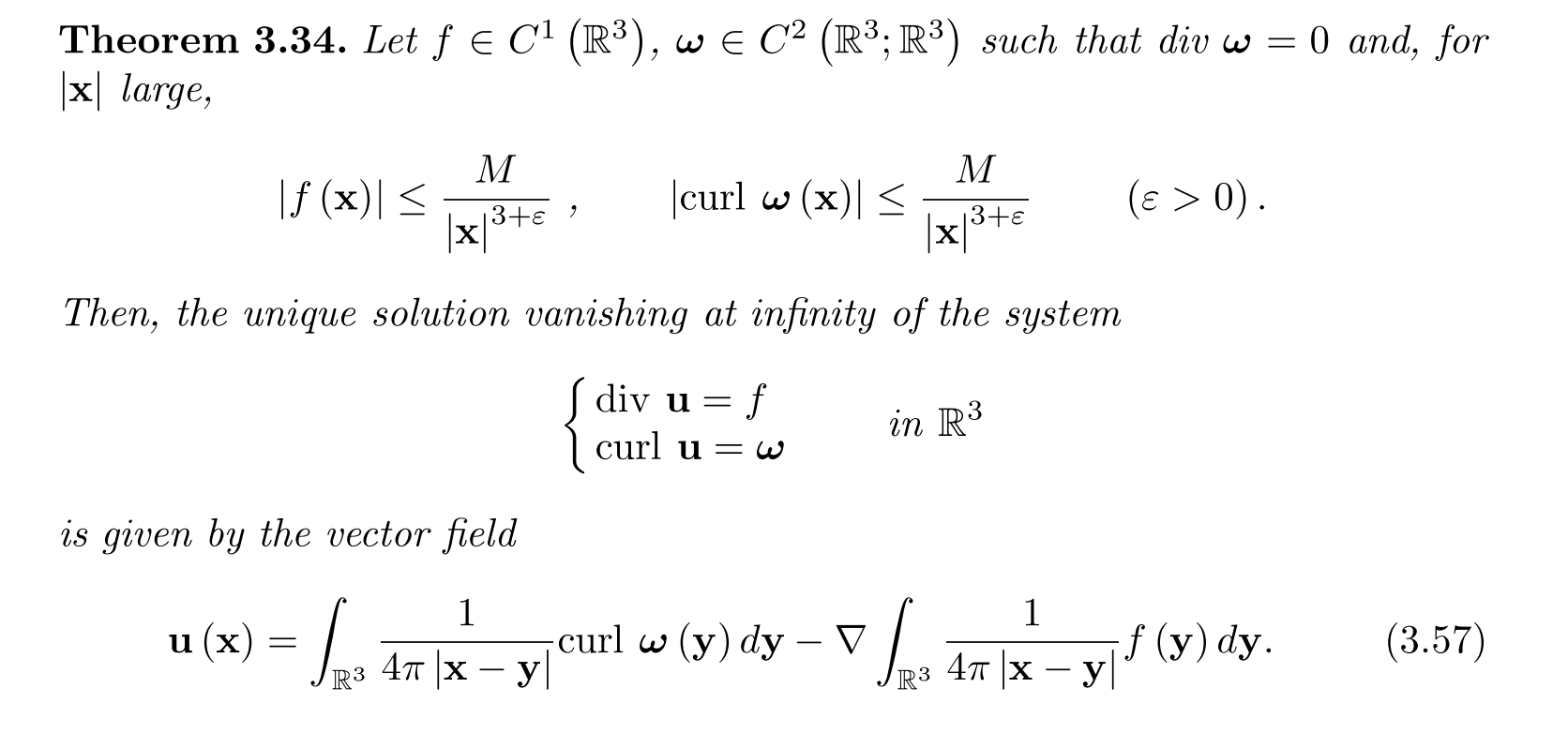
The theorem shown in the attached picture (taken from Sandro Salsa, Partial Differential Equations in Action From Modelling to Theory, Third Edition, Chapter 3.6.3, MR3497072, Zbl 1383.35003) states that we can solve it using Helmholtz decomposition and find an explicit solution.
I'm wondering about the condition on the vector $F$ so that equation \eqref{1} admits a unique solution: are there any references to this equation?

Theorem 3.34. Let $f \in C^1(\mathbb R^3)$, $\boldsymbol\omega \in C^2(\mathbb R^3; \mathbb R^3)$ such that $\operatorname{div} \boldsymbol\omega = 0$ and, for $\lvert\mathbf x\rvert$ large, $$ \lvert f(\mathbf x)\rvert \le \frac M{\lvert\mathbf x\rvert^{3 + \varepsilon}},\quad \lvert\operatorname{curl} \boldsymbol\omega(\mathbf x)\rvert \le \frac M{\lvert\mathbf x\rvert^{3 + \varepsilon}} \quad (\varepsilon > 0). $$ Then, the unique solution vanishing at infinity of the system $$ \begin{cases} \operatorname{div} \mathbf u = f \\ \operatorname{curl} \mathbf u = \boldsymbol\omega \end{cases}\quad\text{in $\mathbb R^3$} $$ is given by the vector field $$ \mathbf u(\mathbf x) = \int_{\mathbb R^3} \frac1{4\pi\lvert\mathbf x - \mathbf y\rvert}\operatorname{curl} \boldsymbol\omega(\mathbf y)\,d\mathbf y - \nabla\int_{\mathbb R^3} \frac1{4\pi\lvert\mathbf x - \mathbf \rvert}f(\mathbf y)\,d\mathbf y.\tag{3.57}\label{481778_3.57} $$

Answer 1

Solutions are never unique. The simplest case to see is when $F$ has divergence zero. Then you can add any constant multiple of $F$ to $\mathbf{v}$ to get another solution.

But in any region where $\operatorname{div}F\ne0$ there are functions $\phi$ such that $\operatorname{div}(\phi F) =0$, and there $\mathbf{v}+\phi F$ is another solution.