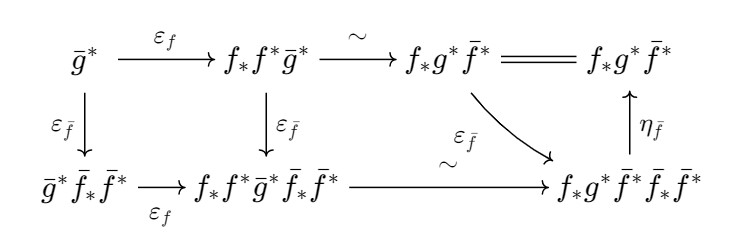2
$\begingroup$

Given a (not necessarily Cartesian) square of spaces $$\require{AMScd}\begin{CD} X @>g>> \overline{X} \\ @VVfV @VV\overline{f}V \\ Y @>\overline{g}>> \overline{Y} \end{CD}$$ does the following $$\require{AMScd} \begin{CD} \overline{g}^* @>\epsilon_f>> f_*f^*\overline{g}^* @= f_*g^*\overline{f}^* \\ @V\epsilon_{\overline{f}}VV @. @A\eta_{\overline{f}}AA \\ \overline{g}^*\overline{f}_*\overline{f}^* @>\epsilon_f>> f_*f^* \overline{g}^*\overline{f}_*\overline{f}^* @= f_*g^*\overline{f}^*\overline{f}_*\overline{f}^* \end{CD} $$ commute?

By space I mean either scheme/stack/prestack and the second diagram occurs in $\text{QCoh}(-)$, or a more restrictive class of spaces like Artin stacks and the diagram occurs in the category of $\ell$-adic sheaves, or some sheaf theory with the six functors.

The right-up composition $\overline{g}^*\overline{f}^*\to f_*g^*$ is called the Beck-Chevalley composition, and a version of this question appears in 4.1.1 of "Ambidexterity" by Haine, but it has additional assumptions of "ambidexterity" on the maps, which one might hope could be dropped for the above question.

Improve this question
$\endgroup$

1 Answer 1

Reset to default
5
$\begingroup$

This is true in complete generality. The outer rectangle in the diagram below is the diagram in question. I have duplicated $f_*g^*\bar{f}^*$ and added two more $\varepsilon_{\bar{f}}$. The left and middle square commute because everything is natural. The right triangle commutes because of the zigzag identity for the $\bar{f}_* \dashv \bar{f}^*$ adjunction.

1

Improve this answer
$\endgroup$
0

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .