A plane continuum is a bounded, closed and connected subset of the plane.
A bounding box $B$ for a plane continuum $C$ is
a rectangle $B=[a,b]\times[c,d]$ (including sides and interior)
such that $C$ is contained in $B$ and each side of $B$ intersects $C$.
(Here $a\le b$ and $c\le d$ with possible equalities in degenerate cases.)
In other words $B$ is the smallest rectangle with vertical and horizontal sides
that contains $C$.
If $J$ is a line segment with slope $1$ or $-1$ then clearly the bounding box for $J$ is a square. Every subcontunuum of $J$ is also a line segment with slope $1$ or $-1$, so the bounding box for every subcontinuum of $J$ must be a square. (We will consider a singleton to also be a degenerate line segment of length zero and slope $1$ or $-1$; its bounding box is the singleton itself, and it is a square.)
Definition.
A plane continuum $C$ will be called hereditarily square-boxed
if the bounding box for every subcontinuum of $C$ is a square. For short,
I will use the abbreviation hsb for hereditarily square-boxed.
Question.
Is there is a plane continuum $C$ that is hereditarily square-boxed
(hsb), but that is not a line segment with slope $1$ or $-1$ (and not a singleton either)?
Motivation.
Kevin Johnson (https://mathoverflow.net/users/31069/kevin-johnson),
asked the following question:
URL (version: 2013-01-31):
Does every connected set that is not a line segment cross some dyadic square?
The statement of his question is short enough (though it has remained unanswered for more than 10 years now) so I hope it is ok to include it in its entirety here:
Question (Kevin Johnson).
A dyadic square is a subset of $R^2$ of the form $x + 2^{-n} [0,1]^2$
with $x \in 2^{-m} Z^2$, for integers $m,n \geq 0$.
We say that a set $A$ crosses a square $S$ if there exists a connected subset
of $A \cap S$ which intersects two opposite sides of the square $S$.
Clearly, the 45 degree line $\{ (\pi + t, t) : t \in R \}$ does not cross any dyadic square.
Does every non-trivial closed, connected set that is not a line segment cross some dyadic square?
(End of Kevin Johnson's Question.)
It is easily seen that if $C$ is a plane continuum whose bounding box $B$ is not
a square then $C$ does cross some dyadic square. (I may include a sketch at the end.)
Also, if $C$ contains a continuum $K$ whose bounding box is not a square,
then $K$ (and therefore $C$ too) crosses
some dyadic square.
(I tend to think of rational squares instead - having
horizontal and vertical sides, and all corner points having rational coordinates - but these two variations of the question are similar.)
So, if a plane continuum $C$ were to not cross any dyadic square, then $C$ must be hsb. Therefore the motivation of my question above (and I wonder if it is indeed mine, or perhaps there is already something like this in the literature).
I could answer my own question in the special case when the continuum $C$ is the graph of some function (and in this case the function would necessarily be defined on some closed interval and be continuous, though for convenience I will just throw this into the assumptions). (Edit May 29, 2023. I believe I extended my answer for the case when the continuum is path-connected, may insert a sketch some time later.)
Result.
Suppose that $G$ is the graph of some continuous real-valued
function defined on a closed interval, and $G$ is hsb.
Then $G$ must be a line segment with
slope $1$ or $-1$.
I will sketch a proof below (using a picture) but first a couple of related questions.
Question.
Suppose $K$ is an arc in the plane that is hsb.
Must $K$ be a line segment with slope $1$ or $ -1$ ?
(It feels like this case might be similar enough to the case of the graph of a continuous function, but I didn't try to come up with a proof, so I do not know.)
(Edit. May 29, 2023. I did come up with a proof, yet to write it though. Done, May 31, 2023.)
Question.
Let $C$ be any plane continuum that is hsb.
Must $C$ be a line segment with slope $1$ or $-1$ ?
(I hope one could prove this, that is, I wish that
this would be a correct result. But the case for a general continuum (could be hereditarily indecomposable) could be quite different than the case for the graph of a continuous function, so I don't know how the proof might go, and if this indeed would be a correct result. If it were, then it would also answer Kevin Johnson's question
about crossing dyadic squares.)
I have no reason to believe that my proof for the graph-of-a-function case would easily generalize for arbitrary plane continua, so I don't know how difficult might the last question be.
Let me try write a proof for the graph-of-a-function case (stated without loss of generality with some extra assumptions, for convenience).
Result.
Let $f$ be a continuous function from the
interval $[0,1]$ into the reals, and for
convenience assume that $f(0) = 0$.
Let $G = \{ (x,f(x)) : x \in [0,1] \}$ be its graph.
If $G$ is hsb, then $G$ must be a line segment
with slope $1$ or $-1$.
Lemma.
With the above assumptions, there
cannot be $x,y \in [0,1]$ such that
$| f(x) - f(y) | > | x - y |$ (strict inequality).
Proof.
If there were such $x,y$ (say with $x<y$)
then the graph of the restriction of $f$ to $[x,y]$
would be "taller" than "wider", that is,
the height of its bounding box would be
longer than its width, a contradiction,
since the bounding box must be a square.
(The height would be at least $| f(x) - f(y) |$
and the width exactly $| x - y | = y - x$ . )
Corollary.
The function $f$ must be Lipschitz $1$, that is,
given any $x,y \in [0,1]$ we must have that
$| f(x) - f(y) | \le | x - y |$ .
Lemma.
Given $f$ is Lipschitz $1$, if $f(1) = 1$
(and $f(0) = 0$ as already assumed)
then $f(x) = x$ for every $x \in [0,1]$
(that is, the graph $G$ of $f$ must be
a line segment with slope $1$).
Proof. (Ought to be obvious, but here it is.)
If $x < f(x)$ for some $x$ then $0<x<1$ and
$ | f(x) - f(0) | = f(x) > x = | x - 0 |$ ,
a contradiction.
If, on the other hand,
$x > f(x)$ (or, equivalently $-f(x) > -x$)
for some $x$, then $0<x<1$ and
so $f(1) = 1 > x > f(x)$ and
$| f(1) - f(x) | = f(1) - f(x) = 1 - f(x) > 1 - x = | 1 - x |$ ,
a contradiction.
In a similar way one could prove :
Lemma.
Given $f$ is Lipschitz $1$, if $f(1) = -1$
(and $f(0) = 0$ as already assumed)
then $f(x) = -x$ for every $x \in [0,1]$
(that is, the graph $G$ of $f$ is a line
segment with slope $-1$).
Lemma.
If $f$ is Lipschitz $1$ (and as before
$f(0) = 0$ ) and if $-1 < f(1) < 1$, then
the graph $G$ of $f$ is not hsb.
Proof.
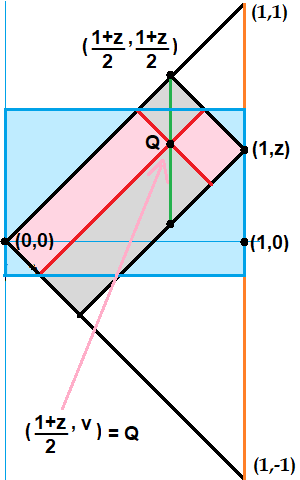
Say $f(1) = z$, so $-1 < z < 1$ and
the point $(1,z)$ is on the graph $G$ of $f$.
Assume for convenience that $0 < z <1$, and
see the enclosed picture.
Take a perpendicular from point $(1,z)$
to the line with slope $1$ going through the origin,
so this is a straight line segment
connecting $(1,z)$ to $( \frac{1+z}2 , \frac{1+z}2)$.
Let $f( \frac{1+z}2 ) = v$, so the point
$Q = ( \frac{1+z}2 , v )$ is on the graph $G$ of $f$. (Note that $Q$ must be on the vertical line segment shown in green on the
picture, with bottom point $( \frac{1+z}2 , \frac{3z-1}2 )$ and top point $( \frac{1+z}2 , \frac{1+z}2 )$, since the point $(1,z)$
is on the graph of $f$ which is Lipschitz $1.$)
Taking lines with slope $1$ and $-1$ through
$Q$ and intersecting with some other lines
with slope $1$ and $-1$ we obtain the pink-shaded
region as shown in the picture.
Since $f$ is Lipschitz $1$ (and since the points
$(0,0), (1,z)$ and $Q$ belong to $G$)
it follows that the graph $G$ of $f$ is contained in this
pink-shaded region.
Let $B$ be the bounding box for this pink-shaded
region. In the picture $B$ is blue-shaded.
The height of $B$ is $\frac{1+z}2$, indeed, the bottom
of $B$ may go below the $x$-axis, but by an amount
which is exactly the same as the amount
by which the top of $B$ goes below the point
$( \frac{1+z}2 , \frac{1+z}2 )$.
The width of $B$ is clearly $1$.
The bounding box $X$ for $G$ is not shown but it
ought to be contained in $B$ (and hence has height
at most $\frac{1+z}2$) and the width of $X$ is $1$
since $G$ contains the points $(0,0)$ and $(1,z)$.
But then $X$ is not a square, since $\frac{1+z}2< 1$,
which completes the proof.
Here is also a sketch of a proof relating my question to Kevin Johnson's question.
Claim.
If $C$ is a plane continuum whose bounding box $B$ is not
a square then $C$ does cross some (dyadic) rational square.
Proof.
Let $B=[a,b]\times[c,d]$ with, say, $b-a>d-c\ge0.$
There are (dyadic) rationals $p,q,r,s$ with $a<p<q<b$ and
$r<c\le d<s$, and $q-p=s-r.$
In particular $S=[p,q]\times[r,s]$ is a (dyadic) rational square.
There is a subcontinuum $L$ of $C$ such that $L$ is contained in the vertical strip
$V=\{(x,y):p\le x\le q\}$ and $L$ intersects both vertical lines $x=p$ and $x=q.$
(Some variation of the boundary-bumping theorem is used here.)
We have $L\subseteq V\cap B = [p,q]\times[c,d]\subset [p,q]\times[r,s]=S$.
It follows that $C$ crosses $S$ (as $L$ is a connected subset of $C\cap S$ and
$L$ intersects both vertical sides of $S$).
To prove the existence of $L$ one might go as follows. For each positive integer
$n$ let $U_n$ be the $\frac1n$-neighborhood of $C$ and let $K_n$ be a path
in $U_n$ from some point of $C$ on the right edge of $B$ to some point of $C$
on the left edge of $B$. Each such path has a piece $P_n$ that goes from
a point on the vertical line $x=q$ to a point on the vertical line $x=p$ and remains
in the strip between the two lines. These pieces $P_n$ have a subsequence
that converges (in the hyperspace topology) to some continuum $L$ which works.
(Well, again a big theorem was used, but I see no way around this.)
(Whether my question is research-level may be debatable, but I post on MO since it is related to a question already posted on MO.)
Edit (May 7, 2023).
I posted a related question (a link provided further down), including definitions of slenderness and lank rank of a plane continuum, and I think the present question could be stated in a better/more general way using these definitions.
Definition.
Let $B=[a,b]\times[c,d]$ be a rectangle in the plane,
with $w=b-a$ and $h=d-c$ being its width and height.
Define slenderness of $B$ as $\sigma(B)=\frac{h}w$.
Clearly $0<\sigma(B)<\infty$ whenever $w$ and $h$ are positive,
and if $S$ is a square (so $w=h$) then $\sigma(S)=1$.
But we would like to also consider "rectangles" with
either zero width (a vertical line segment) or zero
height (a horizontal line segment) and in this case
$0\le\sigma(B)\le\infty$. To avoid working with $\infty$
we adopt a different but closely related definition,
with values in the interval $[-1,1].$ We call
$\lambda(B)=\frac{-w^2+h^2}{w^2+h^2}$ the lankiness,
or lank rank of $B.$
A vertical line segment
(when $w=0<h$) has lankiness $1$ (it is $100\%$ lanky),
while a horizontal line segment (when $w>0=h$) has
lankiness $-1$, and a square has lankiness $0$.
(The lankiness of a singleton remains undefined,
unless we want to allow the entire interval $[-1,1]$ as its value).
If $C$ is a closed, bounded subset of the plane
then we also define its slenderness $\sigma(C)=\sigma(B)$
and its lankiness $\lambda(C)=\lambda(B)$ where $B$ is the
bounding box for $C.$
For example if $J$ is a line segment of
slope $1$ then $\sigma(J)=1$ and $\lambda(J)=0$, and the same is true for every non-degenerate subcontinuum of $J$.
If $D$ is a line segment of
slope $\frac12$ then $\sigma(D)=\frac12$ and $\lambda(D)=\frac{-3}5$.
With these definitions one could ask a question which is very closely related to the one asked at the top, but without the need to specify slope $-1$ or $1$ in the question.
Question.
Let $C$ be any plane continuum that is not a straight line segment (and not a singleton). Must $C$ contain two subcontinua
$K_1$ and $K_2$ such that
$\lambda(K_1)\not=\lambda(K_2)$
(equivalently $\sigma(K_1)\not=\sigma(K_2)$)?
If the answer to this question was positive then there could not exist any hereditarily square-boxed plane continuum $C$ that is different from a line segment with slope $1$ or $-1$. (This would answer our question stated at the top.) Indeed, if $K_1$ and $K_2$ were two subcontinua of $C$ with $\lambda(K_1)\not=\lambda(K_2)$ then at least one of these two values would be different from $0$, say $\lambda(K_1)\not=0$, so $K_1$ would not be squre-boxed (and hence, also, $K_1$ would cross some (dyadic) rational square).
Here is a related question with the definitions of $\sigma(C)$ and $\lambda(C)$, discussing similar topics.
URL (version: 2023-05-07):
Find at least one square-boxed subcontinuum
Edit May 29-31, 2023.
Result.
Suppose that $C$ is a path-connected
plane continuum that is hsb.
Then $C$ must be a line segment with
slope $1$ or $-1$.
Sketch of proof.
Take any two different points $D$ and $E$ in $C$. It is well known that path-connected is equivalent to arc-connected (for plane continua), that is there is an arc $\gamma:[0,1]\to C$ with $\gamma(0)=D$ and $\gamma(1)=E$. (An arc has no self-intersections, the map $\gamma$ is a homeomorphism from $[0,1]$ to its image $\gamma([0,1]).$)
Let $B$ be the bounding box for $\gamma([0,1])$. Let $T$ and $Y$ be the top and bottom (closed) edges of $B.$ The arc $\gamma$ must intersect all edges of $B$, and in particular (by reversing direction if necessary) there are $\tau<\nu$ such that $\gamma(\tau)\in T$, $\gamma(\nu)\in Y$, and $\gamma((\tau,\nu))\cap(T\cup Y)=\emptyset.$ Then the bounding box for $\gamma([\tau,\nu])$ must be the same size (same height, a square), and in fact must coincide with the bounding box for the entire $\gamma([0,1]).$
We claim that $\gamma(\tau)$ must be one of the two endpoint of $T$ (that is, either the top left or the top right corner of $B$). Indeed, if not then let $V$ and $W$ be the (closed) left and the right vertical edges of $B$, and let $\mu=\min\{t\in(\tau,\nu):\gamma(t)\in(V\cup W)\}$.
The assumption that $\gamma(\tau)$ is not an endpoint of $T$ (so $\gamma(\tau)\not\in V\cup W$) implies that $\tau<\mu$. Then $\gamma([\mu,\nu])$ must have nonempty intersections with each of $V$ and $W$, but (being compact) must remain some positive distance away from the top edge $T$. So the bounding box for $\gamma([\mu,\nu])$ must be as wide as $B$, but not as tall (so it will not be a square), a contradiction that shows that $\gamma(\tau)$ must be an endpoint of $T$, say without loss of generality the left endpoint, call it $P.$ So, $P=\gamma(\tau)$
is the top left corner point of $B.$ Similarly, $\gamma(\nu)$ must be an endpoint of the bottom edge $Y$, and in fact $Q=\gamma(\nu)$ must be the bottom right corner of $B$, diagonally opposite to $P$ (or else we could again find a subarc whose bounding box is as wide but not as tall as $B$). A similar argument shows that as $t$ goes from $\tau$ to $\nu$, the bounding box for $\gamma([\tau,t])$ must have $P$ as its top left corner point, and in addition its bottom right corner point must belong to $\gamma([\tau,t])$. So $\gamma([\tau,\nu])$ must contain every point on the diagonal connecting $P$ to $Q$, and (being an arc) must therefore coincide with this diagonal. Then one could argue that $\tau=0$ for if not, then $\gamma([0,\tau])$ will remain either above or below the diagonal, only intersecting it at $P$; say $\gamma([0,\tau))$ remains above the diagonal, then easily implying that the width of its bounding box is bigger than its height (even if possibly not as wide as $B$). All in all we picked two arbitrary different points $D$ and $E$, and an arbitrary arc $\gamma$ in $C$ connecting them, and showed that these two points must be the diagonally opposite corners $D=P=\gamma(0)$ and $E=Q=\gamma(1)$ of the bounding box $B$ of $\gamma$, and $\gamma$ must be the diagonal
connecting these two points. Say $\ell$ is the line through $D$ and $E$, then it follows that $C\subset\ell.$ Indeed $\ell$ has slope $-1$ (without loss of generality) but if $F$ was a point in $C\setminus\ell$ then we could connect $F$ with one arc in $C$ to $D$ and with another arc in $C$ to $E$, and these two arcs would each have to be the diagonal of its respective bounding box, and will have to have slope $1$ (since $-1$ is already taken by $\ell\ $), and this could not happen since $D$ and $E$ are different points.
This completes the proof (or sketch) that if $C$ is a path-connected hsb continuum in the plane then $C$ must be a line segment with slope $1$ or $-1.$
Extra comments.
I had a failed proof that an hsb continuum $C$ must be a line segment with slope $1$ or $-1$, even without the assumption that $C$ is path-connected. As a step in the proof I incorrectly thought that I had proved that for every subcontinuum $K$ of $C$, at least one of the corner points of the bounding box $B$ of $K$ must belong to $K$. The idea was that, if not, then use the boundary bumping theorem, we extend $K$ to a slightly bigger continuum, and since this bigger continuum must stay away from the corner points of $B$, then it must go through "somewhere in the middle" of one of the edges. I was thinking "the first moment of time" when this bigger continuum "leaves" $B$, it must be through one of the edges, and then the result is that the bounding box of this slightly bigger continuum cannot be a square (since we elongated or extended the original bounding box at only one of the edges). The problem with this argument is that there need not be a "first moment of time"... this concept may apply when we have a path (or an arc) but not for a general continuum. So, this slightly bigger continuum may pop out of $B$ "simultaneously" at or through two, three, or all four edges (to the best of my understanding). I feel I may be overlooking something, and perhaps this argument could be fixed (need to spend more time with this). At any rate, IF one could prove that for every subcontinuum $K$ of $C$, at least one of the corner points of the bounding box $B$ of $K$ must belong to $K$ (or at least to $C$), THEN one could also prove that $C$ must be a line segment of slope $1$ or $-1$. (The main idea: Gradually grow $K$ in size, get a chain of increasing by set inclusion subcontinua of $C$, then without loss of generality for a dense set in some time interval it will be, say, the top left corner point of the respective bounding box that belongs to the respective subcontinuum. Take the closure of these top left corner points, we obtain an arc, a subcontinuum of $C$, so this arc must be a line segment of slope $1$ or $-1$, and well there were a few more details from there but perhaps we get a line segment of slope $1$ to intersect another one of slope $-1$ and the union of these two segments is not square-boxed ... don't remember all details, but I believe they could be recovered one way or another.)
So this motivates one more question (which for now I don't feel asking separately):
Question.
Suppose that $C$ is a hsb plane continuum, that $K$ is any subcontinuum, and $B$ is the bounding box of $K$. Is it necessarily true that at least one of the corner points of $B$ must belong to $K$? (Is it necessarily true that at least one of the corner points of $B$ must belong to $C$?)

 $G$, boxed" />
$G$, boxed" />